Distribution of sample means from a normal population

ejss_model_RandomProbabilityFunction  by +Boon Leong Ng and Francisco Esquembre (Paco) is an artifact of learning by a Math teacher who attended the EJS-OSP Singapore workshop.

http://weelookang.blogspot.sg/2014/11/distribution-of-sample-means-from.html https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_model_RandomProbabilityFunction/RandomProbabilityFunction_Simulation.xhtml source: https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_src_RandomProbabilityFunction.zip author: boonleong and paco

Introduction

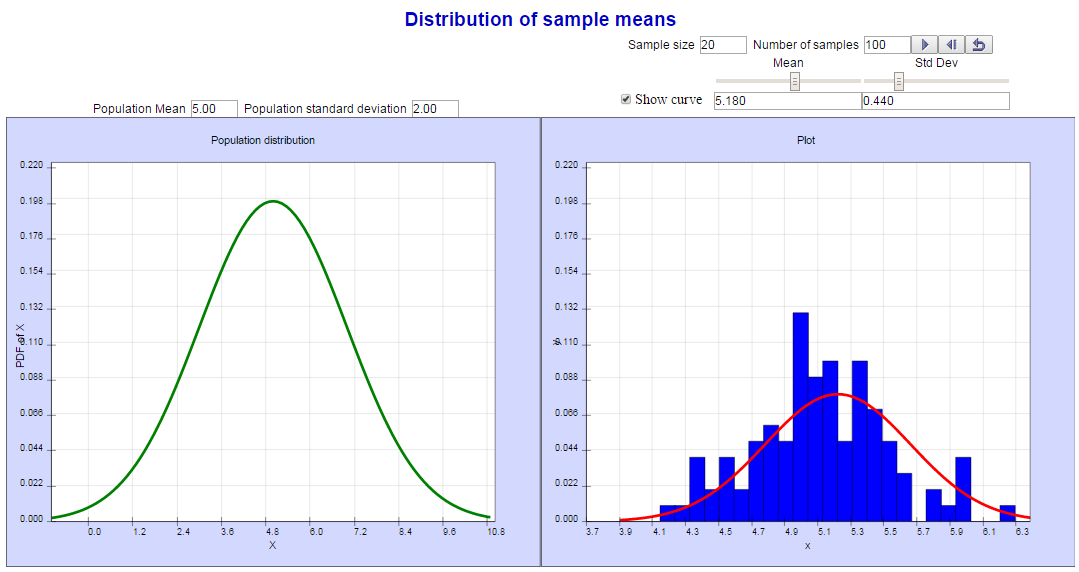
The sampling distribution of a statistic is the distribution of that statistic, considered as a random variable, when derived from a random sample of size n. It may be considered as the distribution of the statistic for all possible samples from the same population of a given size. The sampling distribution depends on the underlying distribution of the population, the statistic being considered, the sampling procedure employed, and the sample size used. There is often considerable interest in whether the sampling distribution can be approximated by an asymptotic distribution, which corresponds to the limiting case as n → ∞.


For example, consider a normal population with mean μ and variance σ². Assume we repeatedly take samples of a given size from this population and calculate the arithmetic mean  x̄ for each sample — this statistic is called the sample mean. Each sample has its own average value, and the distribution of these averages is called the "sampling distribution of the sample mean". This distribution is normal

 (n is the sample size) since the underlying population is normal, although sampling distributions may also often be close to normal even when the population distribution is not (see central limit theorem). An alternative to the sample mean is the sample median. When calculated from the same population, it has a different sampling distribution to that of the mean and is generally not normal (but it may be close for large sample sizes).