Energy of a Satellite in circular orbit in terms of distance from Earth, r

A satellite in orbit possesses kinetic energy, KE, (by virtue of its speed motion) and

gravitational potential energy, PE, (by virtue of its position within the Earth’s gravitational field).

Hence, total energy of a satellite,

TE = PE + KE
 

   $T$ E = ( m ) ( - G M r ) + 1 2 m v 2    --- Equation (1)

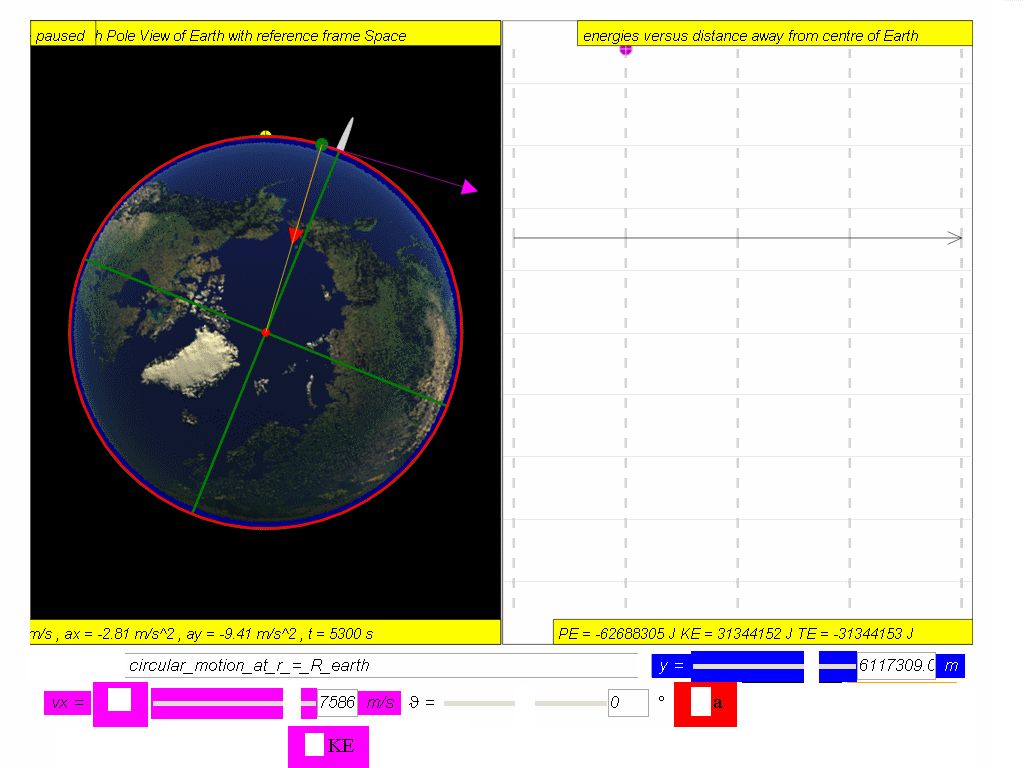
Recall that for a satellite in circular orbit, its gravitational force $$ F = G M 1 M 2 r 2 acts as the centripetal force:
    ΣF =  ma

$\genfrac{}{}{0.1ex}{}{G}{}$ m M r 2 = m v 2 r

simplifying and multiply by 

$\frac{G}{}$ m M 2 r = 1 2 m v 2 = K E--- Equation (2)


Substituting equation (2) into (1),
Hence total energy of a satellite, TE = PE + KE
   

$T$ E = ( m ) ( - G M r ) + G m M 2 r = - G m M 2 r

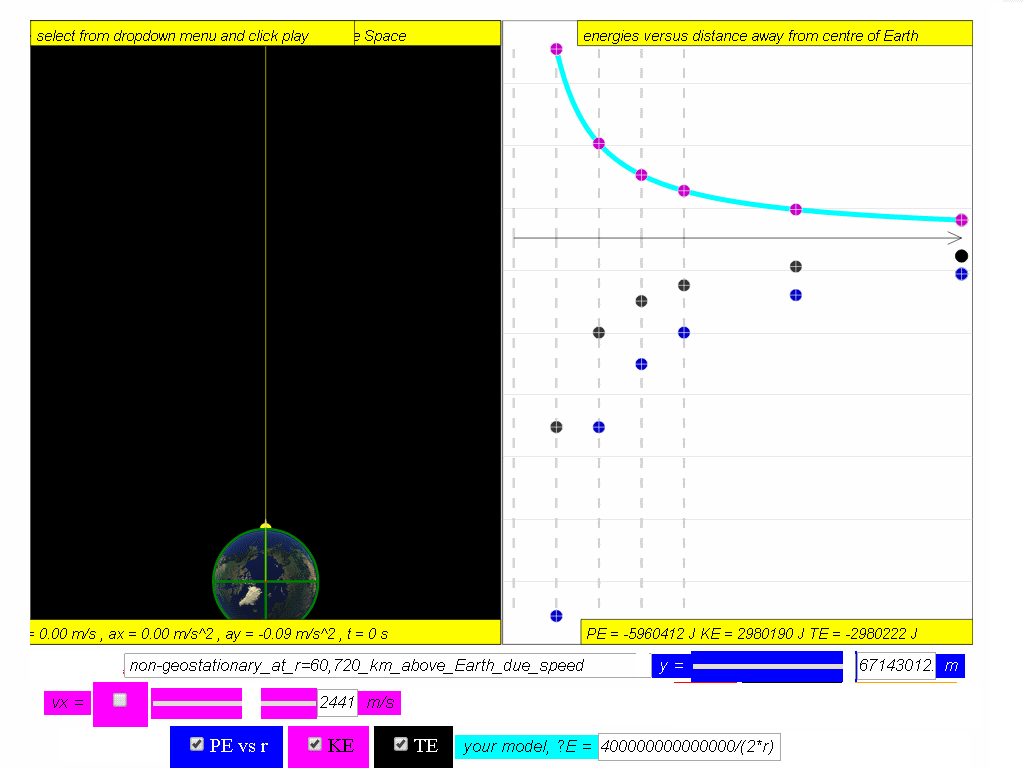
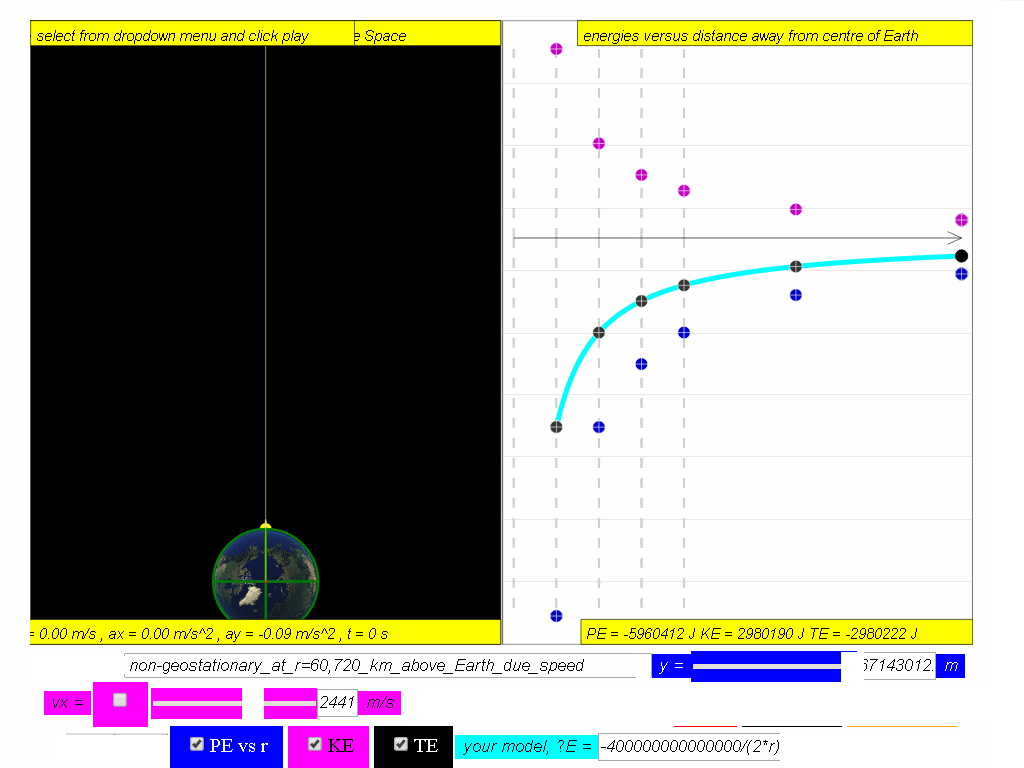
A typical graph showing the relationship between PE, KE and TE with respect to the distance, r, from centre of Earth, O, is as shown.

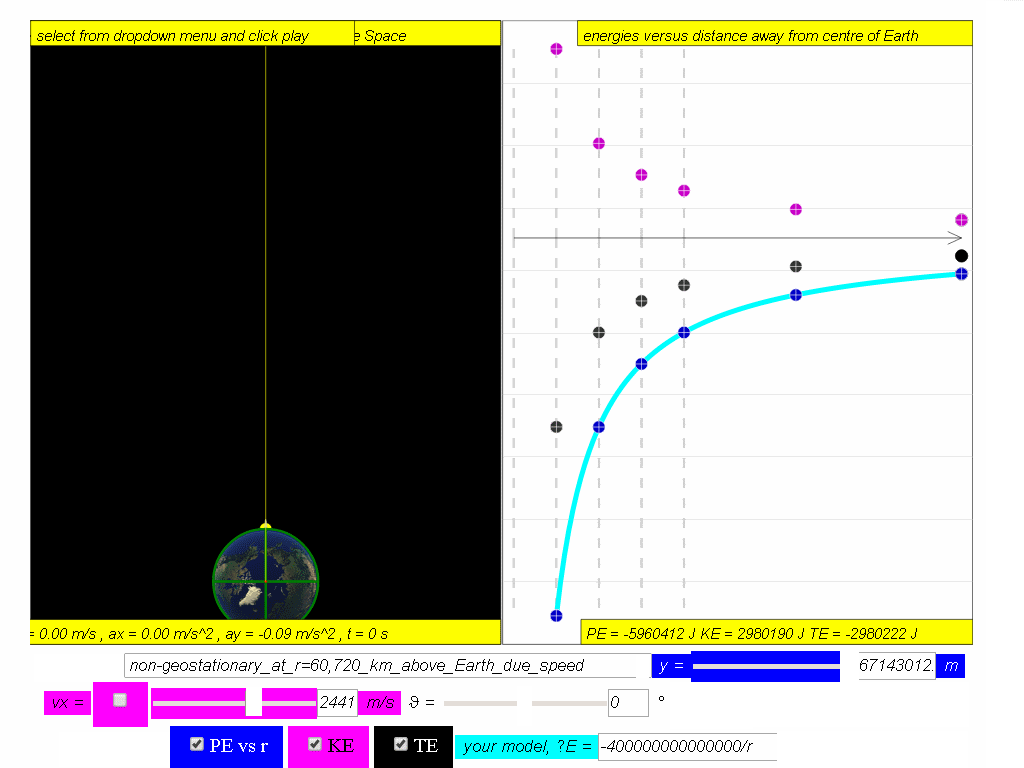
Assuming mass of object m_object = 1kg,  Mass of Earth M_earth = 6.0x10²⁴ kg, G =6.67x10-11 N m2 kg-2 , The potential energy PE = $P$ E = - G m M r = 6.67 x 1 0 -11 ( 1 ) ( 6.0 x 1 0 24 ) r = - 4 x 1 0 14 r

similarly, determine the equation/model that describes KE and TE and key them into the simulation to test your understanding

$K$ E = G m M 2 r = 6.67 x 1 0 -11 ( 1 ) ( 6.0 x 1 0 24 ) 2 r = 4 x 1 0 14 2 * r

$T$ E = - G m M 2 r = - 6.67 x 1 0 -11 ( 1 ) ( 6.0 x 1 0 24 ) 2 r = - 4 x 1 0 14 2 * r

Java Model

http://iwant2study.org/lookangejss/02_newtonianmechanics_7gravity/ejs/ejs_model_NewtonsMountainwee06.jar

Model:

https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_model_gravity08_1/gravity08_1_Simulation.xhtml