Example:
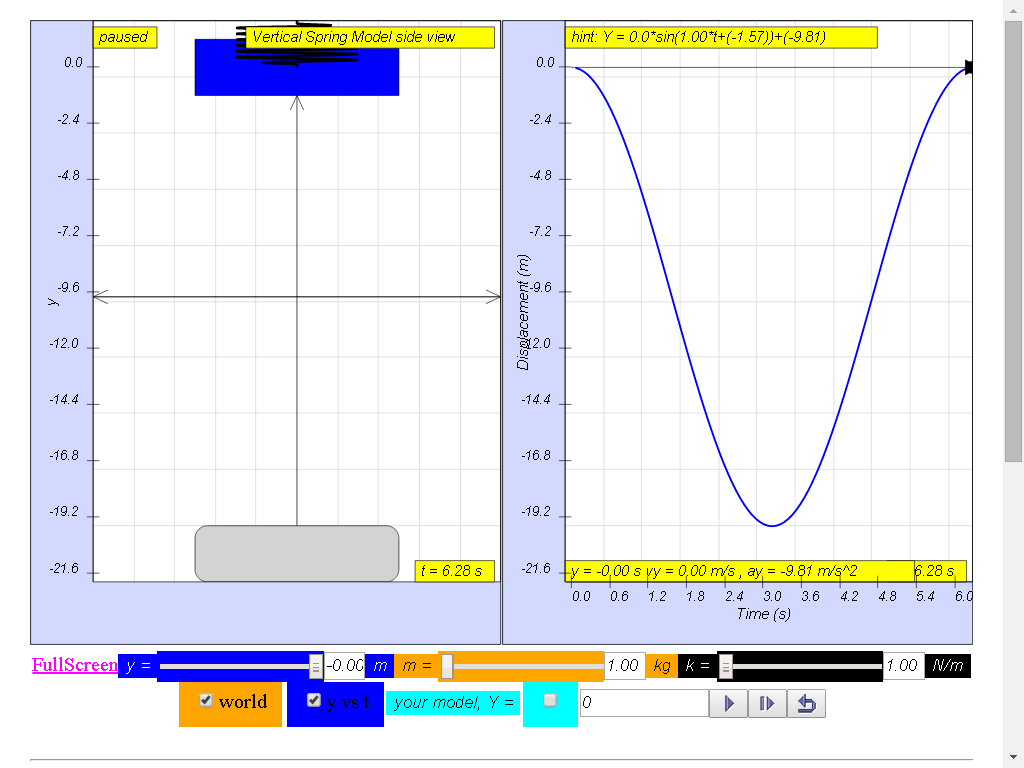
Spring-mass system
A mass suspended from a spring, displaced vertically and released
to move freely to produce up and down motion
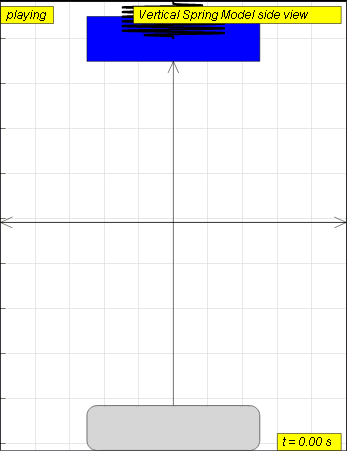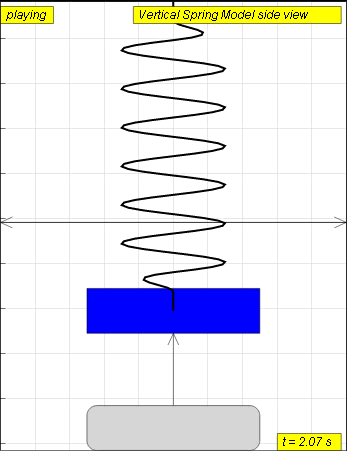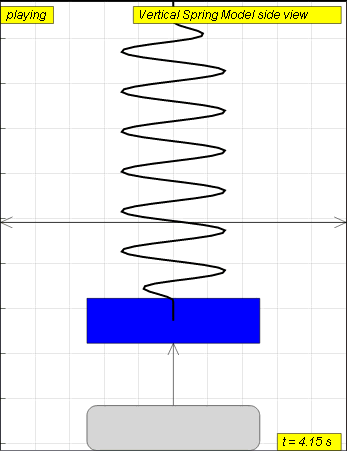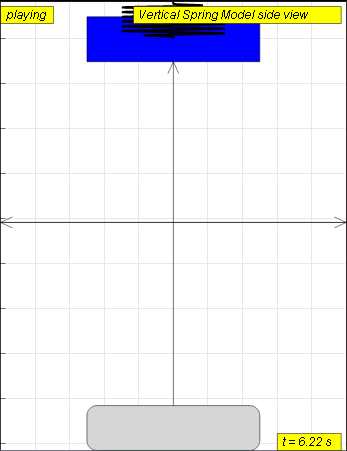
The model view
of a mass suspended from a spring, displaced vertically and
released to move freely to produce up and down motion with right
panel showing the displacement, velocity and acceleration.
Q1:
under what conditions would this vertical spring mass system’s
motion be not well modeled as a simple harmonic motion?
H1: the model assumes the restoring force by the spring is F =
-ky, it is always correct for any magnitude of oscillation y in
real springs. Is it always valid in the real spring motion when
the spring is hyper extending beyond the linear limits of Hooke's
Law ?
Q2:
change the simulation y=0 m and Play the model. In the your model
Y = _________, suggest your own suitable model that can describe
the motion y.
Q3:
in this same motion, propose the velocity and acceleration
model(s)
Q4:
carry out some other conditions, verify that the general equations
for displacments if can be model by Y = Y0sin(ωt+φ)+Yshift where the usual
symbols have the usual meaning. Hence or otherwise, Suggest with
reasons, the meaning of Y0 , ω , φ , and Yshift.
Q5:
similarly, suggest whether the model(s) for velocity, vY =ωY0cos(ωt+φ)
and
aY = - ω2Y0sin(ωt+φ).
Test them out using an example of your choice.
Youtube
http://youtu.be/P-Umre5Np_0 MIT
Physics Demo -- Spray Paint Oscillator. A can of spray paint is
attached to a spring oscillator. A roll of paper is run past the
oscillating can. The result is a sine wave -
http://en.wikipedia.org/wiki/Sine_wave - on the paper.Watch the
original video on MIT TechTV -http://techtv.mit.edu/videos/803-spray-paint-oscillator
Tracker
activity:
http://weelookang.blogspot.sg/2012/08/tracker-modeling-in-spring-mass-system.html
Run
Model:
https://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_model_SHM16_1/SHM161_Simulation.xhtml
backup
http://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_model_SHM02/SHM02_Simulation.xhtml

