Example

A body moves in a simple harmonic motion and the following graph gives the variation of its displacement x with time t.

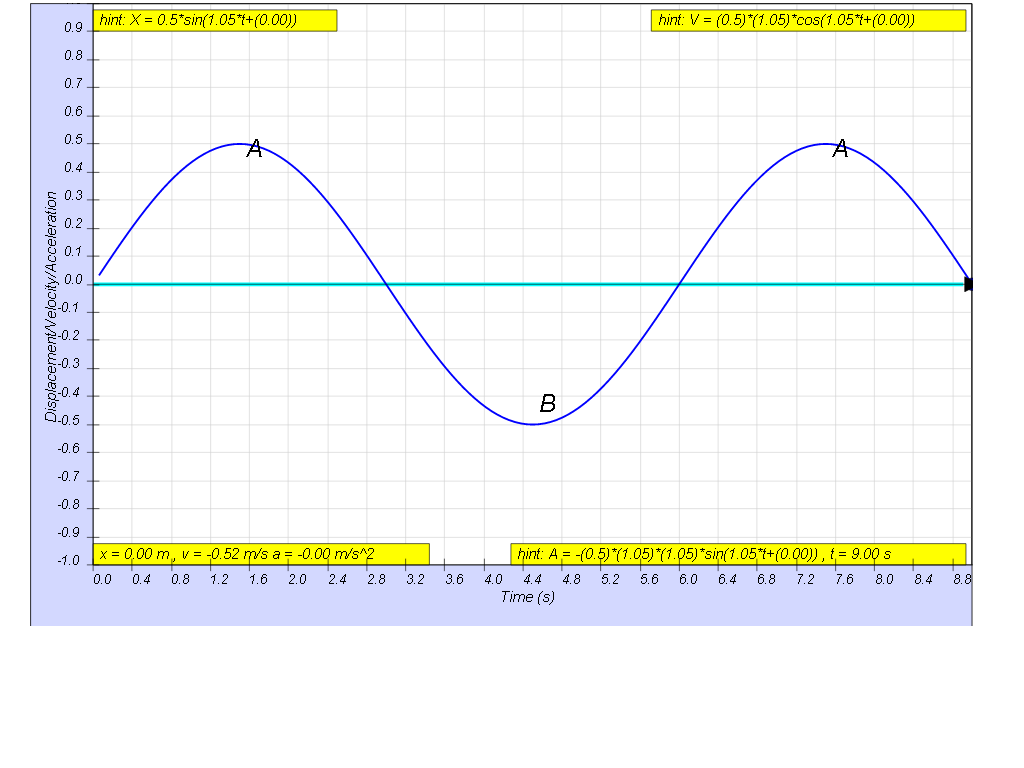
a) Write an equation to represent the given simple harmonic motion.
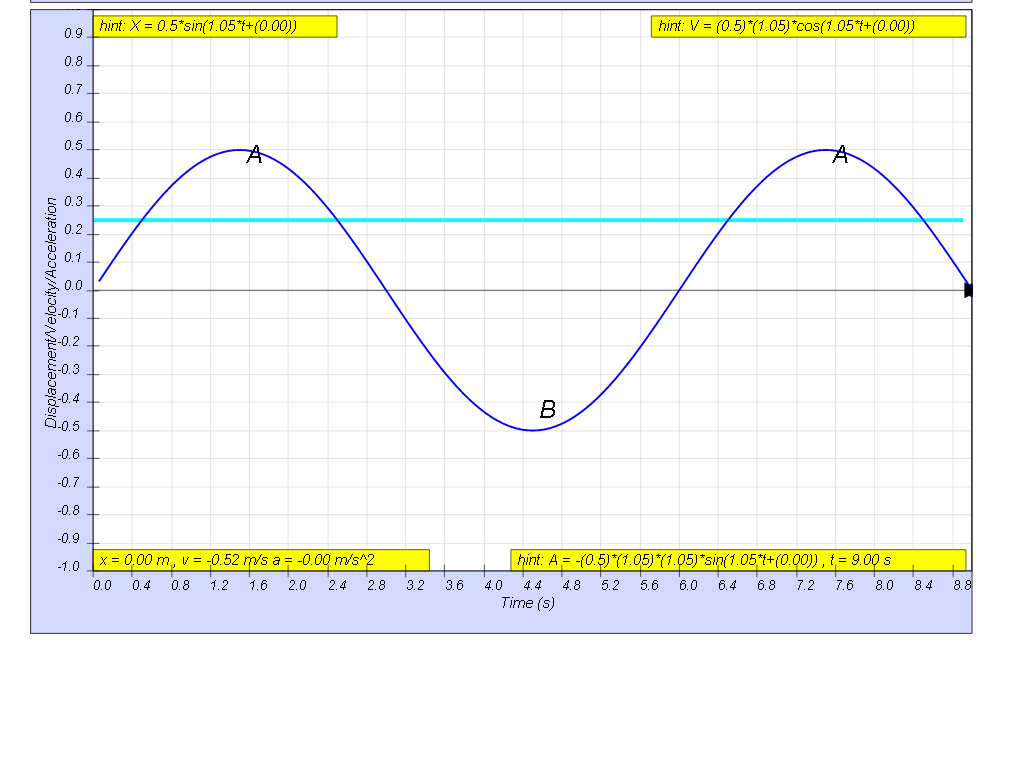
b) Find the time duration in the first cycle when the body is located above a displacement of 0.25 m.

[ x = x_o sin (1.05 t) , Δt = 1.9 s ]

The hint can be found in the model

Solution:

a) x0 = 0.5 m

$since ω$ = 2 π T $, ω$ = 2 π 6.0

ω = 1.05
x = x0sin(ω t) = 0.5 sin(1.05t)

b) At x = 0.25 m,

substituting back into the equation,

0.25 = 0.5 sin(1.05t)

solving for t gives

0.5 = sin(1.05t)

solving of the ranging of time, t

1.05t = 0.524 and π - 0.524

t₁ = 0.5 , t₂ = 2.4 s

looking at the graphical form of the solution of the 2 times, t₁ and t₂.

giving the range to be t₂ - t₁ = 2.4 - 1.5 = 1.9 s

Model:

http://dl.dropboxusercontent.com/u/44365627/lookangEJSworkspace/export/ejss_model_SHM13/SHM13_Simulation.xhtml