About
1.2.7 Example
A body moves in a simple harmonic motion and the following graph gives the variation of its displacement x with time t.
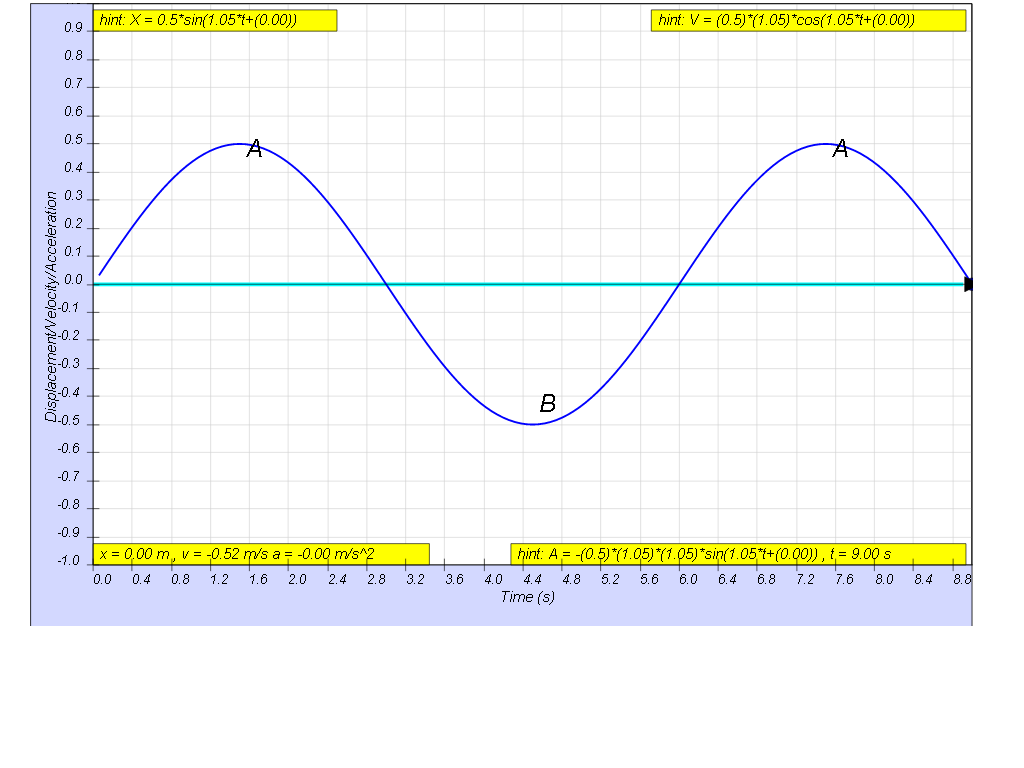
a) Write an equation to represent the given simple harmonic motion.
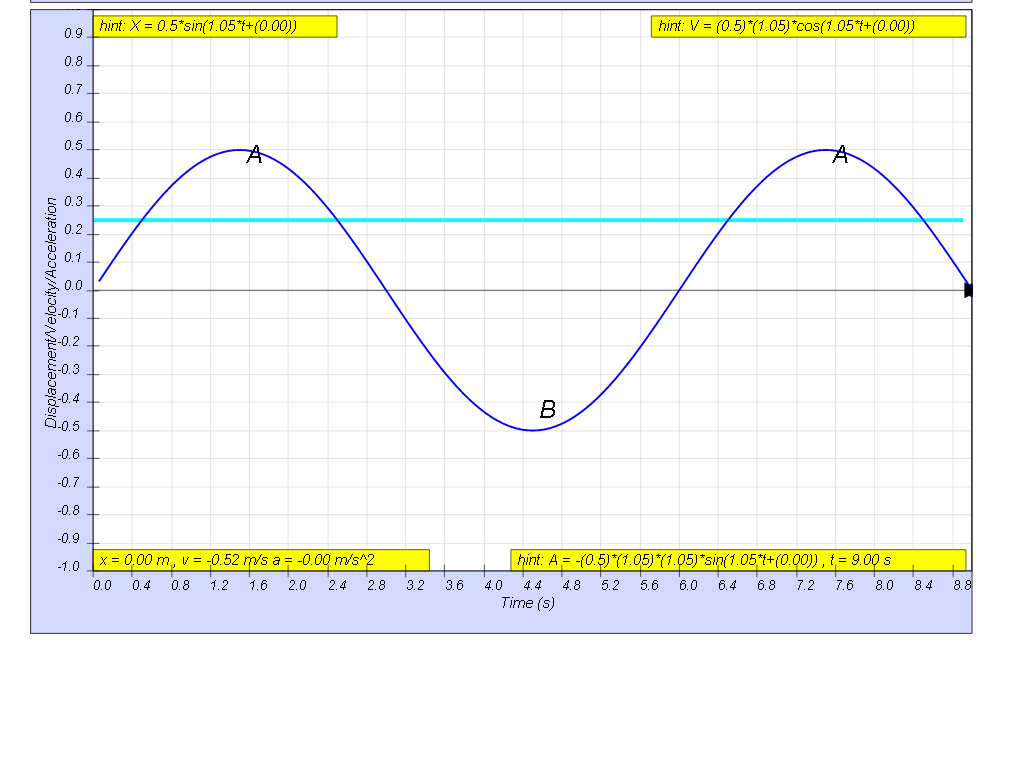
b) Find the time duration in the first cycle when the body is located above a displacement of 0.25 m.
[ x = xo sin (1.05 t) , Δt = 1.9 s ]
The hint can be found in the model

1.2.7.1 Solution:
a) x0 = 0.5 m
ω = 1.05
x = x0sin(ω t) = 0.5 sin(1.05t)
b) At x = 0.25 m,
substituting back into the equation,
0.25 = 0.5 sin(1.05t)
solving for t gives
0.5 = sin(1.05t)
solving of the ranging of time, t
1.05t = 0.524 and π - 0.524
t1 = 0.5 , t2 = 2.4 s
looking at the graphical form of the solution of the 2 times, t1 and t2.
giving the range to be t2 - t1 = 2.4 - 1.5 = 1.9 s
1.2.7.2 Model:
Translations
| Code | Language | Translator | Run | |
|---|---|---|---|---|
 |
||||
Software Requirements
| Android | iOS | Windows | MacOS | |
| with best with | Chrome | Chrome | Chrome | Chrome |
| support full-screen? | Yes. Chrome/Opera No. Firefox/ Samsung Internet | Not yet | Yes | Yes |
| cannot work on | some mobile browser that don't understand JavaScript such as..... | cannot work on Internet Explorer 9 and below |
Credits
 This email address is being protected from spambots. You need JavaScript enabled to view it.
This email address is being protected from spambots. You need JavaScript enabled to view it.
end faq
Testimonials (0)
There are no testimonials available for viewing. Login to deploy the article and be the first to submit your review!
You have to login first to see this stats.