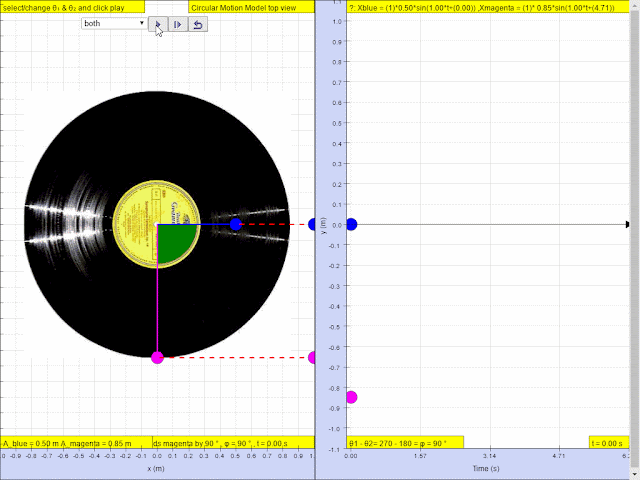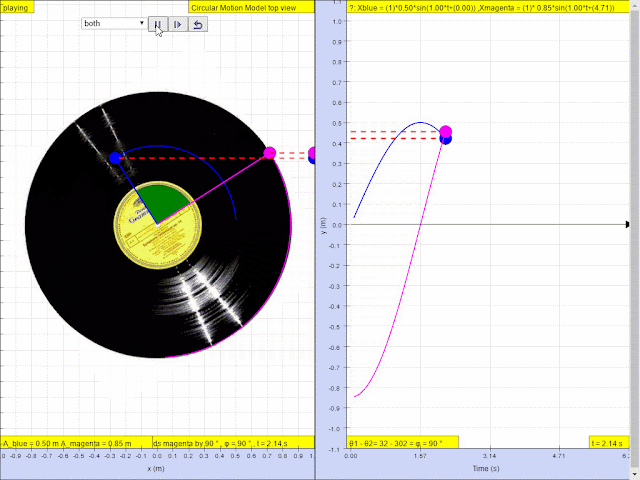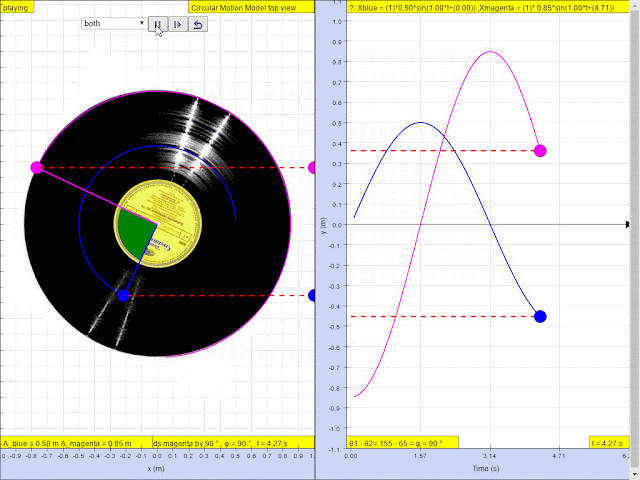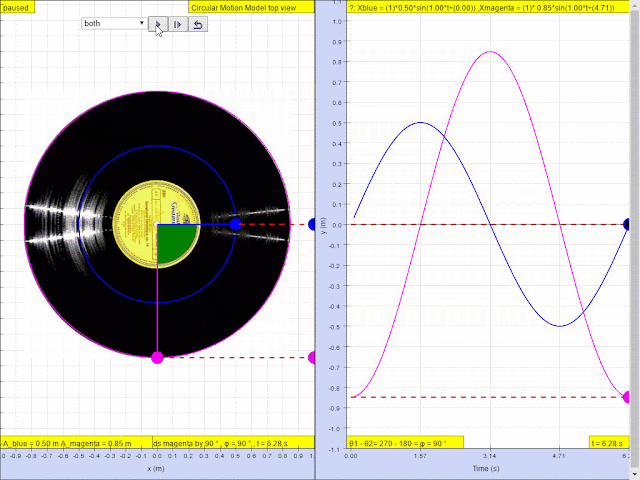
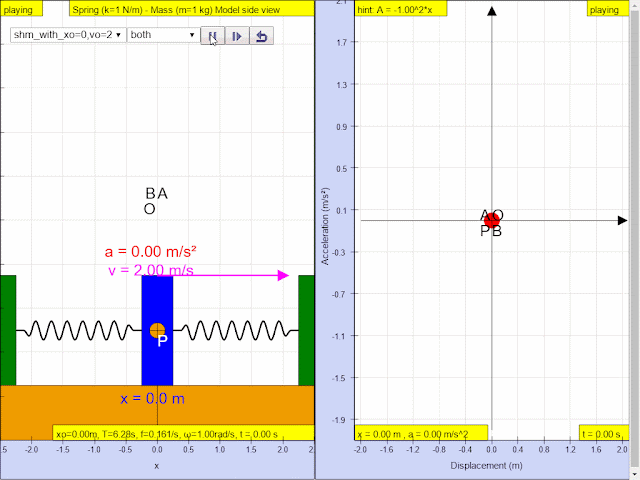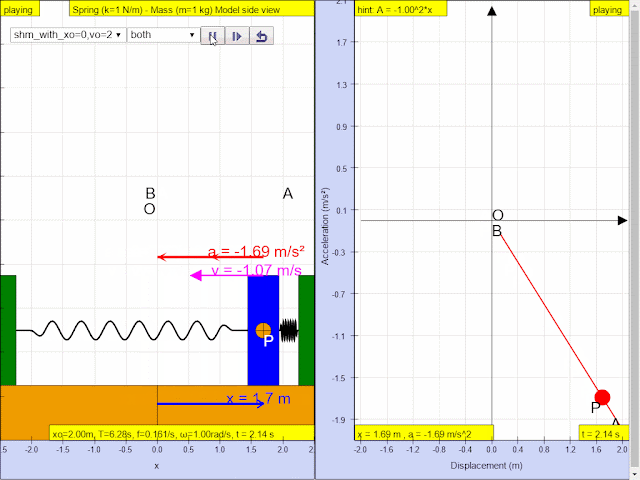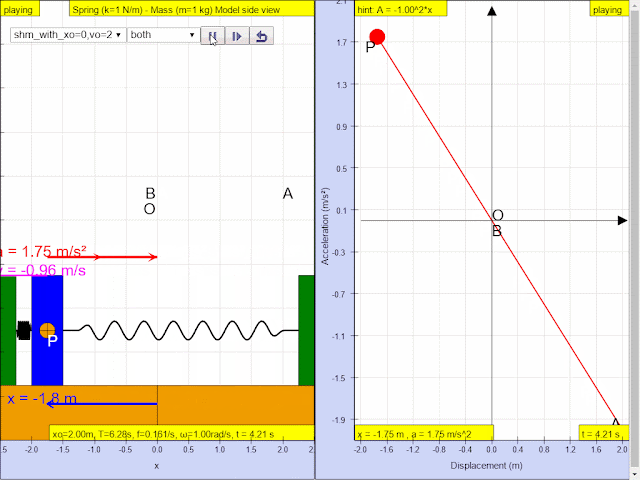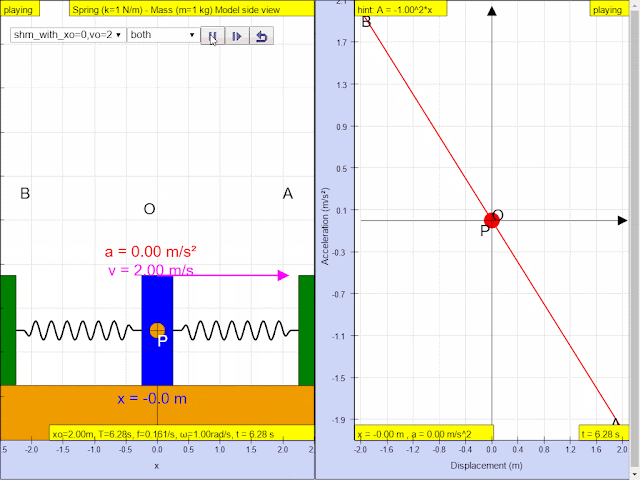
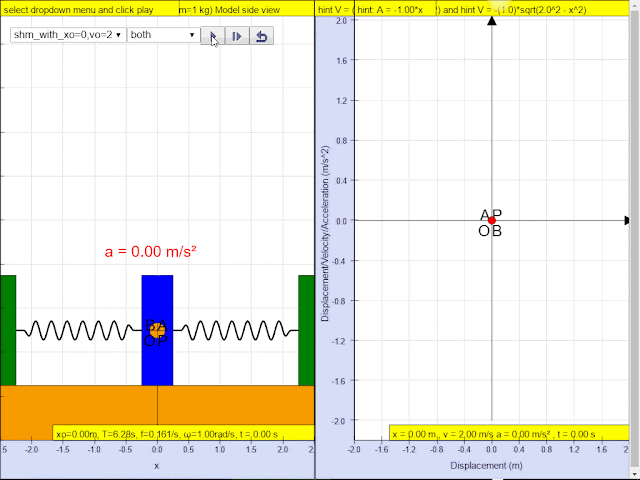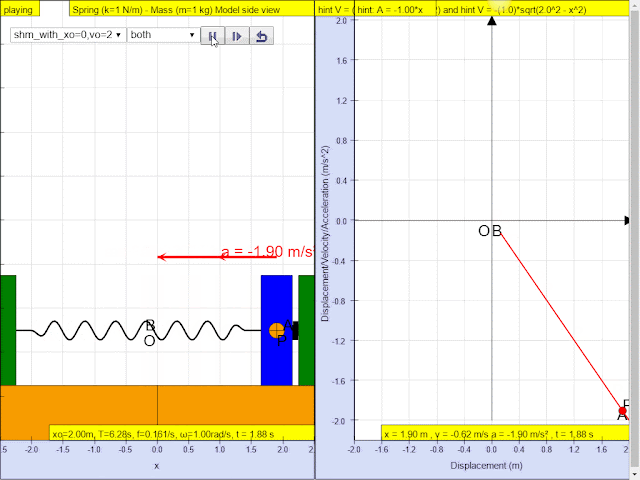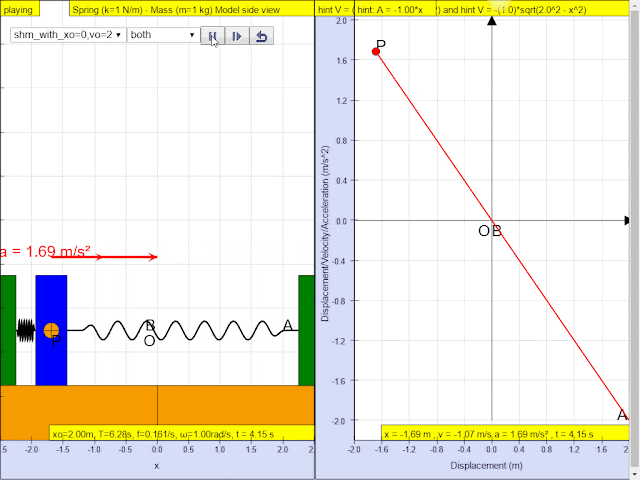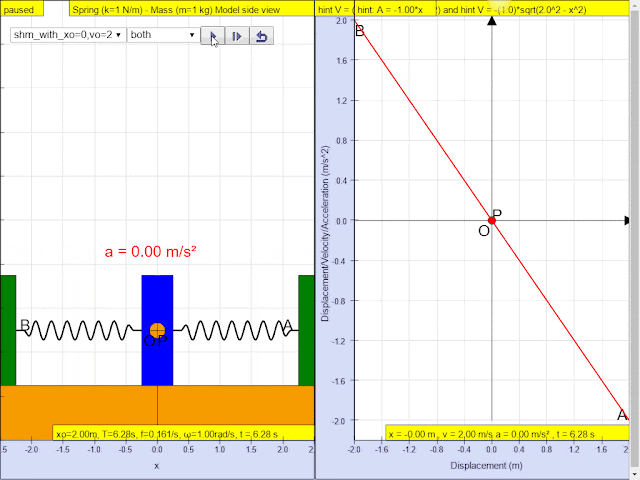
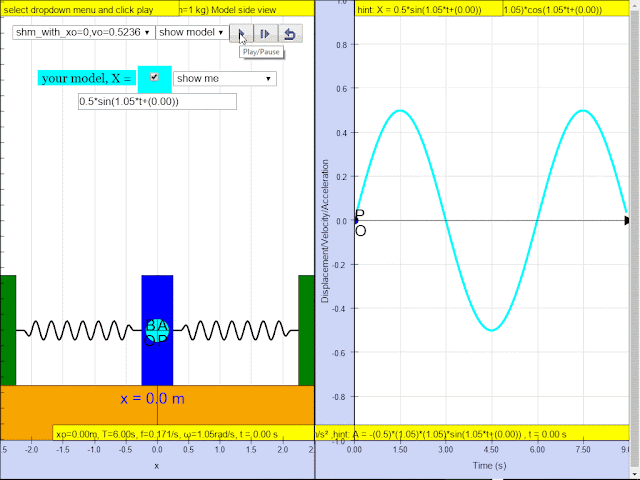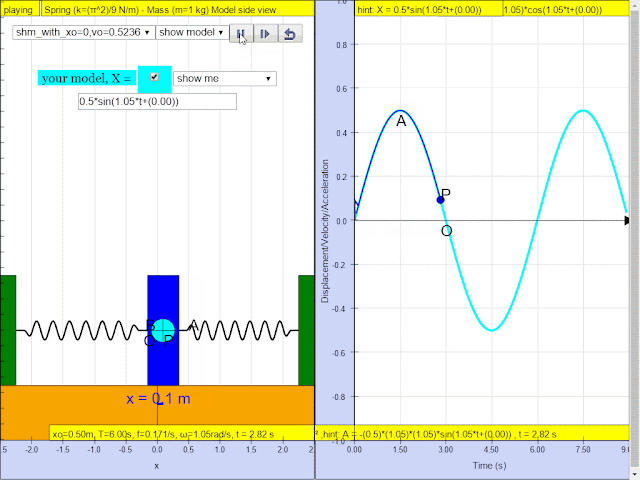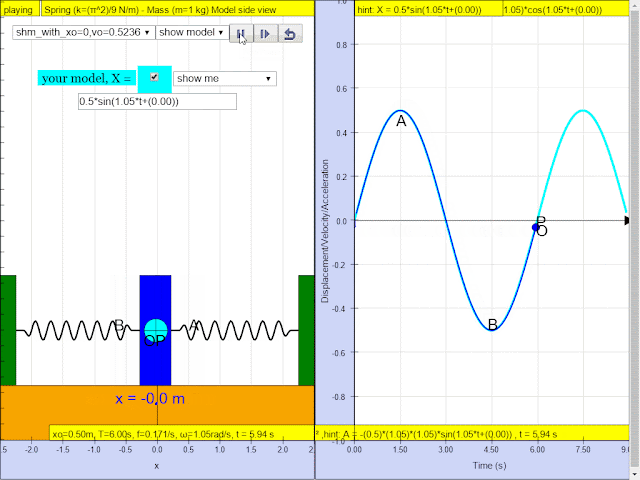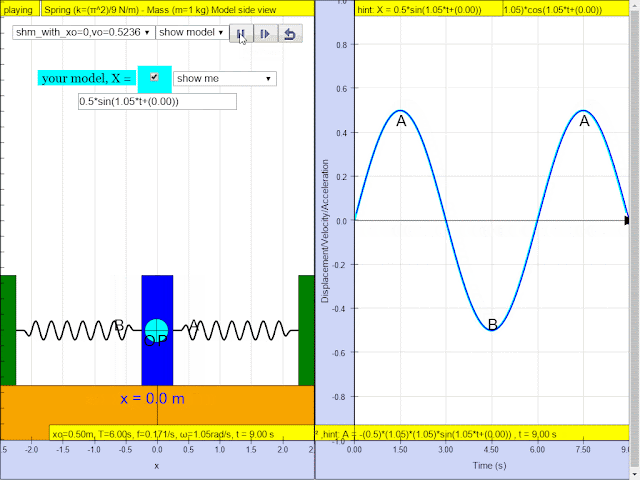
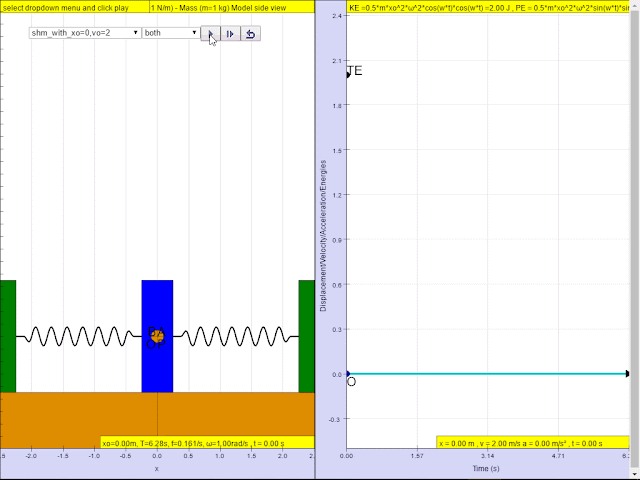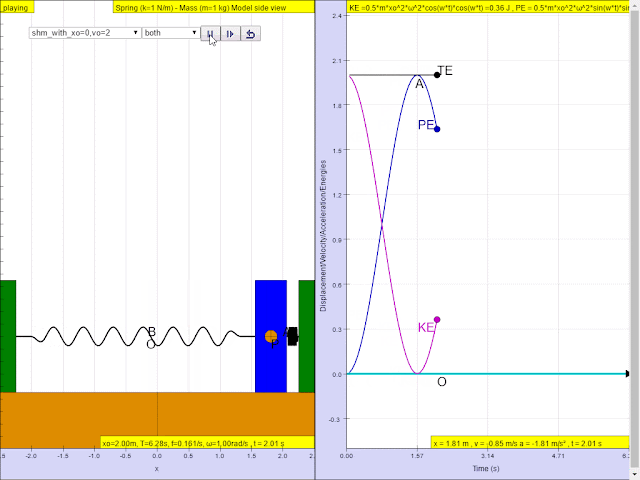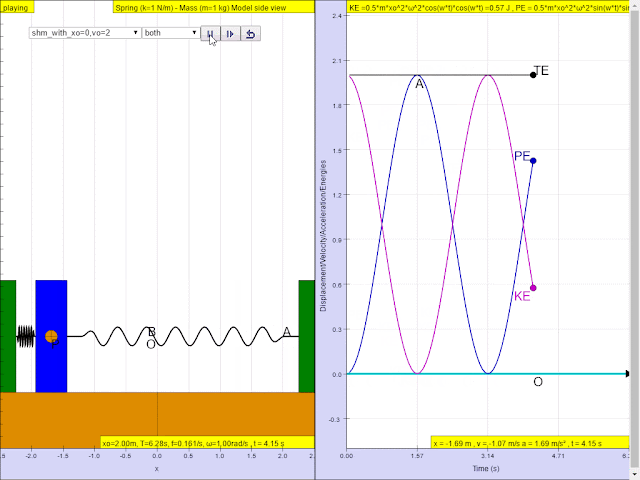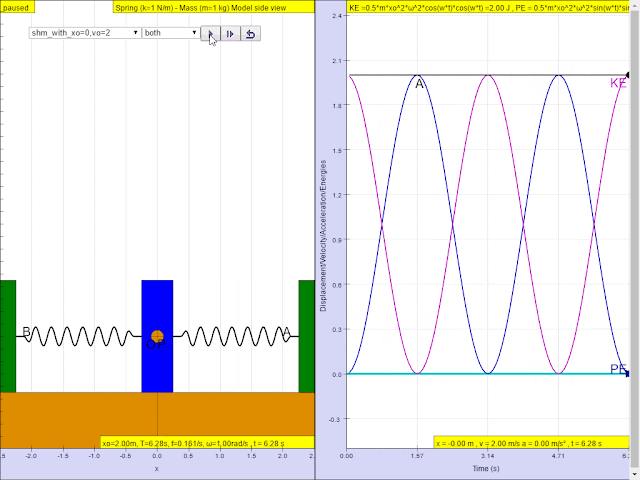
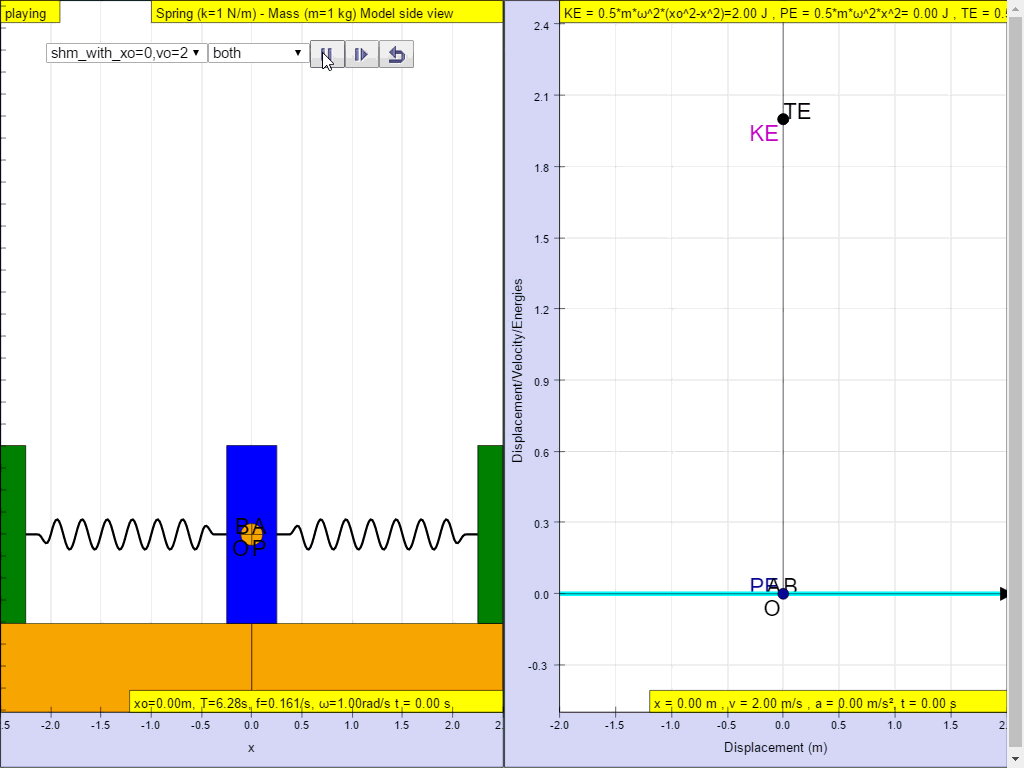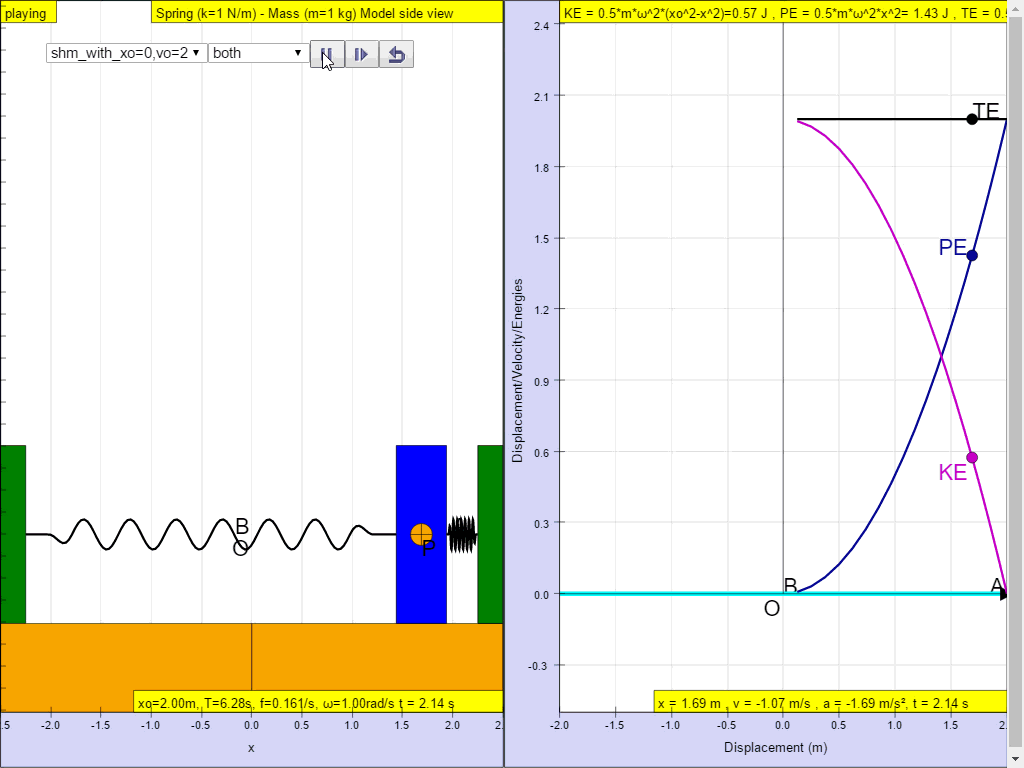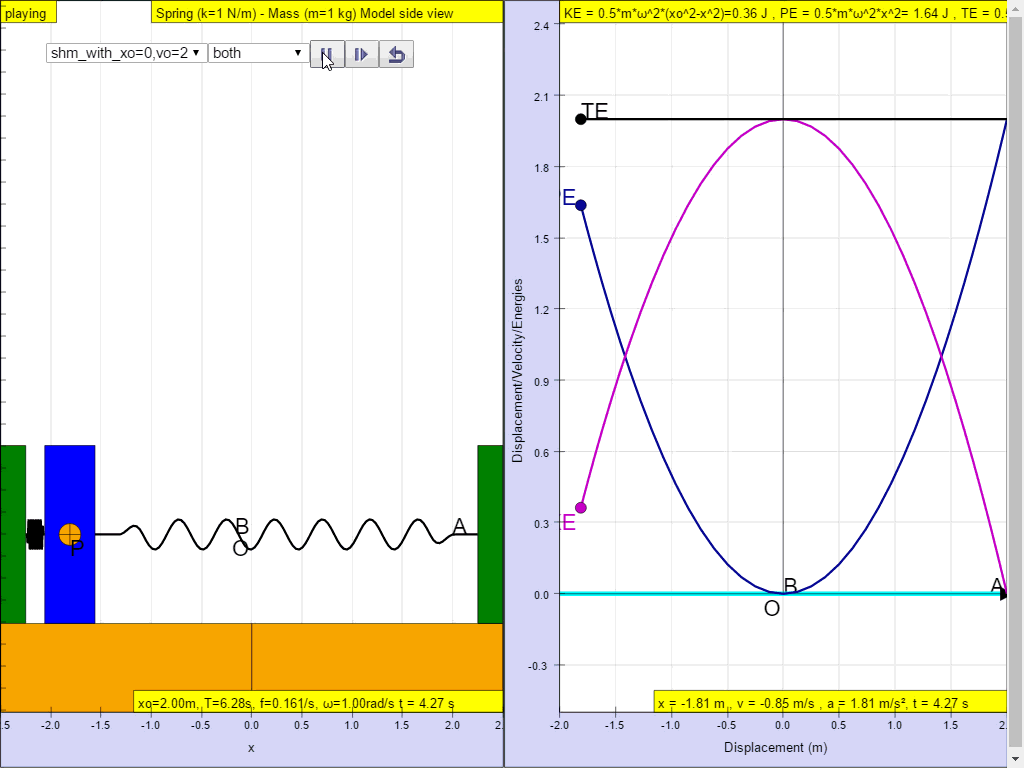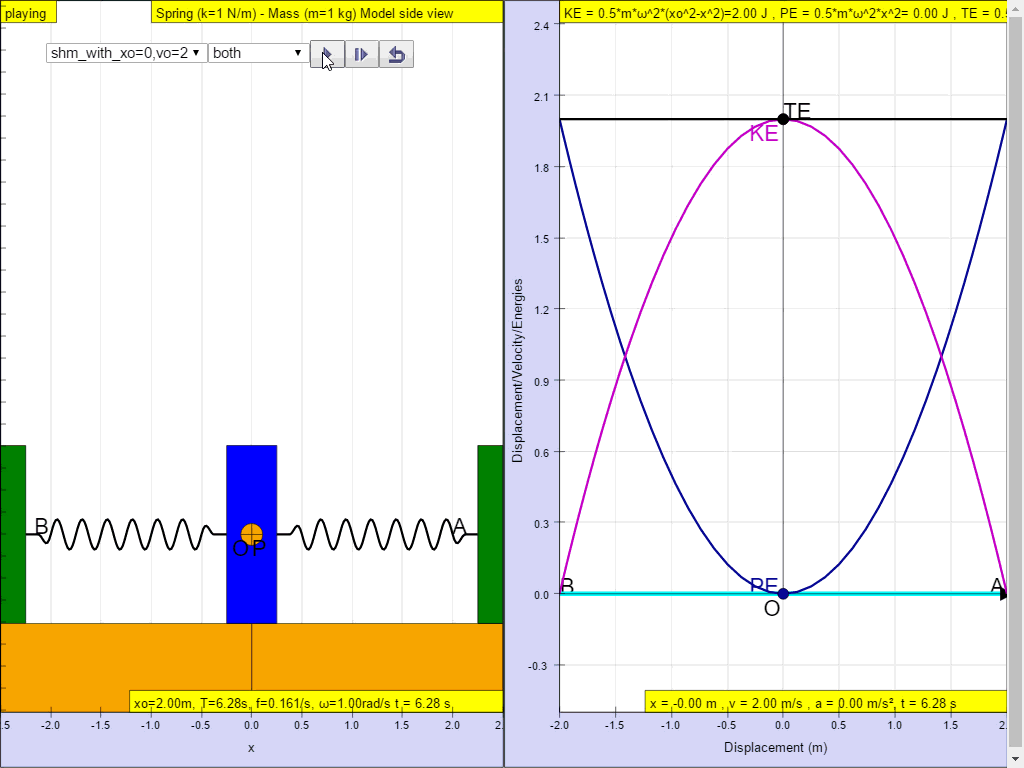
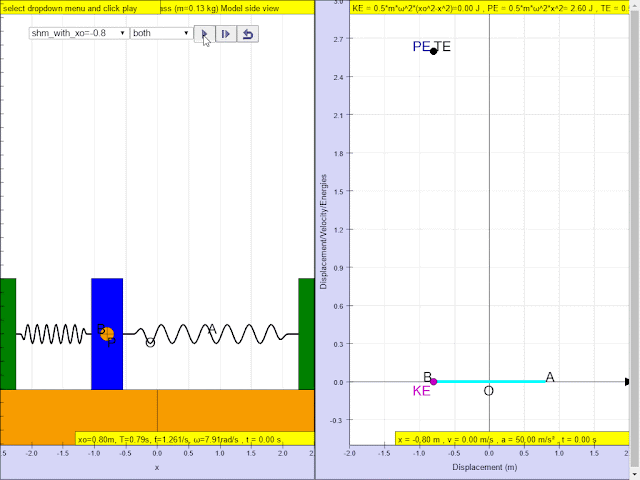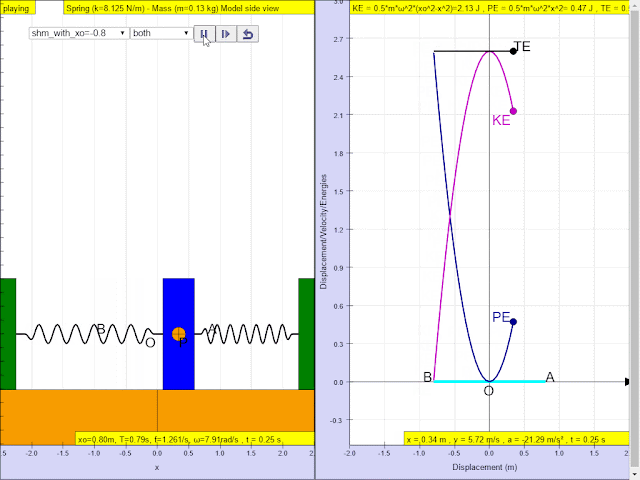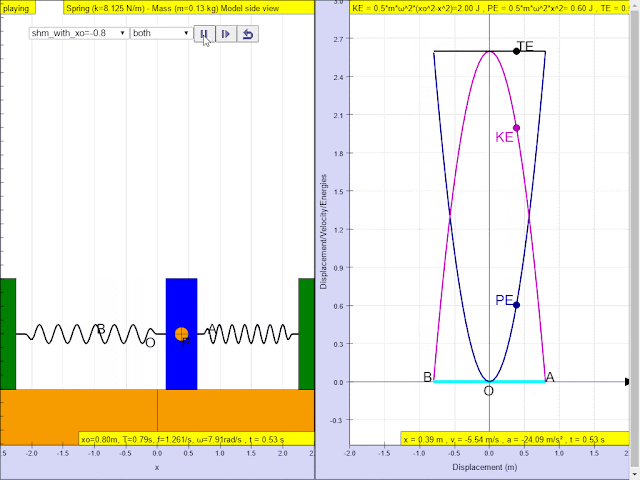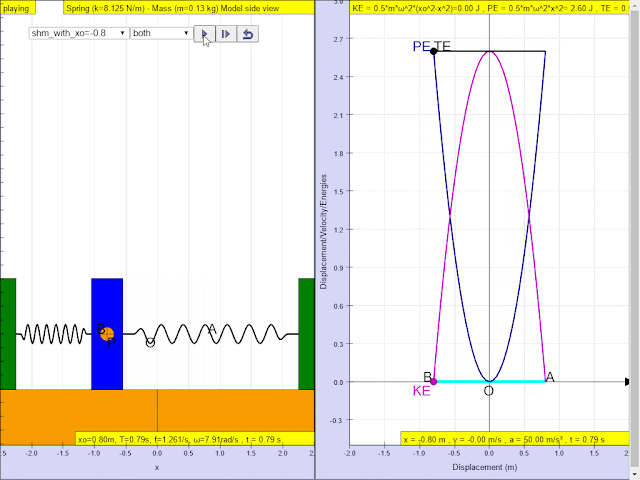
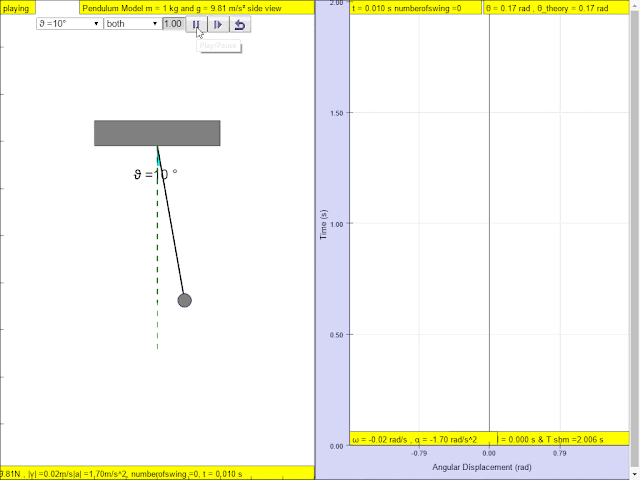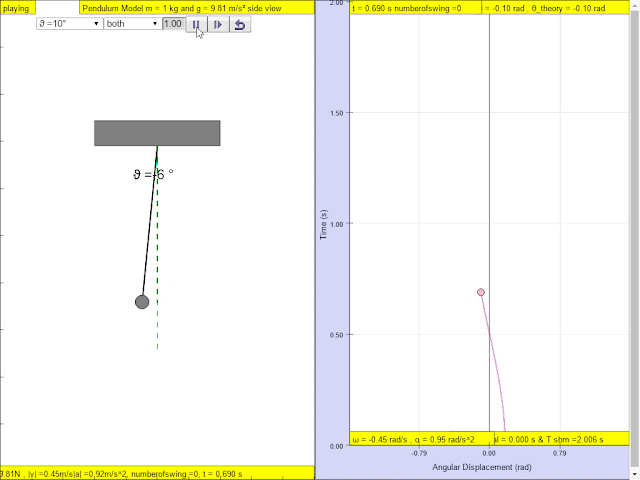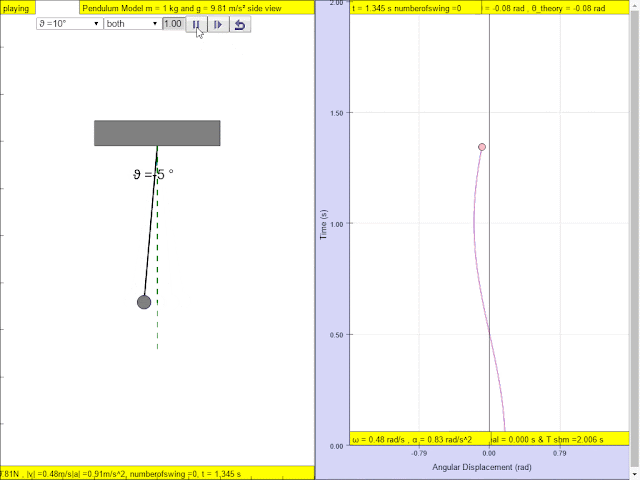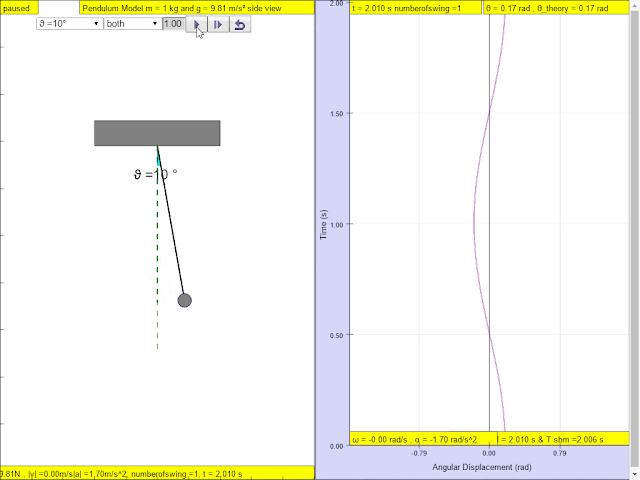
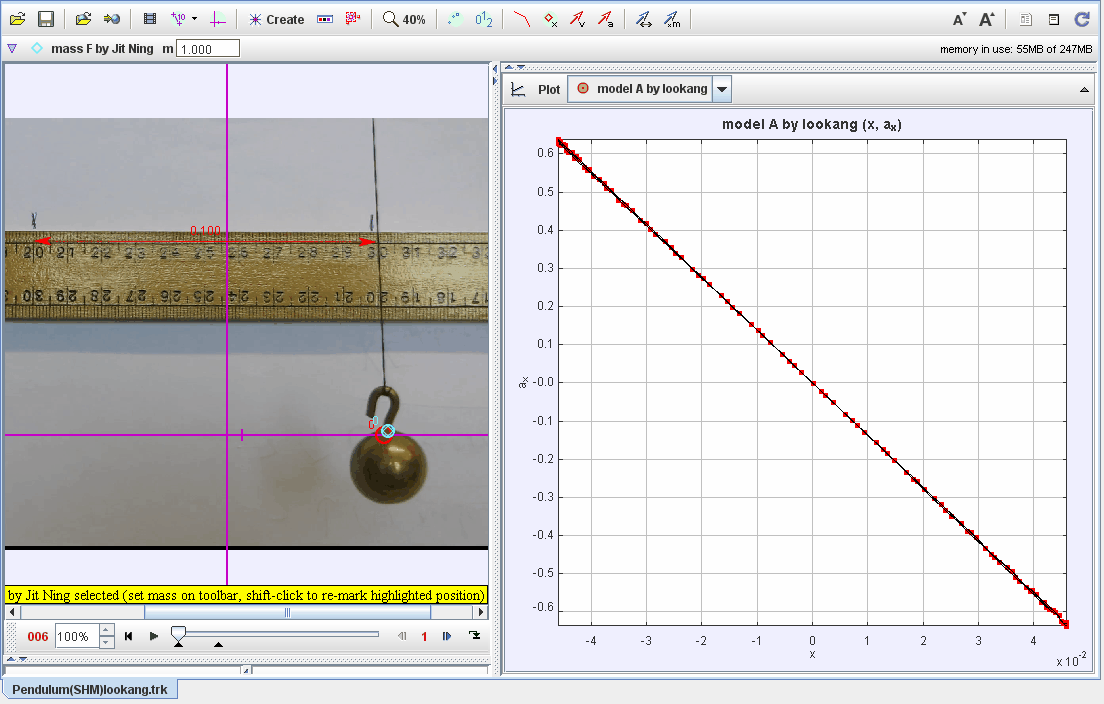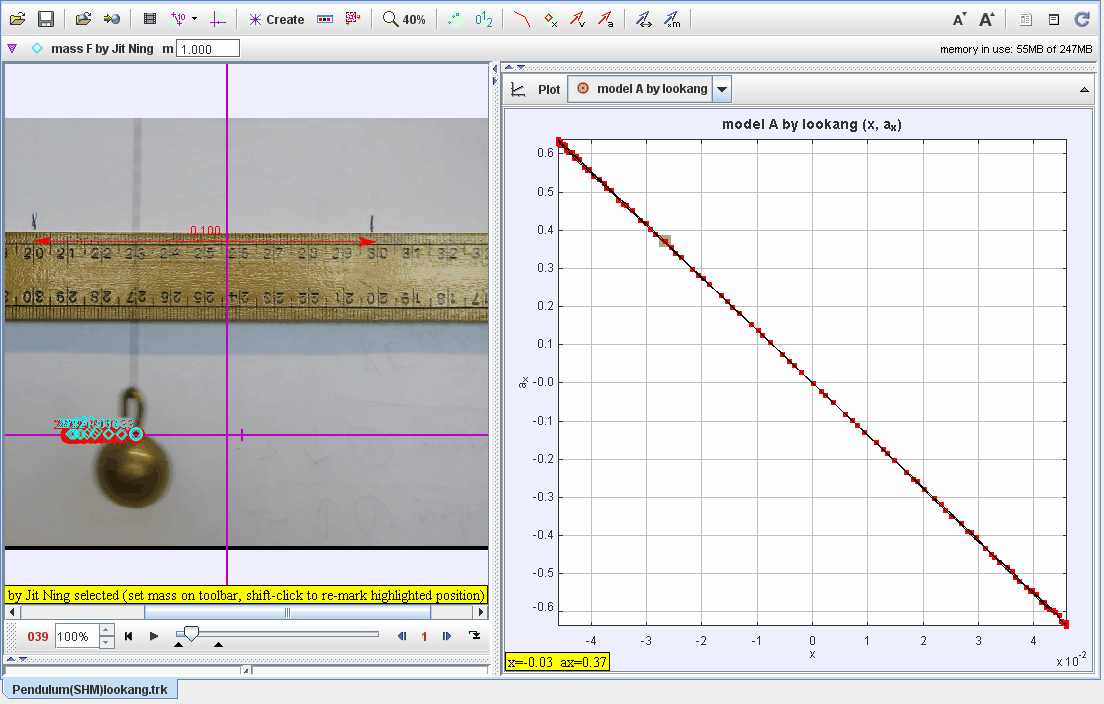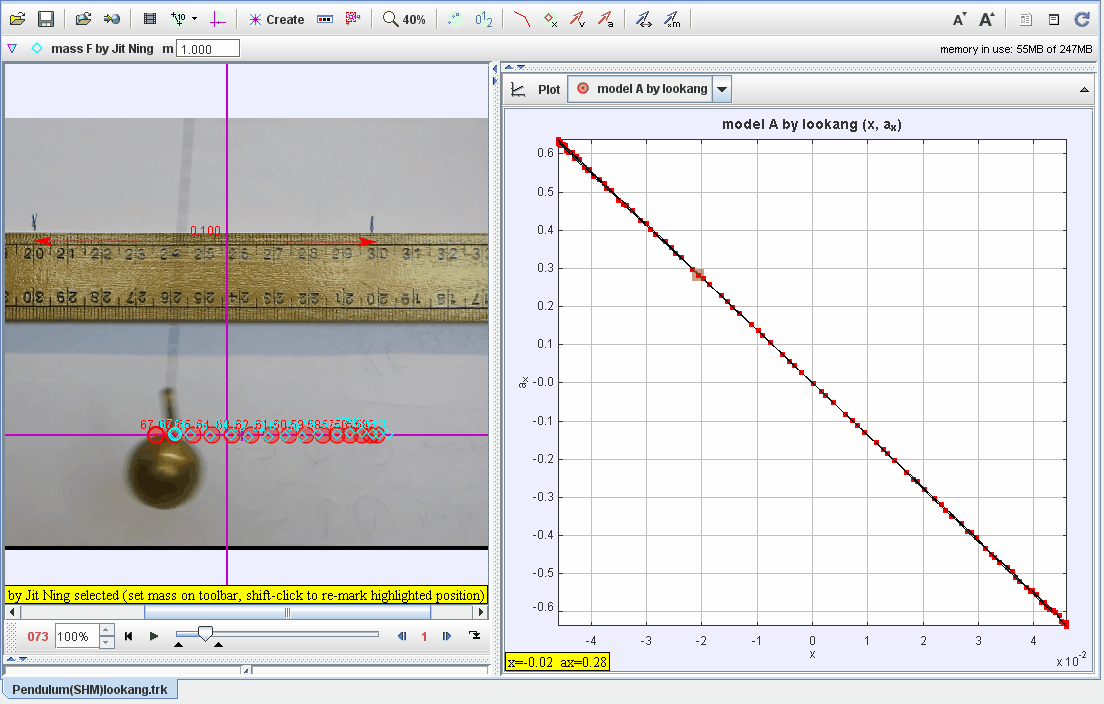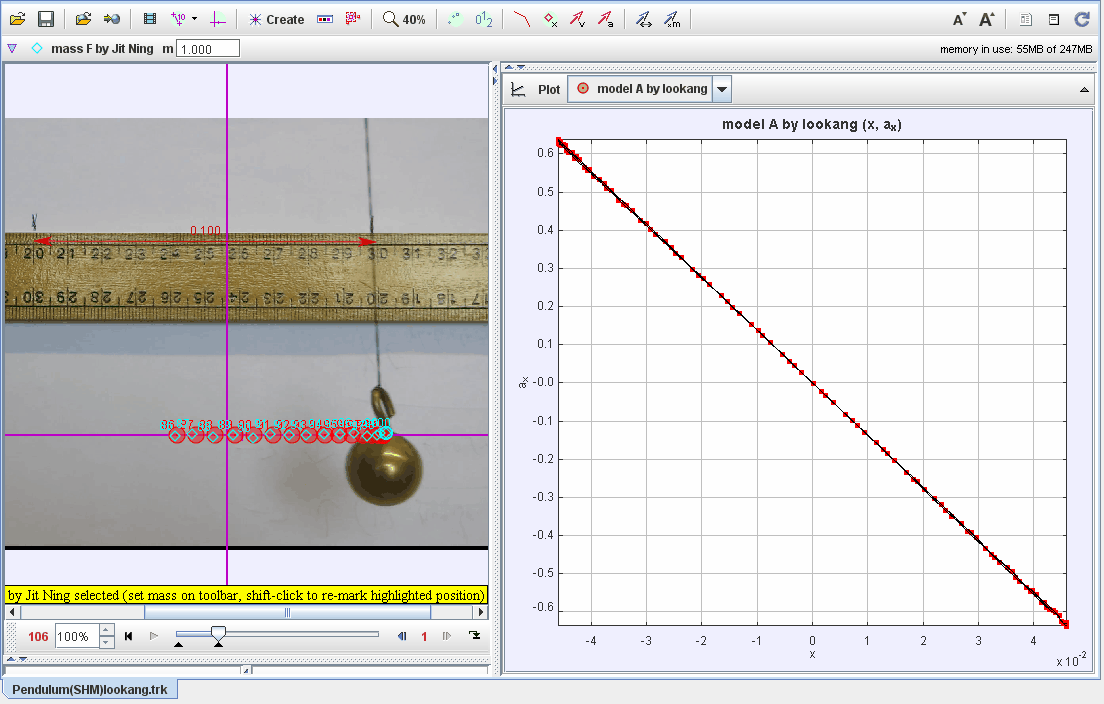
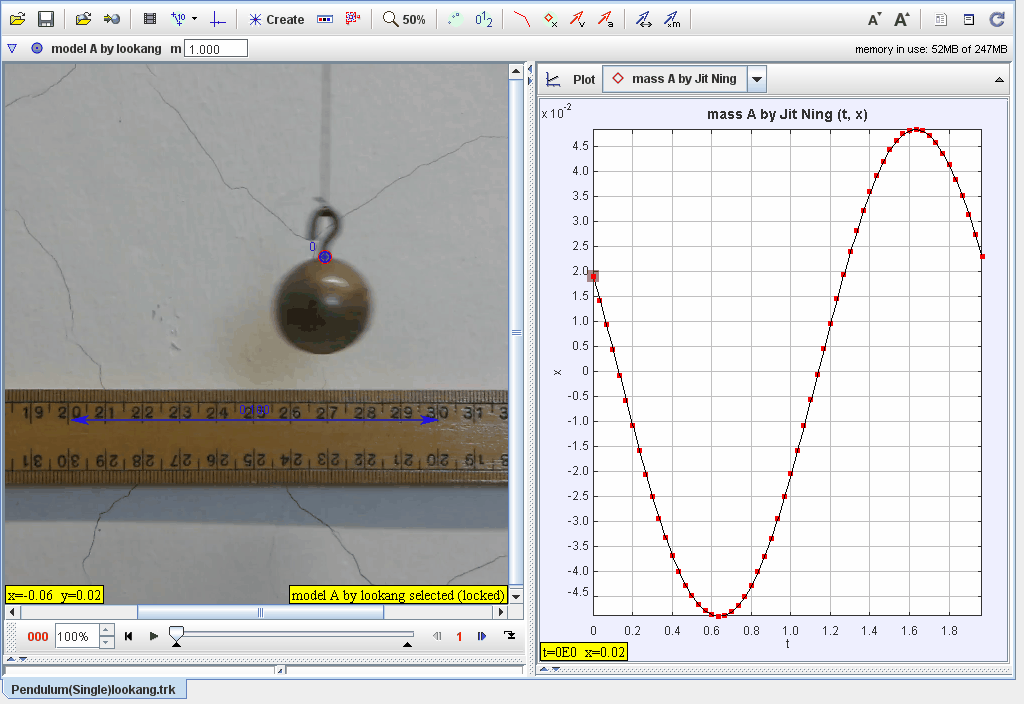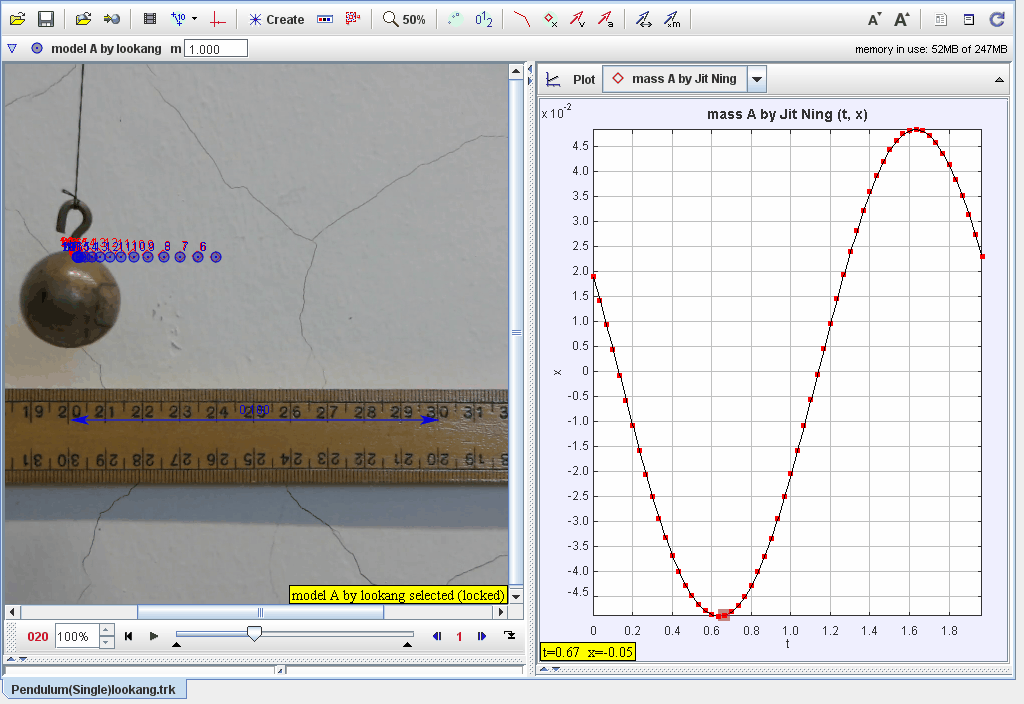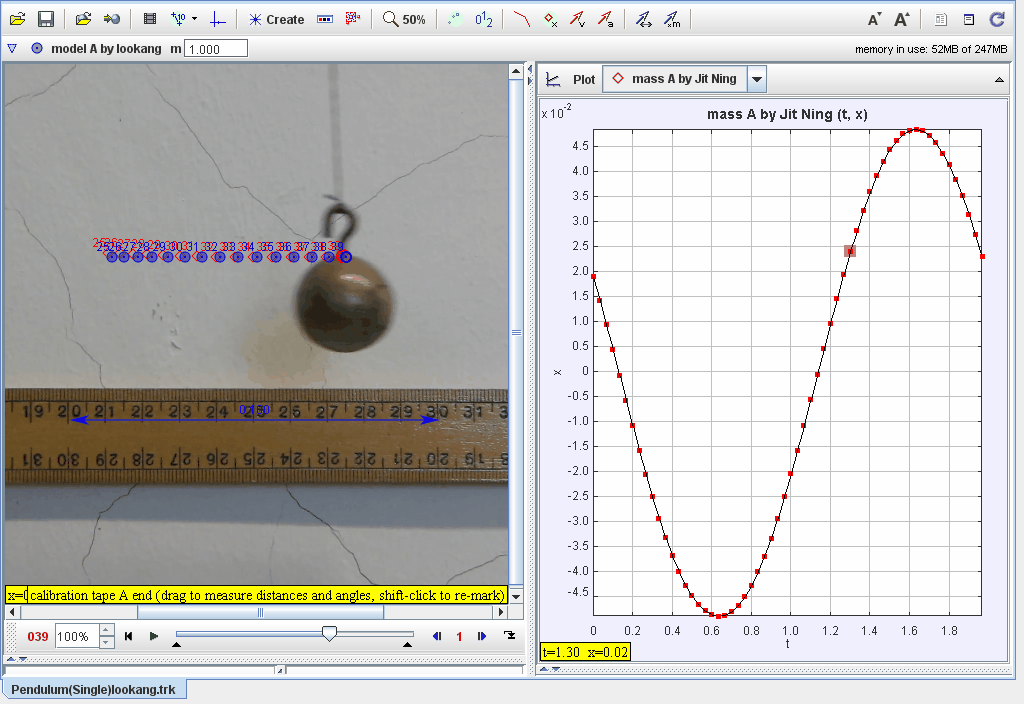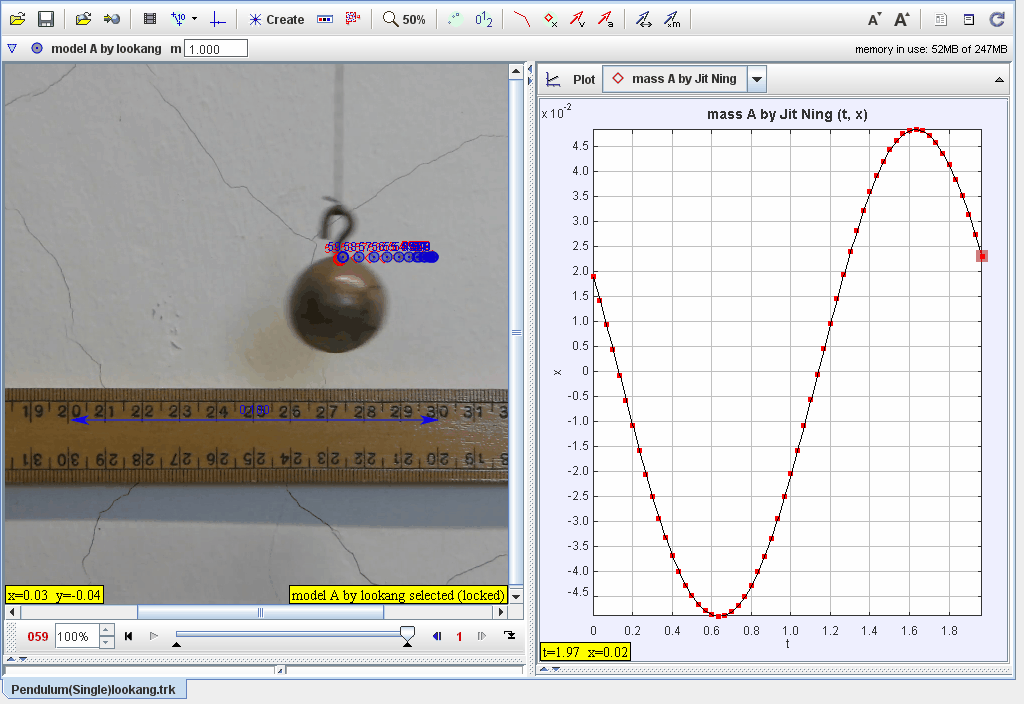
02 Newtonian Mechanics
Big Ideas 0 Computer Models use reasonable approximations to simplify real-world phenomena in order to arrive at useful ways to explain or analyse systems 1. The study of motion involves first studying an idealized system in which complicating factors (like friction) are absent and then transferring this understanding to a real physical process. Analysis of the motion of an object is performed using free-body and vector diagrams, graphical analysis as well as mathematical formulae. 2. There are four fundamental forces in nature: gravitational and electromagnetic forces (which are responsible for our everyday experiences) and strong nuclear and weak forces (which operate only at the sub-atomic scale). Gravitational force (a very weak attractive force between two masses) is very long range and is responsible for the interaction between celestial objects in the Universe as well as the Earth’s gravitational pull on us. Electromagnetic force (a very strong force between two charged objects) is very short range and is responsible for all inter-atomic forces of attraction and repulsion e.g. electrostatic forces, contact forces (normal force, friction, fluid resistance) and magnetic forces. 3. When any two bodies in the Universe interact, they can exchange energy. The law of conservation of energy states that in any closed system (including the Universe), the total quantity of energy remains fixed - energy is transferred from one form to another but none is lost or gained. Many forms of energy can be considered to be either kinetic (motion) energy or potential (stored) energy.4. Newton’s three laws of motion and his law of universal gravitation have been successfully applied to explain and predict the motion of terrestrial and celestial objects. Newton’s laws further show that it is possible to express natural phenomena in terms of a few special rules or laws that can be expressed in mathematical formulae. 5. When any two bodies in the Universe interact, they can exchange momentum. The law of conservation of momentum states that in any closed system (including the Universe) the total quantity of momentum is invariant - momentum can be transferred from one body to another (by an impulse) but none is lost or gained. 6. Many kinds of motion in nature are periodic motions or oscillations. The ideas from a type of oscillation known as simple harmonic motion is applied to explain many physical situations such as waves, sound, alternating electric currents, and light.
Resources from http://mptl.eu/ adapted by M. Benedict, Physics Department, University of Szeged (H) T. Bradfield Northeastern (OK) State University (US) E. Debowska, Physics Department, University of Wroclaw (PL) B. Mason, University of Oklahoma (US) S. Feiner-Valkier Eindhoven University of Technology T. Melder University of Louisiana at Monroe (US) Raimund Girwidz University of Education, Ludwigsburg (G) S. Sen State University of New York, Buffalo (US) L. Mathelitsch, Physics Department, University of Graz (A) I. Ruddock, Physics Department, University of Strathclyde (UK) E. Sassi University of Naples (I) R. Sporken, Physics Department, University of Namur (B)
- Introduction to Chaos and Nonlinear Dynamics: T. Kanamaru, Kogakuin University, and J. M. T. Thompson, Cambridge University. http://brain.cc.kogakuin.ac.jp/~kanamaru/Chaos/e/ This web site contains a wide range of simulations of non-linear systems, including applications of Chaos theory to model operation of the brain. The simulations are very professional and they include descriptions of the systems studied. There are also images and videos of non-linear systems with many links to other pages on chaos theory. This material is suitable for university students. It would be improved by having more teaching examples and problems for students.
- The Pendulum Lab: F.-J. Elmer, University of Basel. http://www.elmer.unibas.ch/pendulum/index.html The Pendulum lab by Franz-Josef Elmer is an extremely thorough investigation of the dynamics of a pendulum. It covers the topic from the simple pendulum to the chaotic motion of the damped and driven pendulum. It includes simulations, reference text, and exercises for the student. Virtual experiments can be performed with background information provided by an extensive set of hyperlinked notes that explains the theory of the system. This material can be used for a wide range of student levels, although much of the material is best suited for advanced undergraduate and graduate students studying dynamical systems.
- PhET - Motion: PhET Research Group, University of Colorado, Boulder. http://phet.colorado.edu/en/simulations/category/physics/motion The PhET resources were recommended in last year’s review of Optics and Waves, and the same comments are appropriate for the materials in Mechanics. The PhET simulations are strongly grounded in research on how students interact with and learn from multimedia. These simulations are designed to create a realistic virtual environment that encourages learners to interact and explore. There is only a very basic guide on how to operate the simulations to encourage student-driven learning. The physics topics and potential learning goals for each simulation are listed and many simulations include examples of learning activities, clicker questions, and virtual labs. A new feature is a rating scheme for these teaching examples. There are 17 different simulations in mechanics covering topics from kinematics and graphing to energy conservation and torque. One drawback of these resources is that there are no indications of the physical models being used for these simulations or definitions of terms.
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 5501
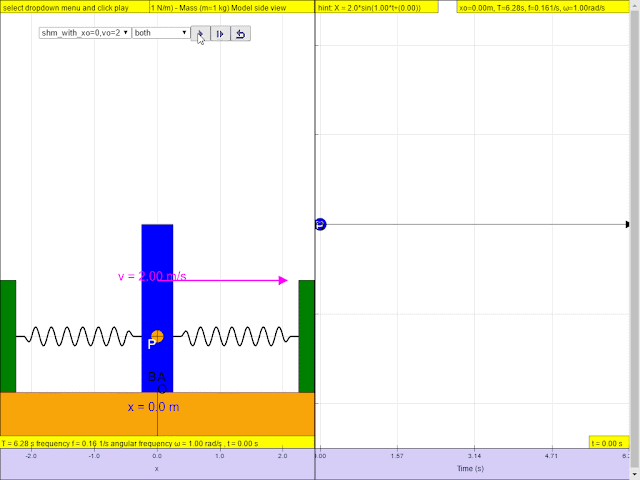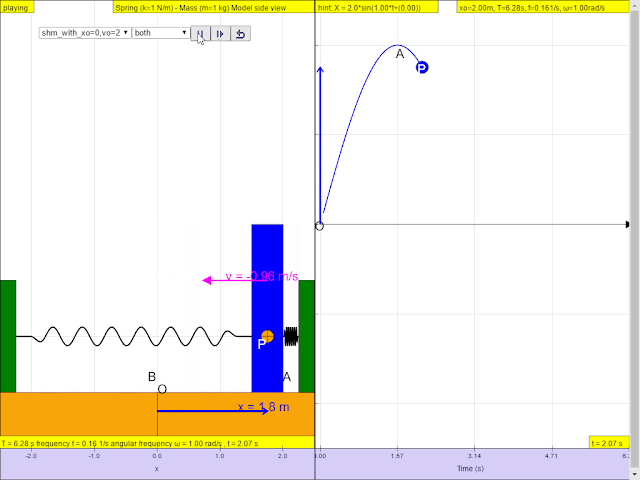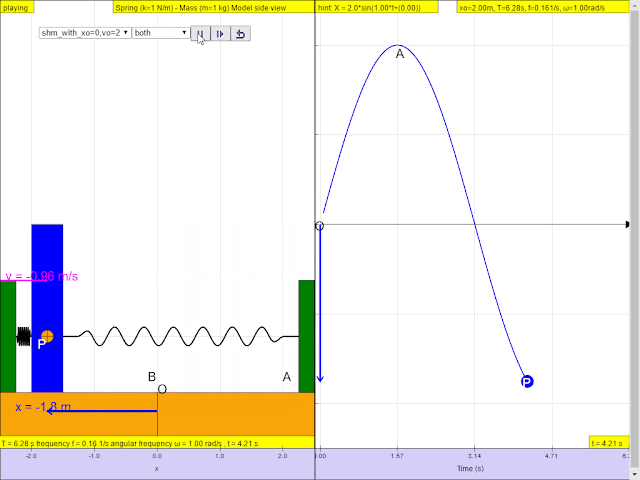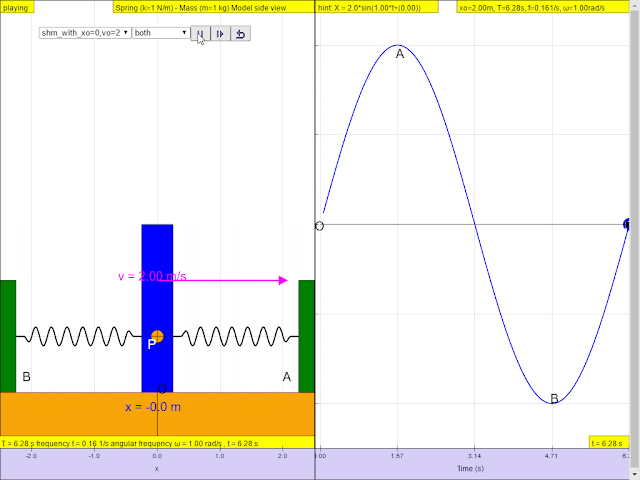
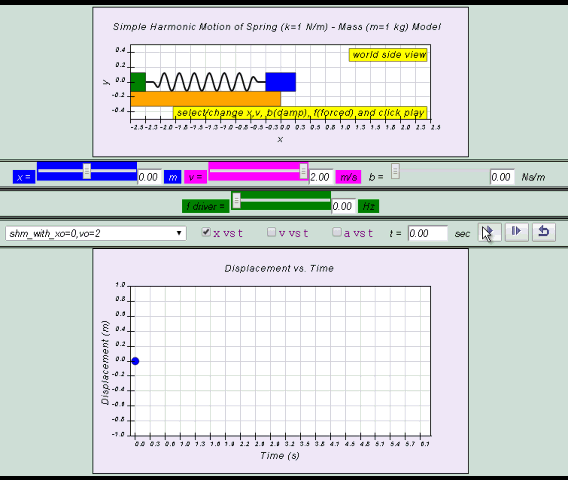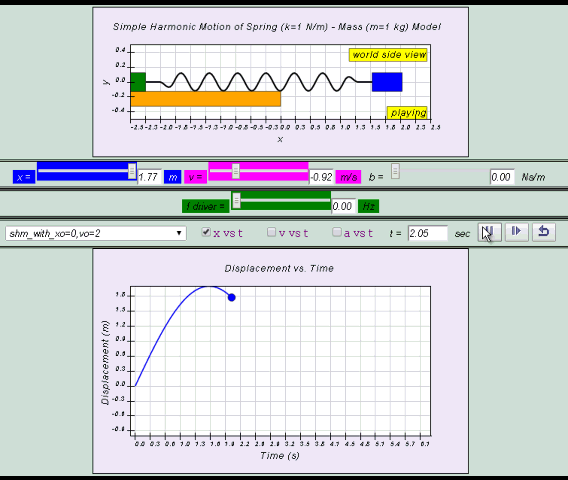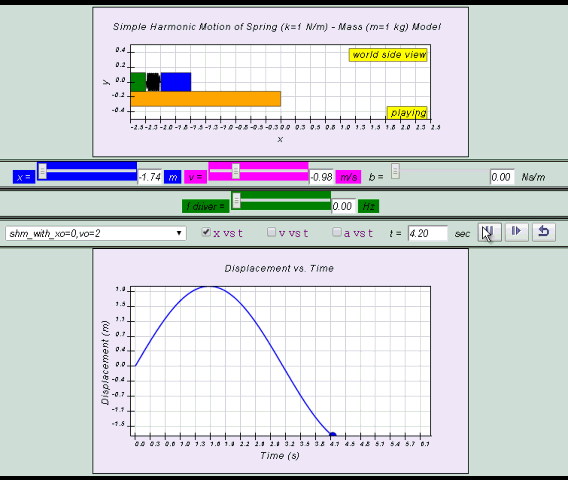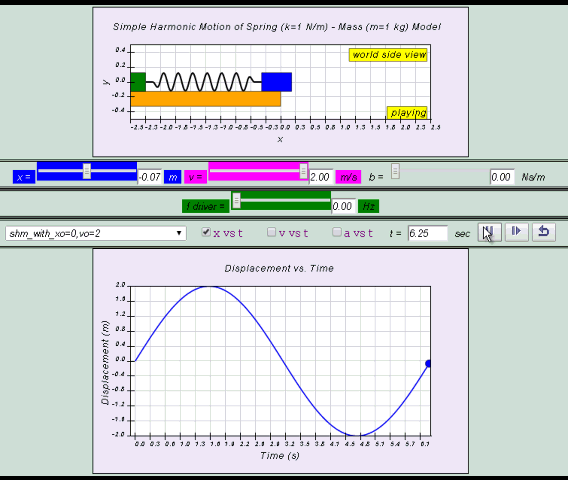
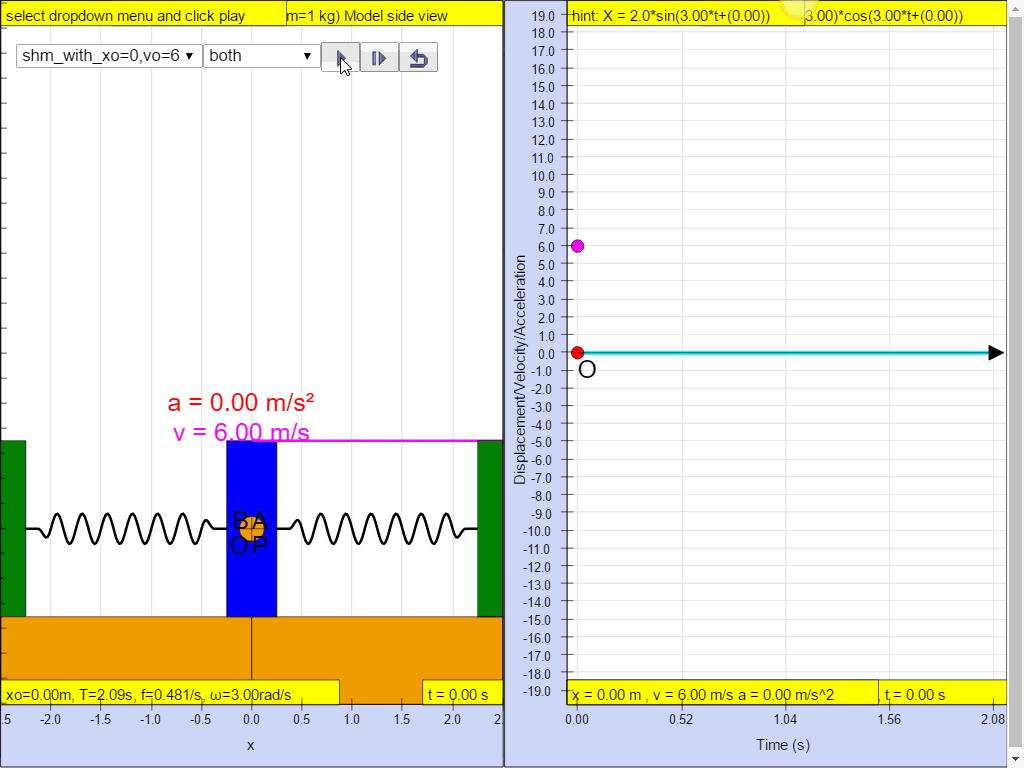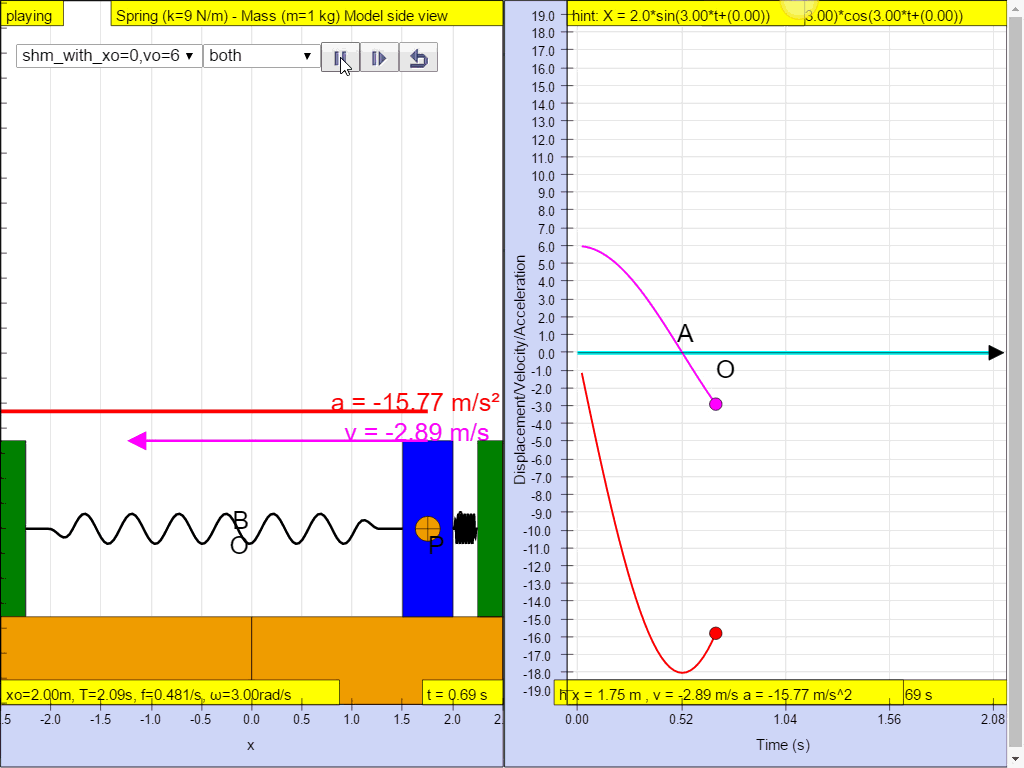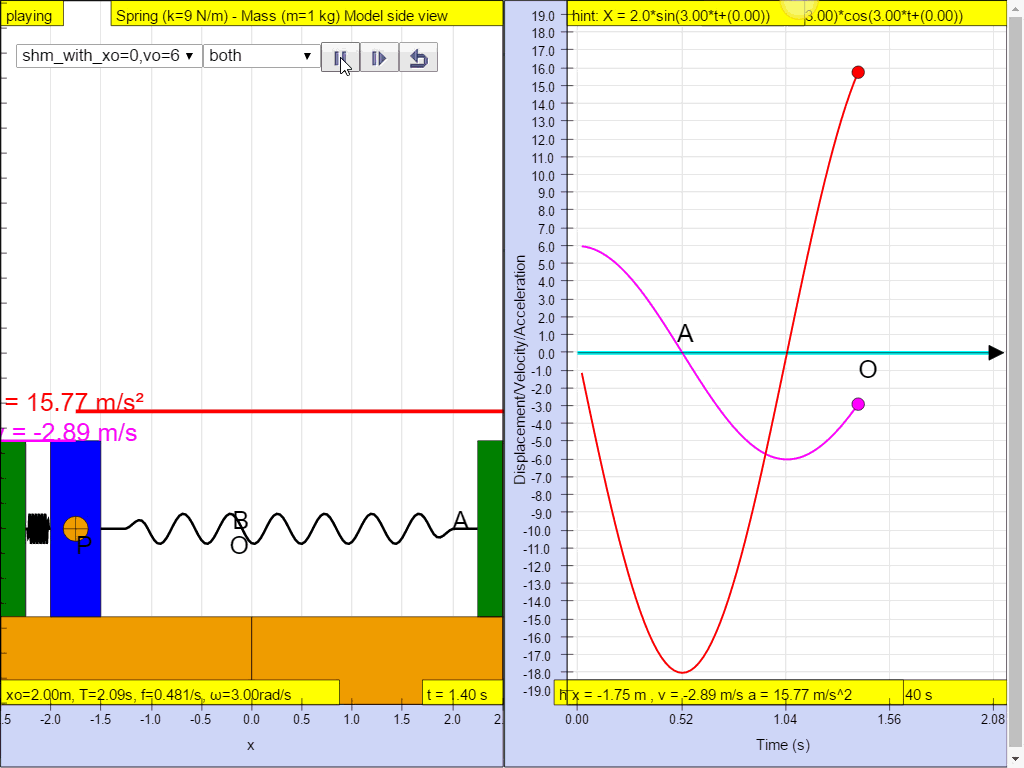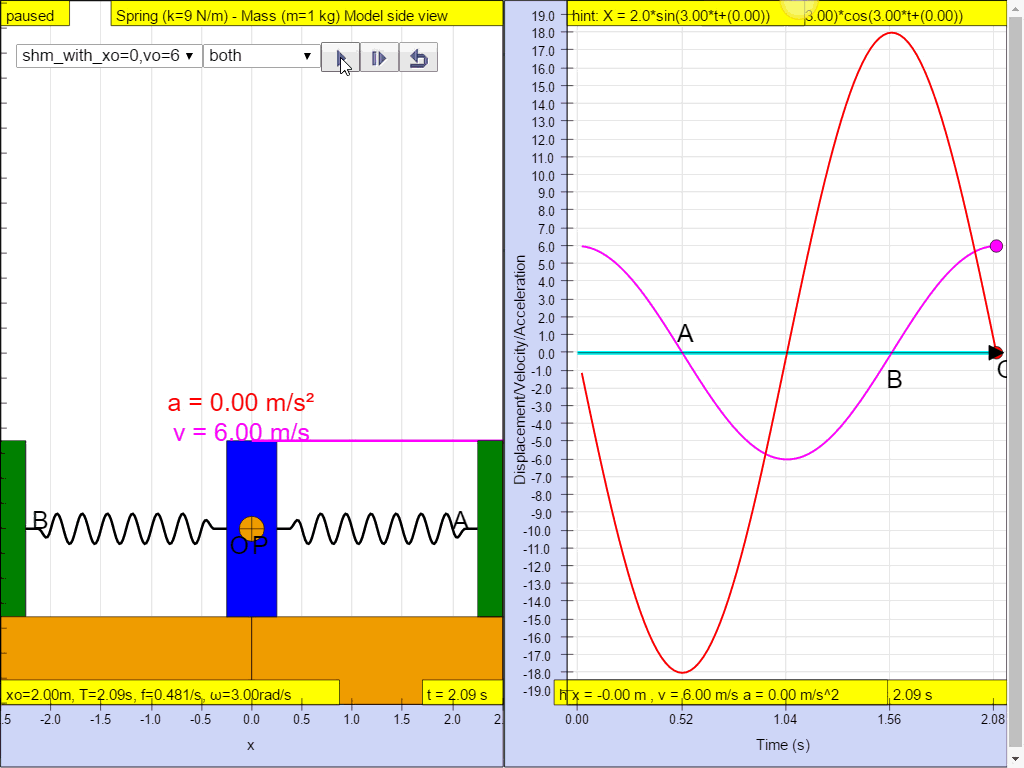
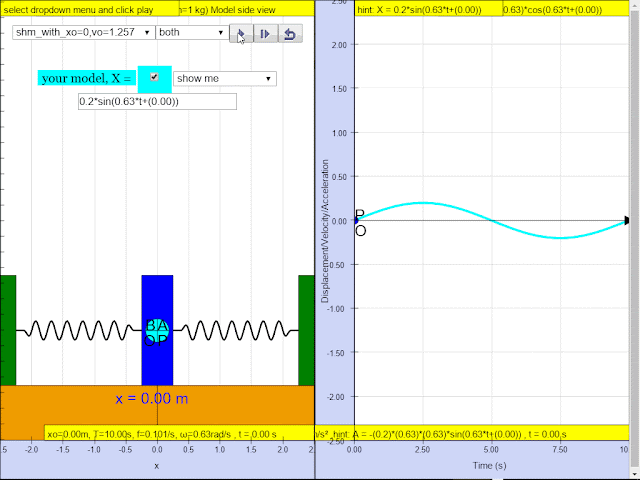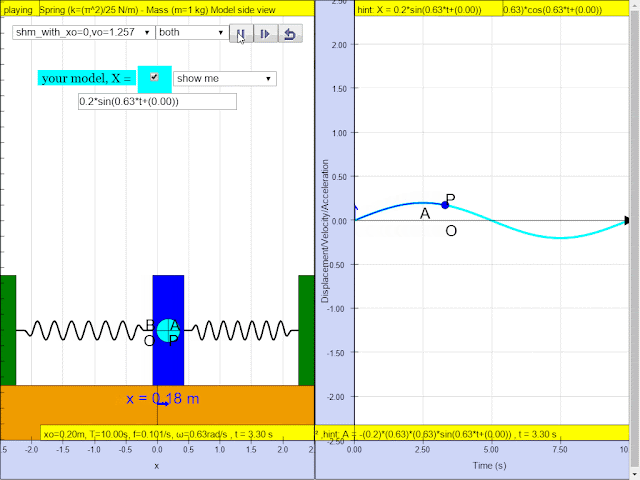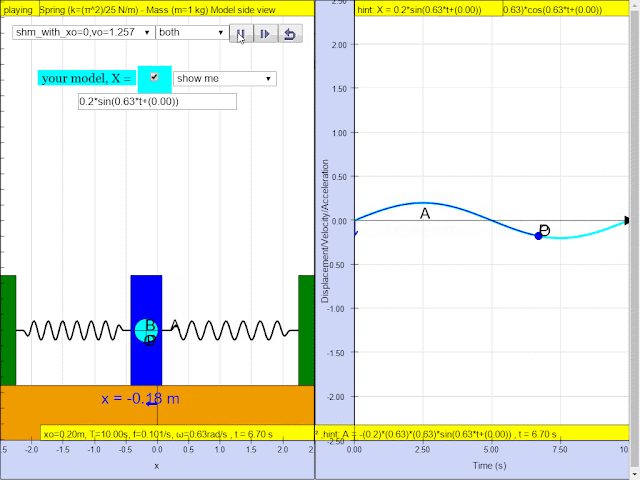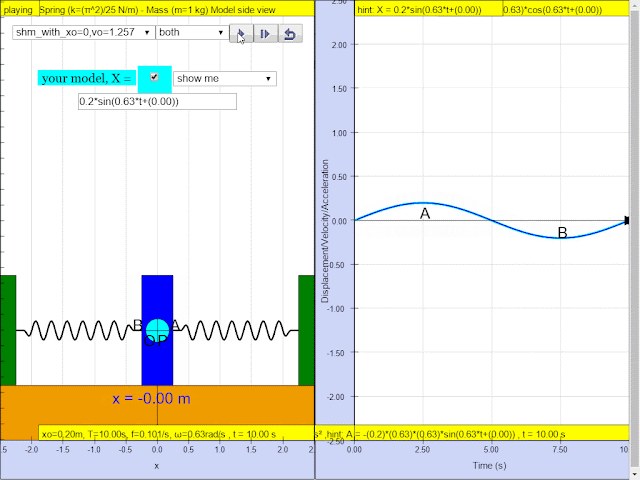
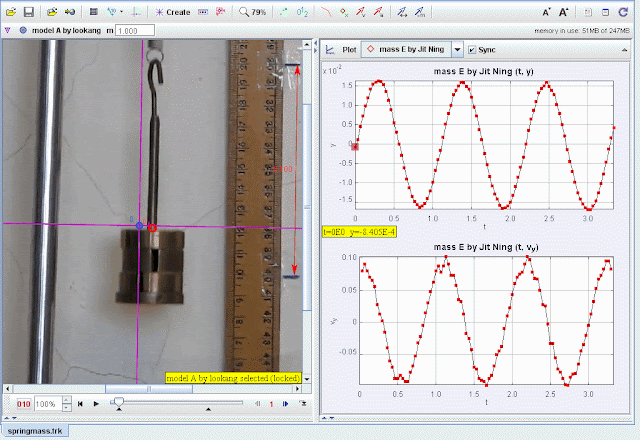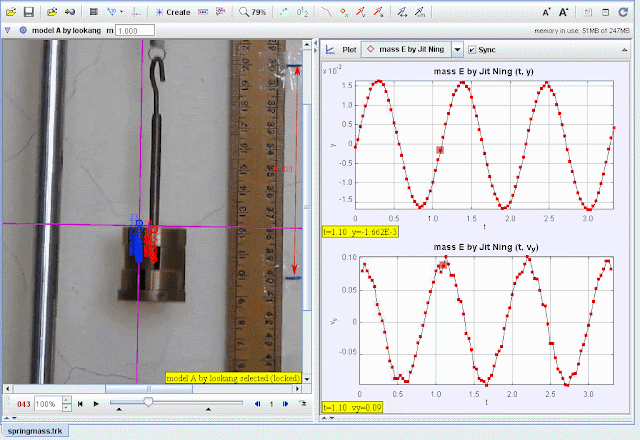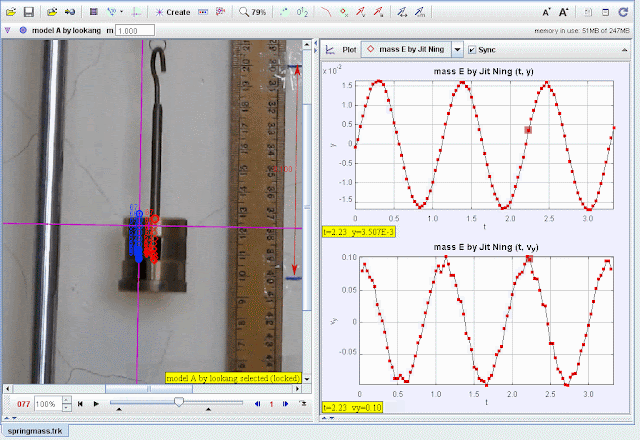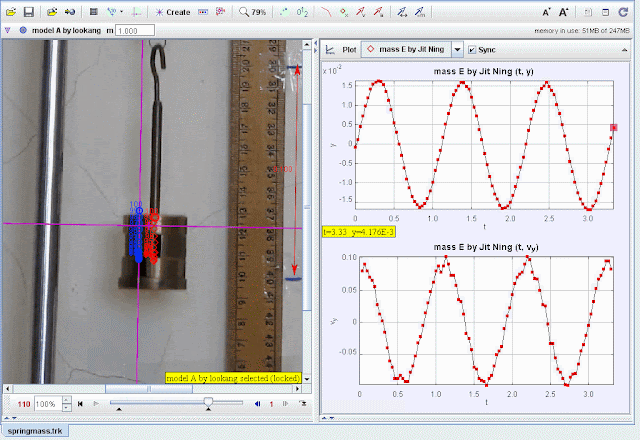
Read more: 10.1.3 Horizontal Spring Mass Model X vs t JavaScript HTML5 Applet Simulation Model
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 7996
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 5128
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 3642
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 4027
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 4025
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 3751
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 3445
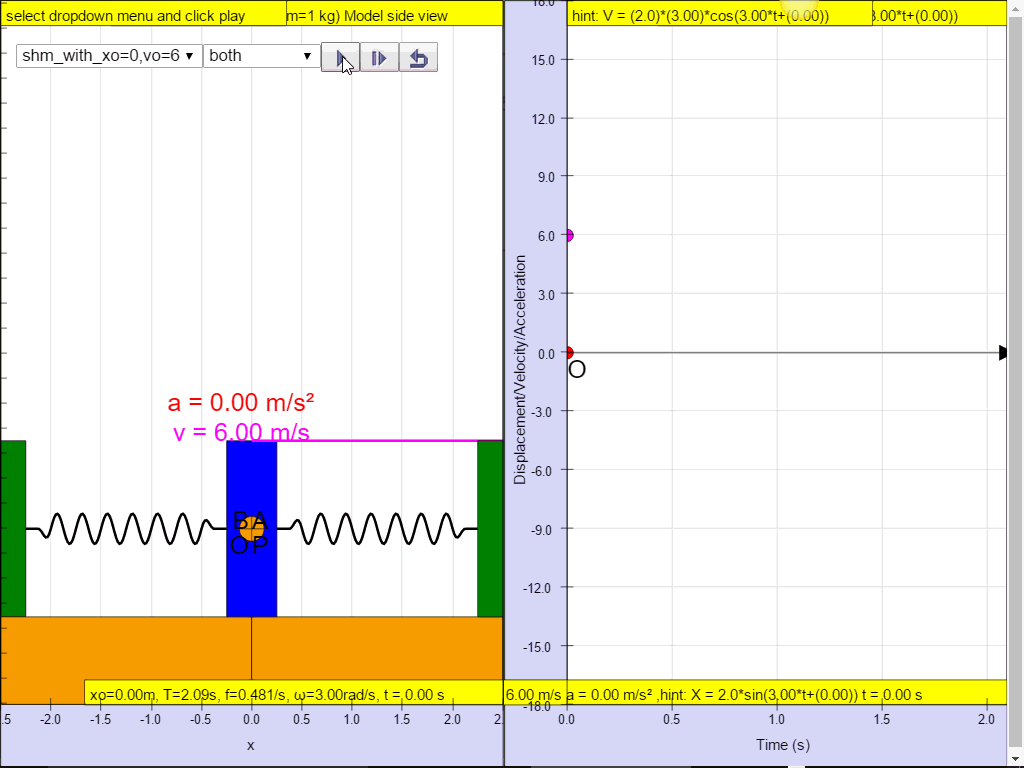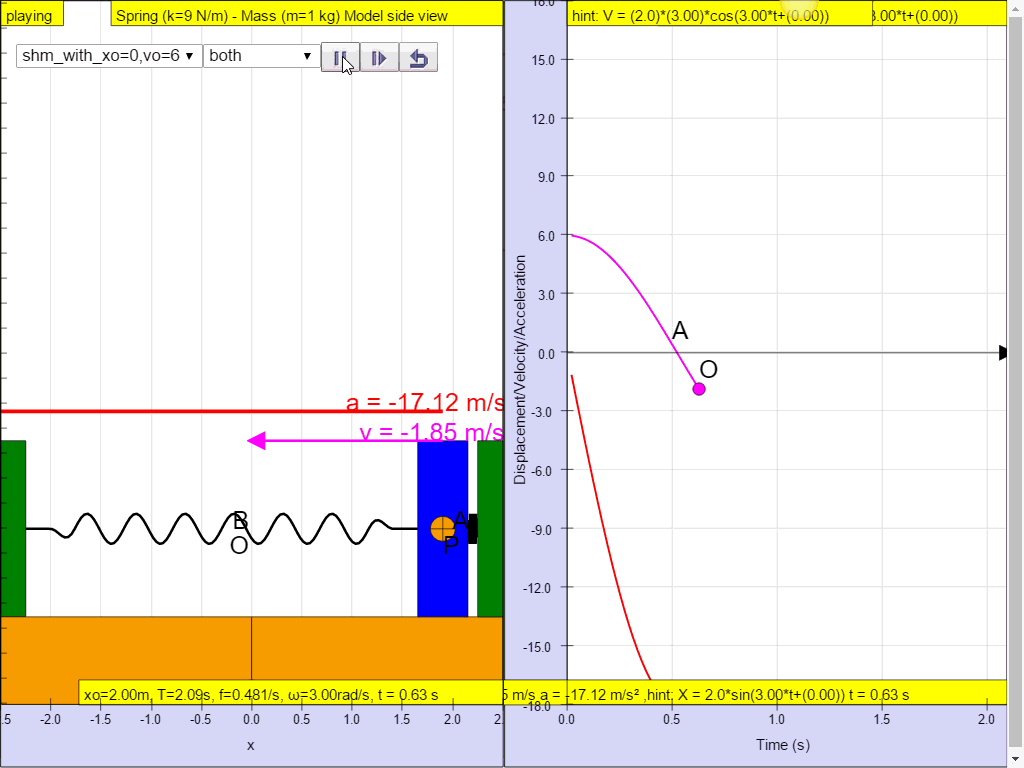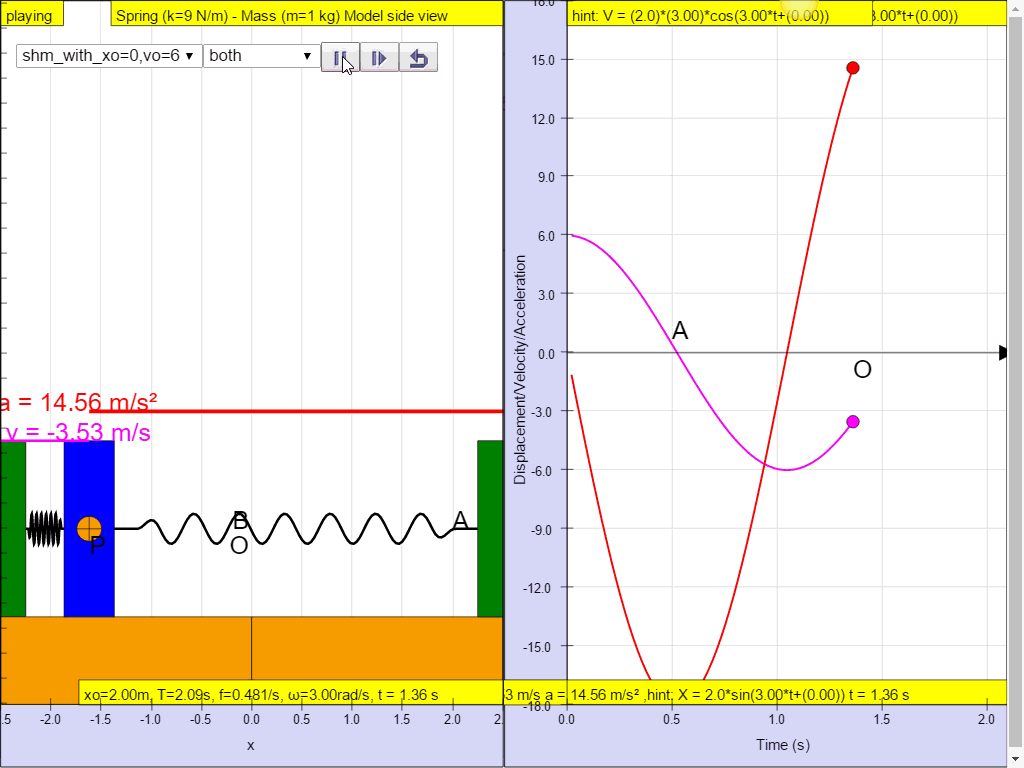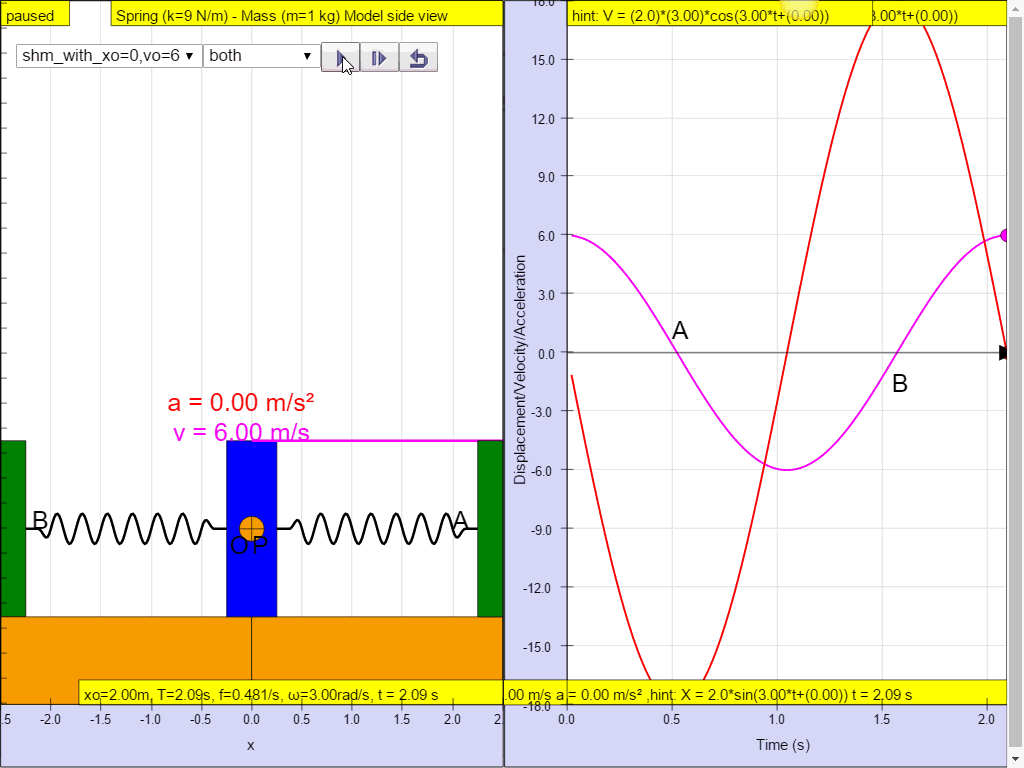
Read more: 10.2.6 Example Spring (k=9 N/m) - Mass (m=1 kg) JavaScript HTML5 Applet Simulation Model
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 3341
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 3871
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 3240
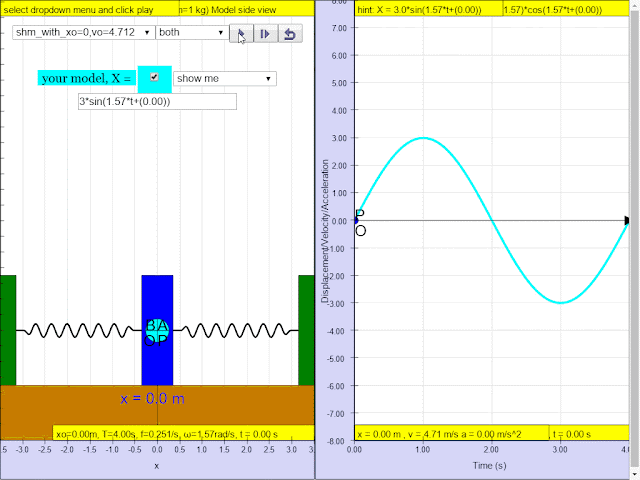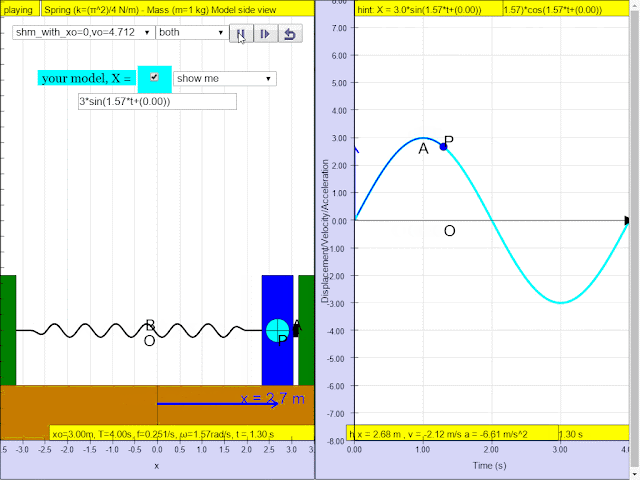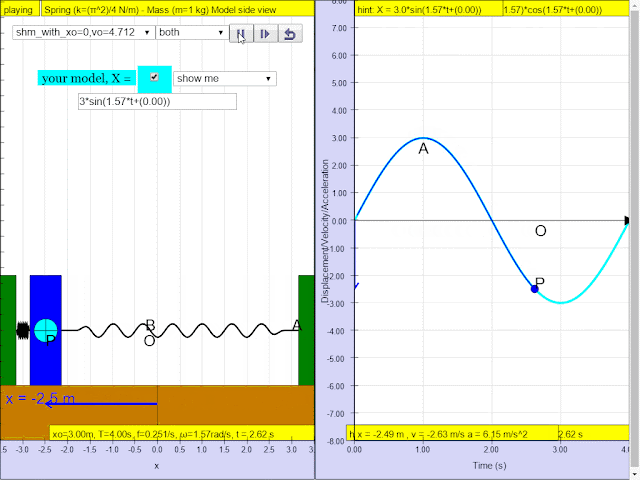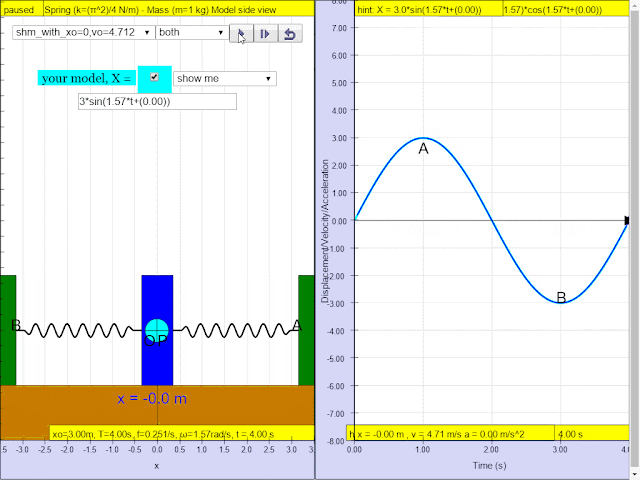
Read more: 10.2.9 Example Spring (k=(π^2)/4 N/m) - Mass (m=1 kg) model versus Time
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 3493
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 4960
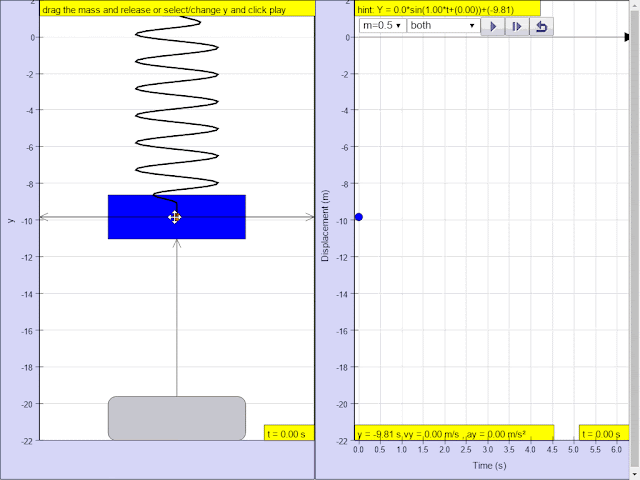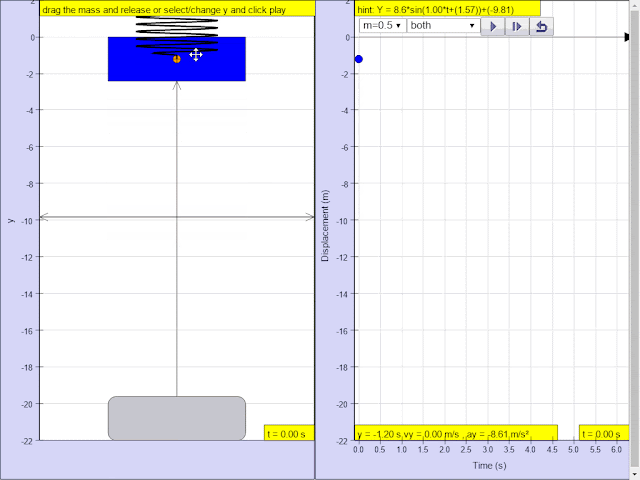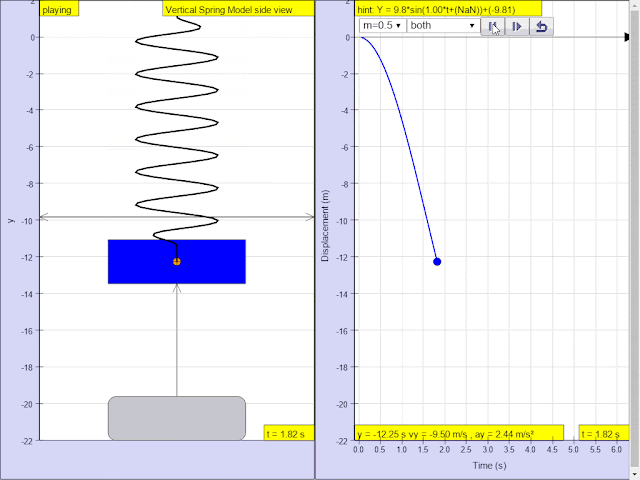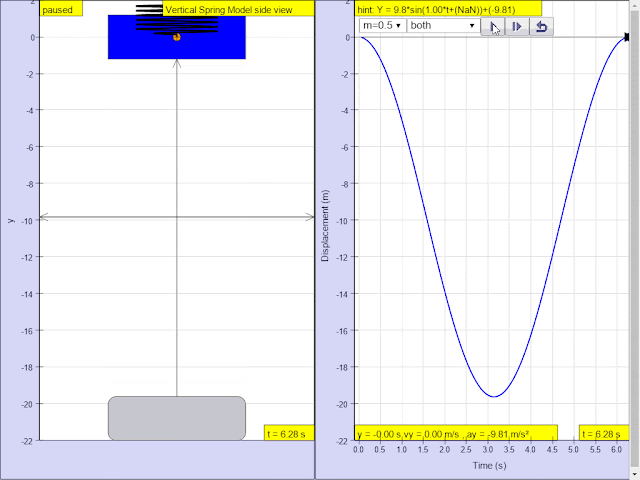
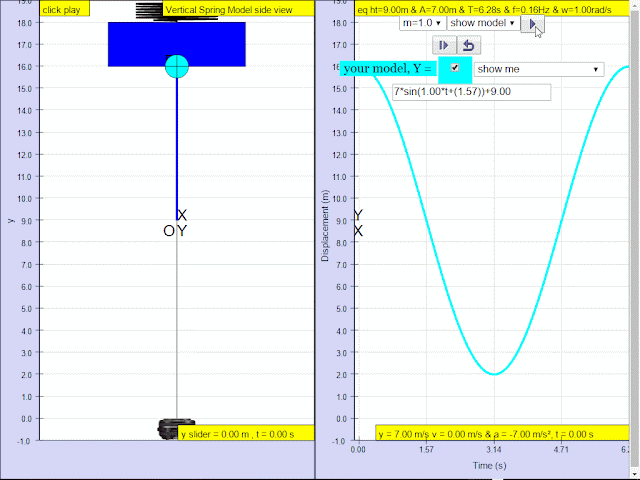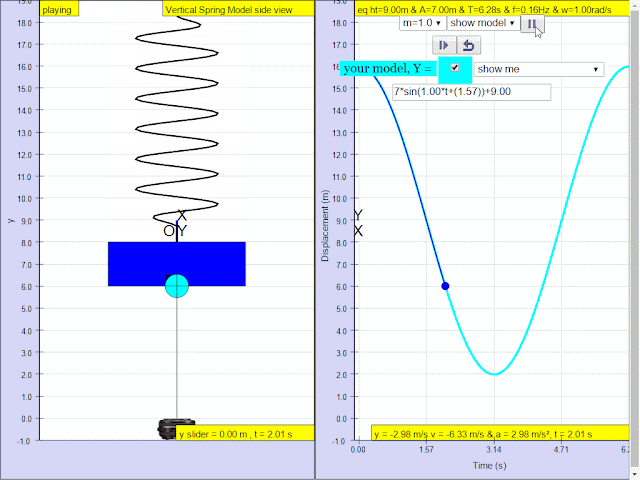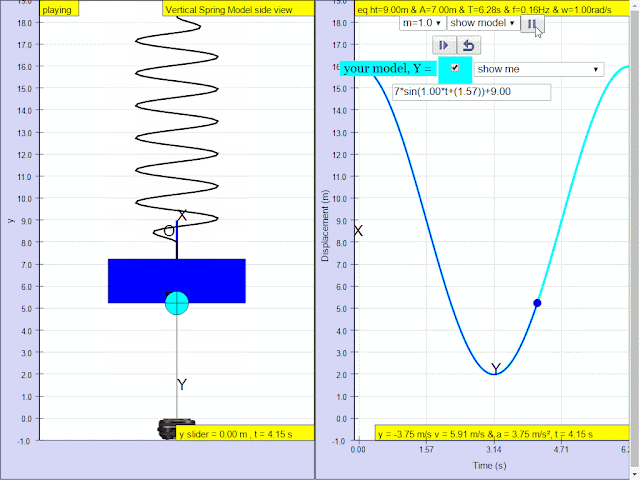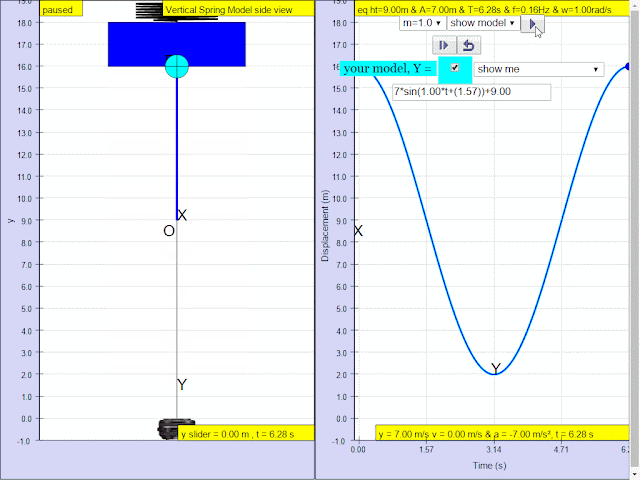
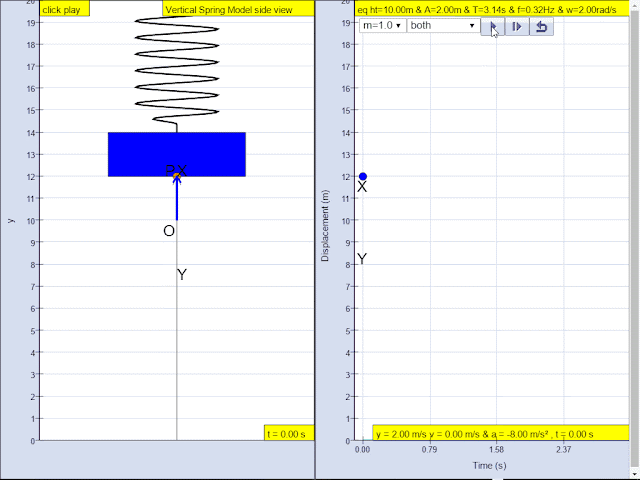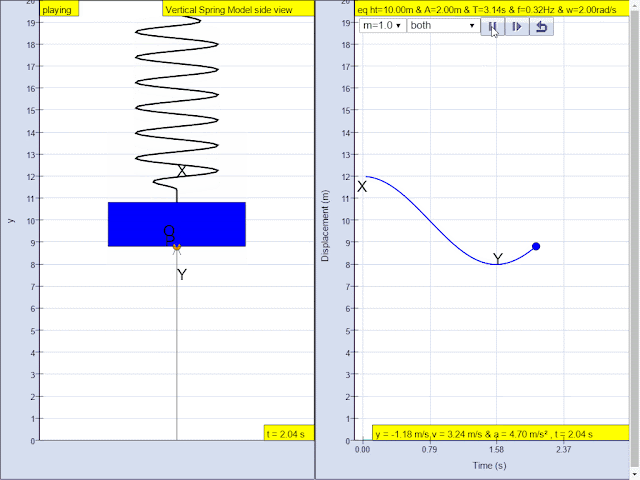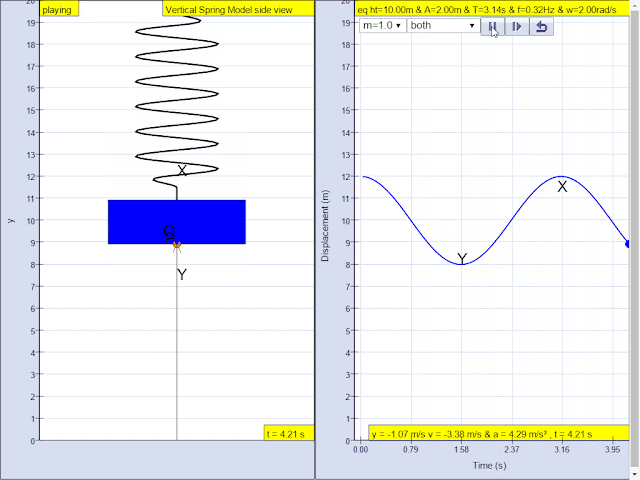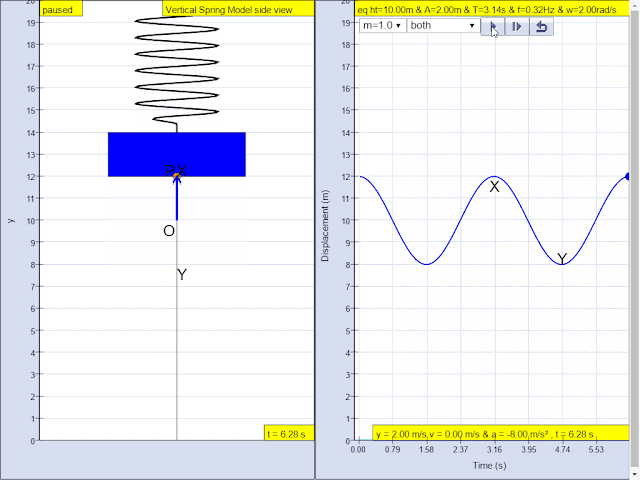
Read more: 10.2.11 Example Vertical Spring Mass JavaScript HTML5 Applet Simulation Model
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 3768
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 3874
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 4087
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 4641
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 3744
Read more: 10.4.3 Example JavaScript HTML5 Applet Simulation Model
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 4645
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 4137
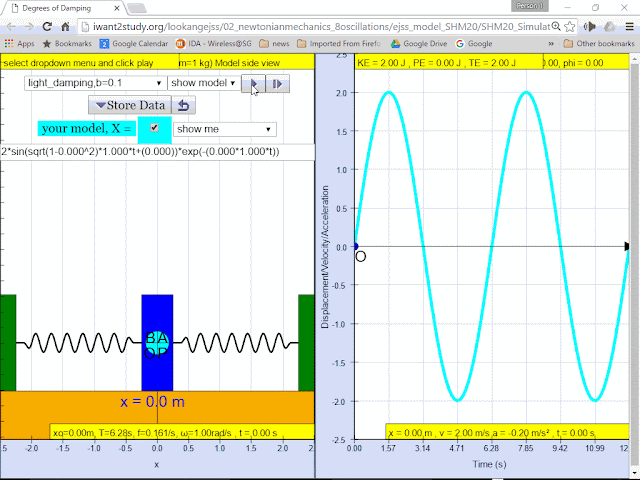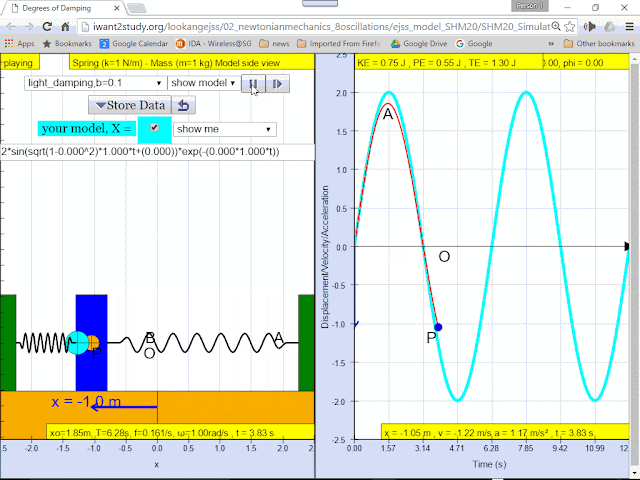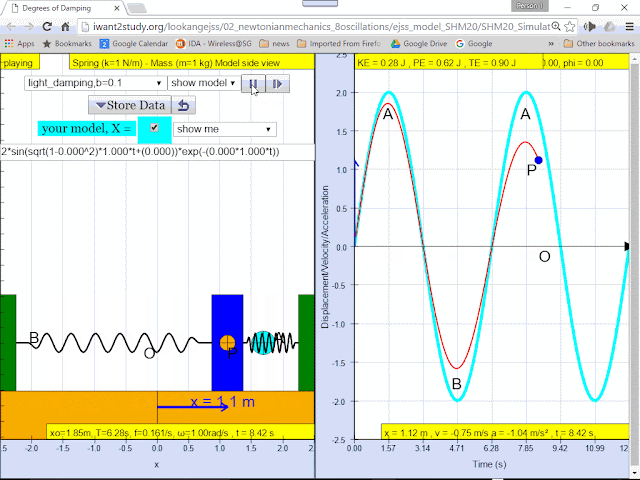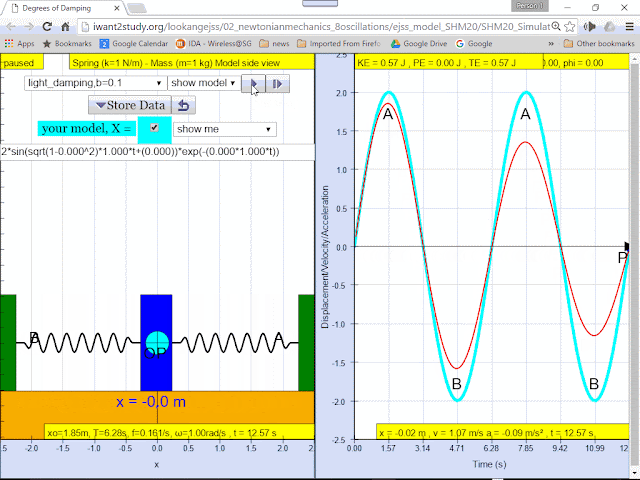
Read more: 10.6 Degrees of Damping HTML5 Applet Simulation Model
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 3839
Read more: 10.6.4.1 Car suspension HTML5 Applet Simulation Model
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 3427
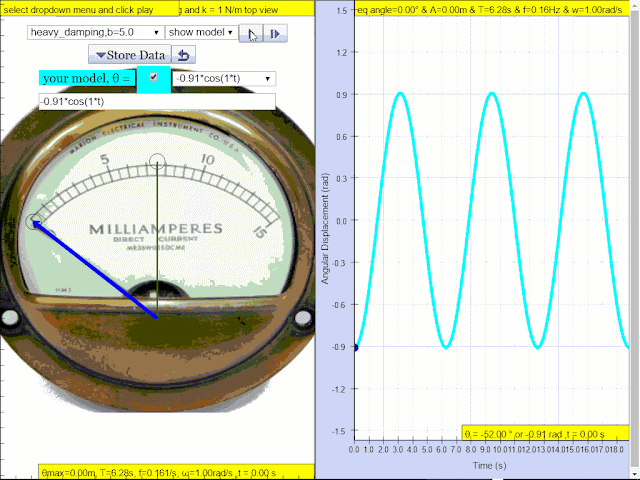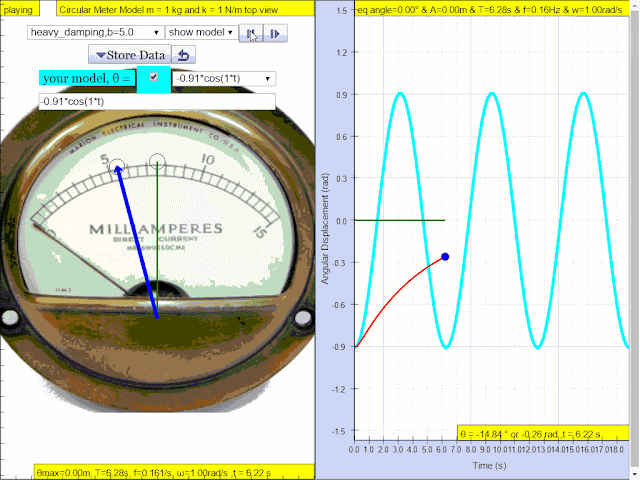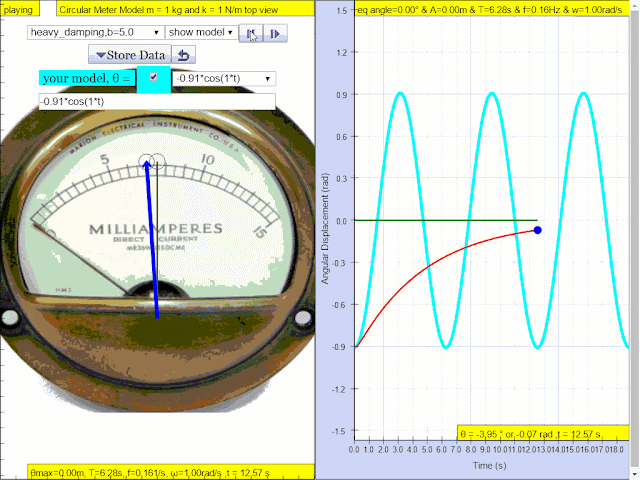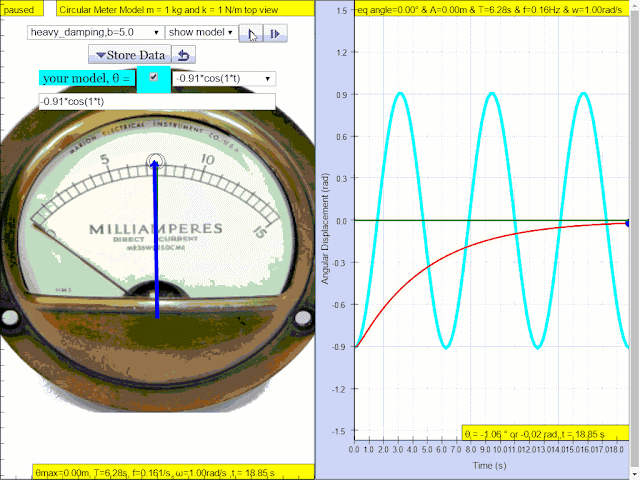
Read more: 10.6.4.2 Moving-coil meters HTML5 Applet Simulation Model
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 4882
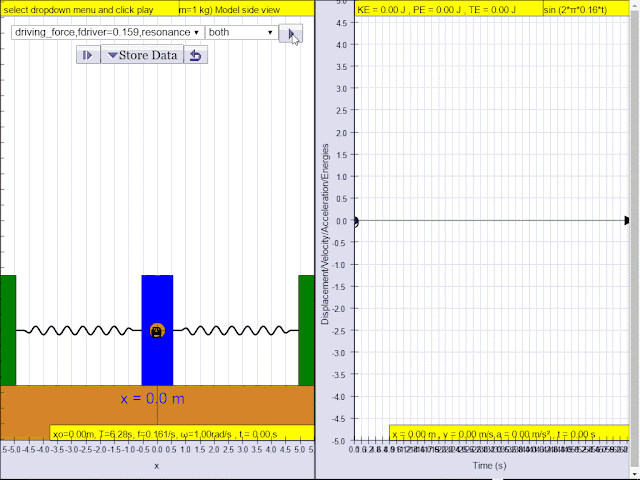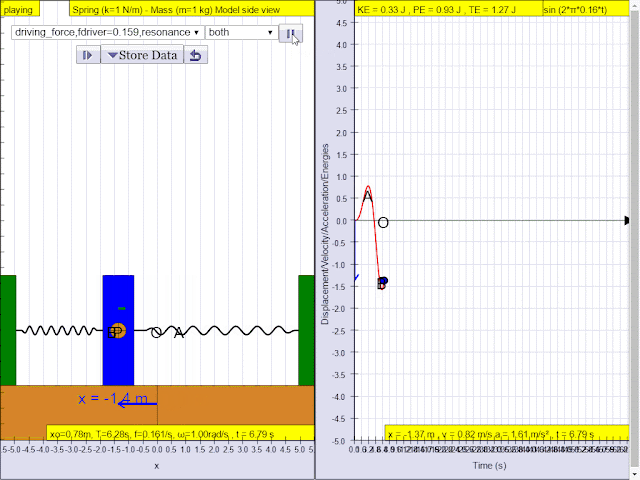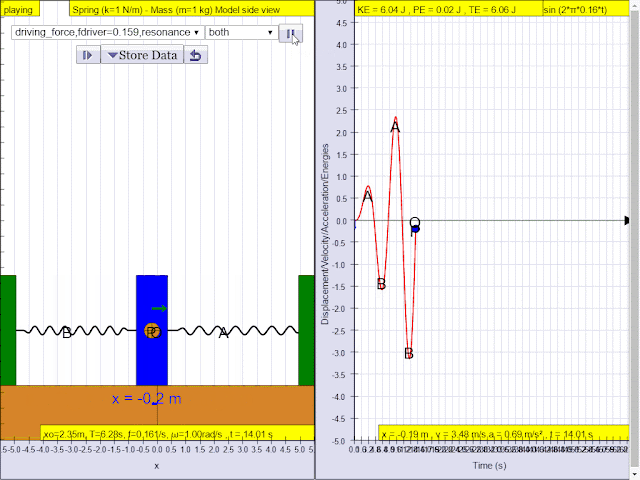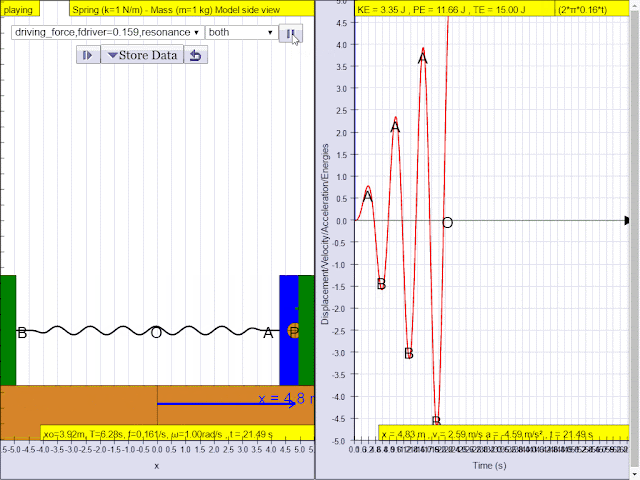
Read more: 10.7 Forced Oscillations and Resonance HTML5 Applet Simulation Model
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 4775
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 6041
Read more: Harmonic Oscillator Virtual Lab HTML5 Applet Simulation Model
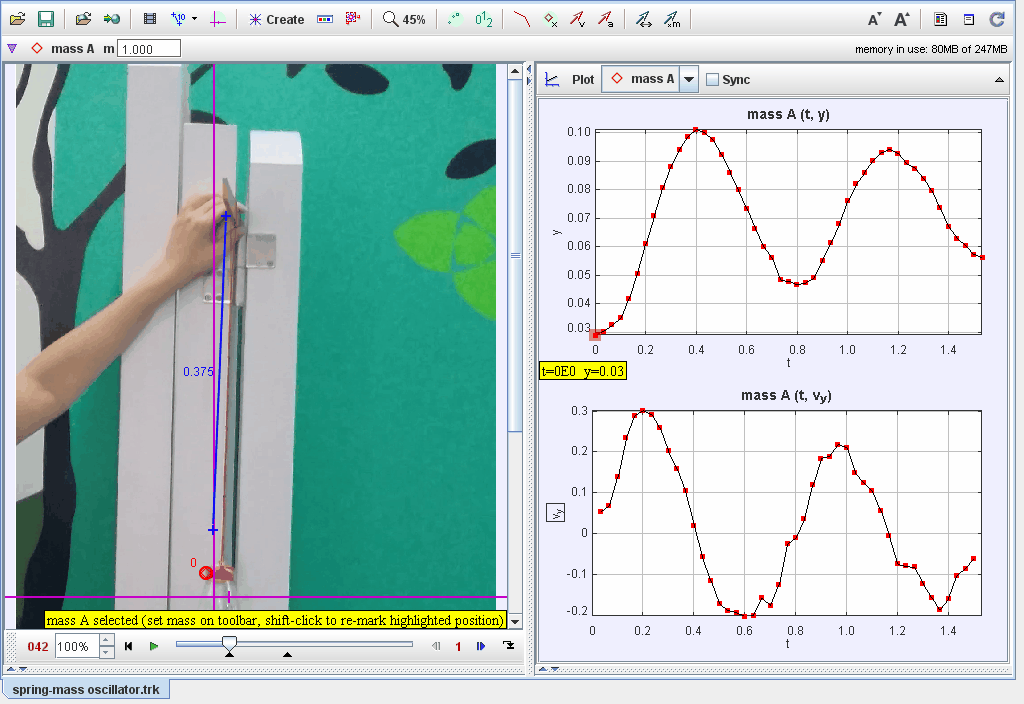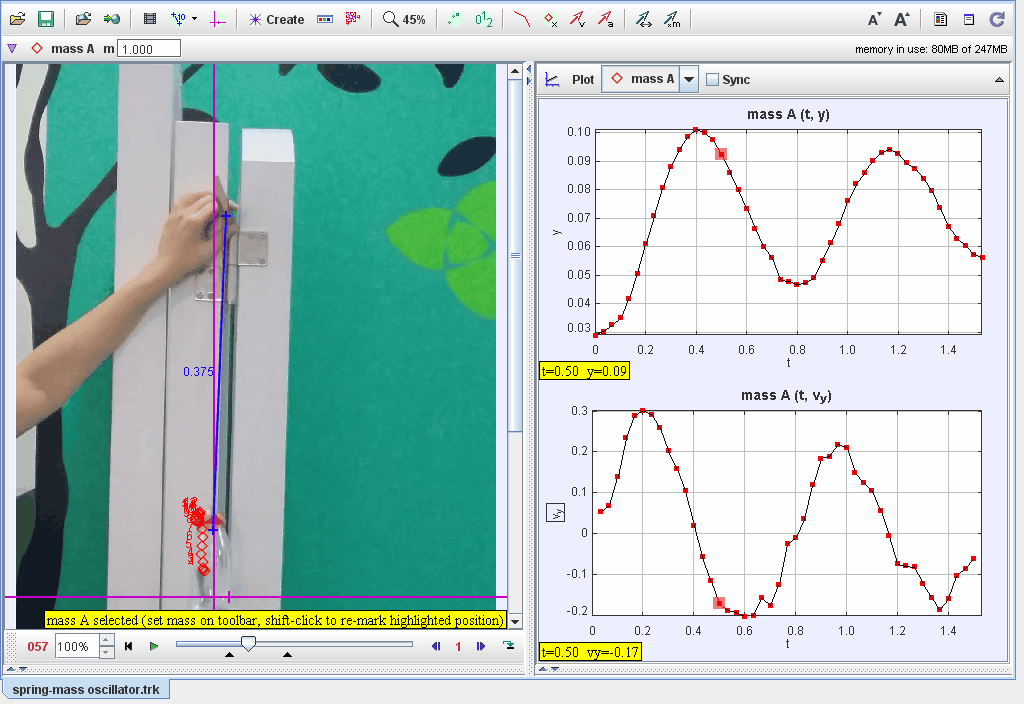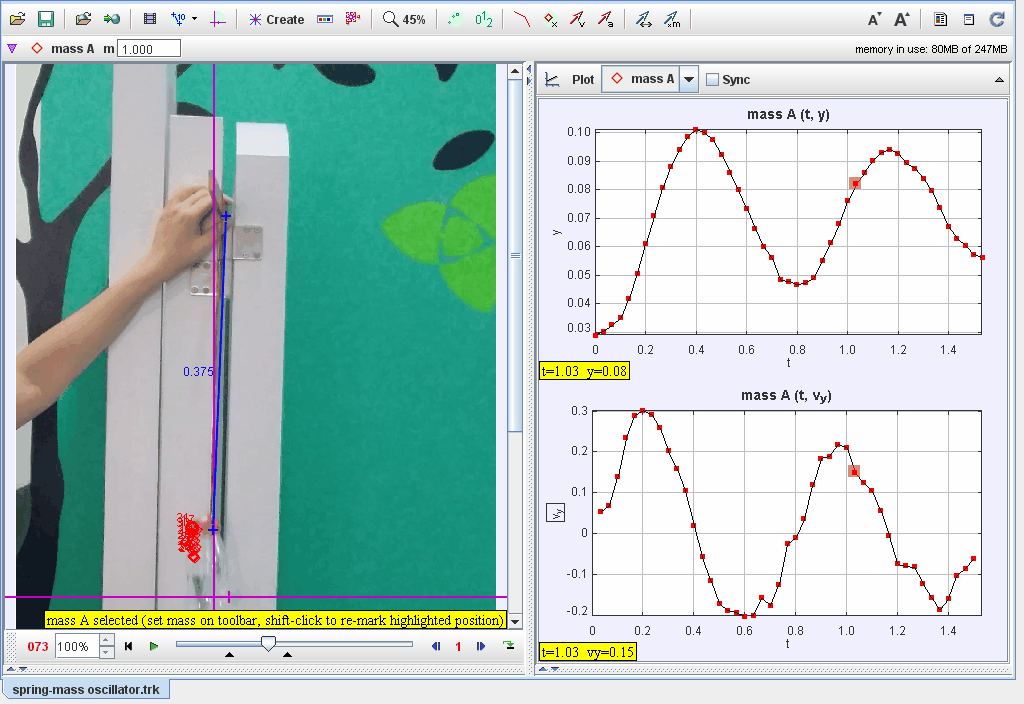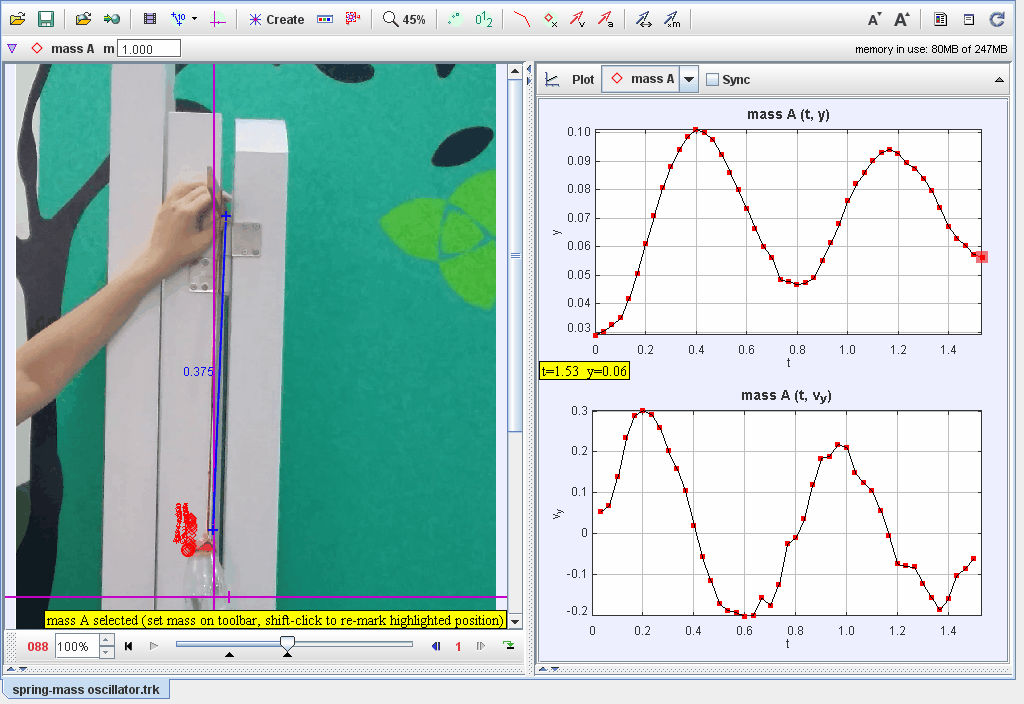
Block and Spring on an Inclined Plane JavaScript HTML5 Applet Simulation Model by Wolfgang Christian
- Details
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 4856
- Details
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 3291
- Details
- Written by leongster
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 2992
- Details
- Written by leongster
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 2696
- Details
- Written by leongster
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 2834
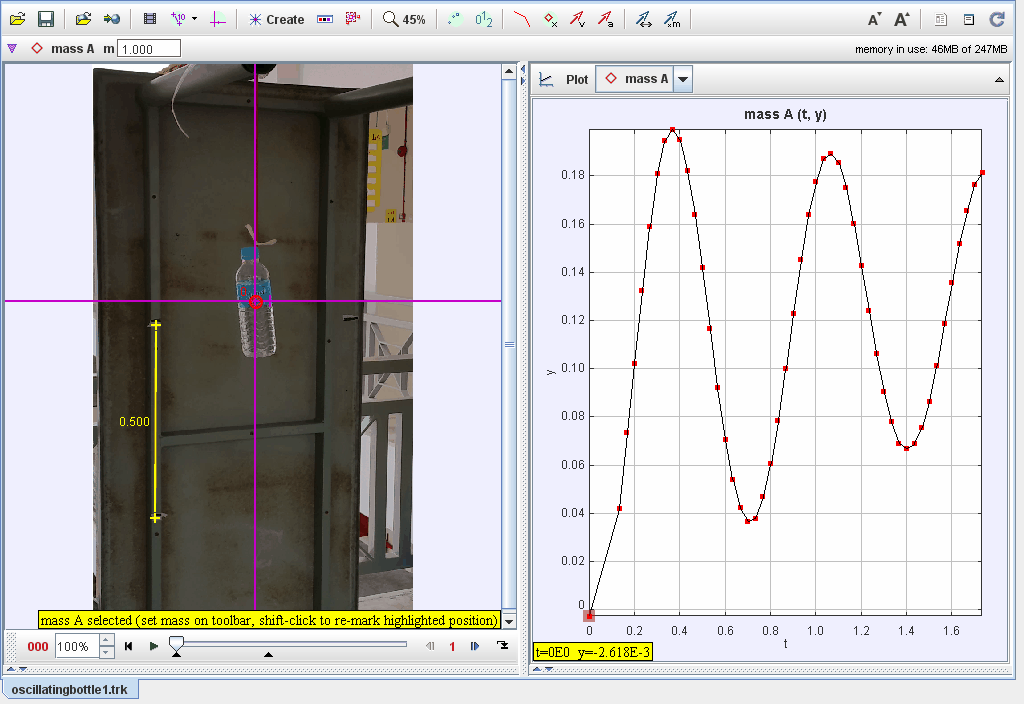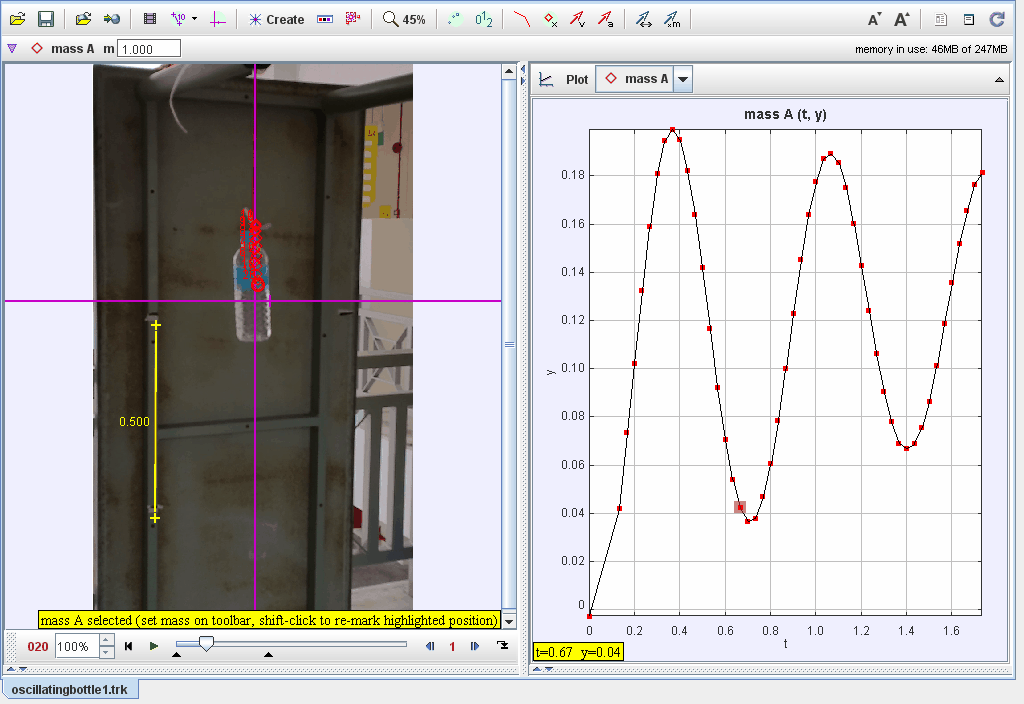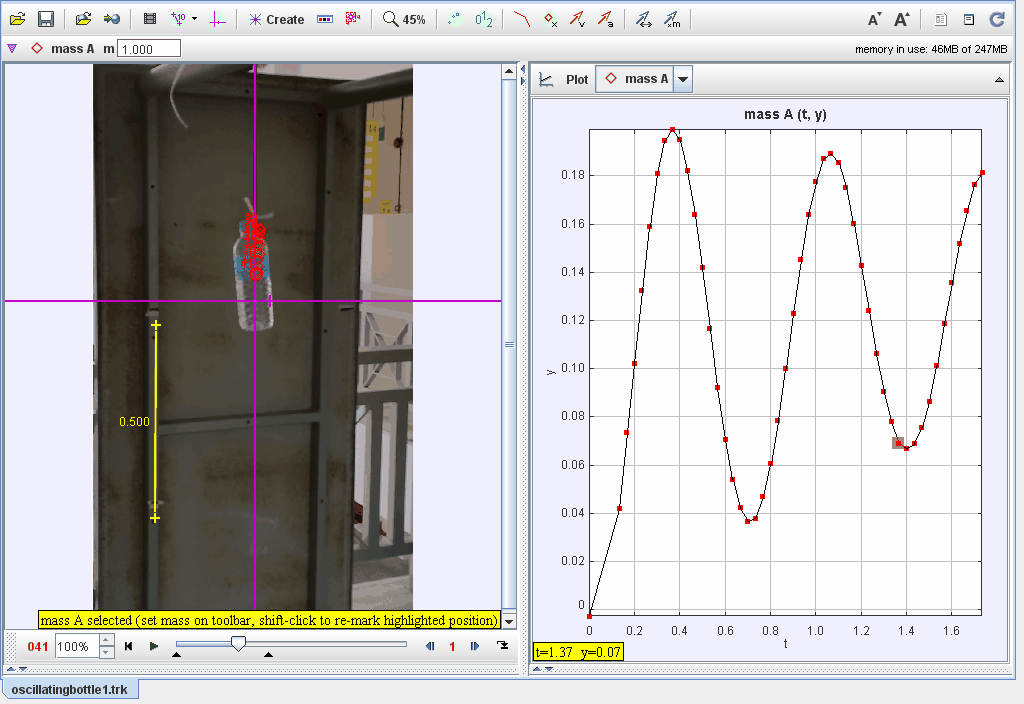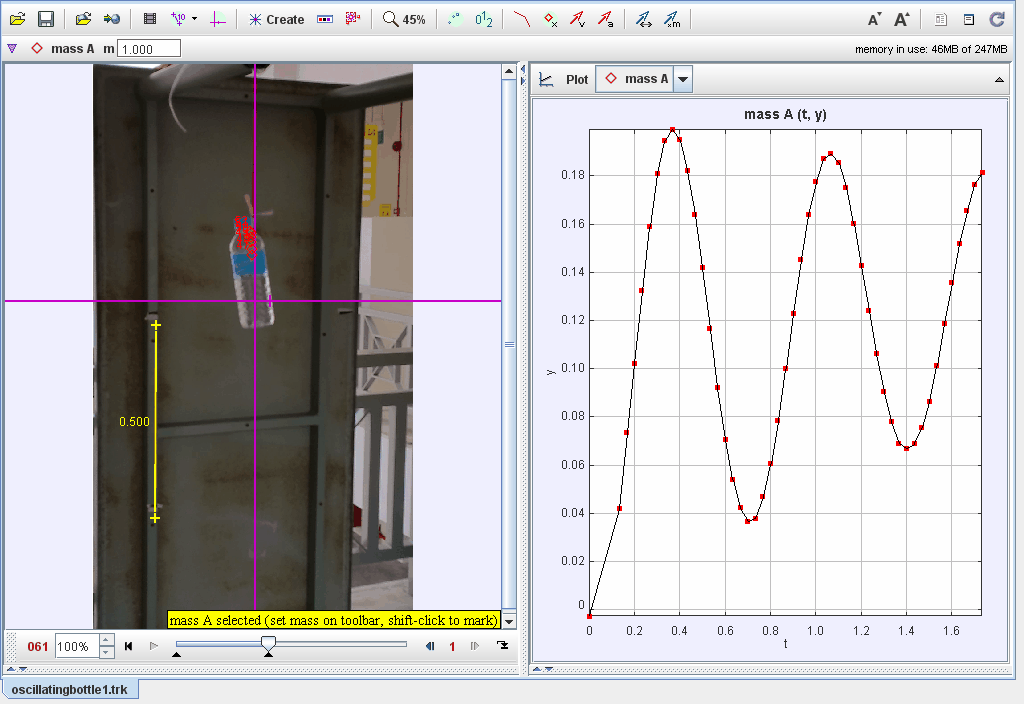
Read more: Tracker Oscillating up and down 2 bottles with model
- Details
- Written by leongster
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 3020
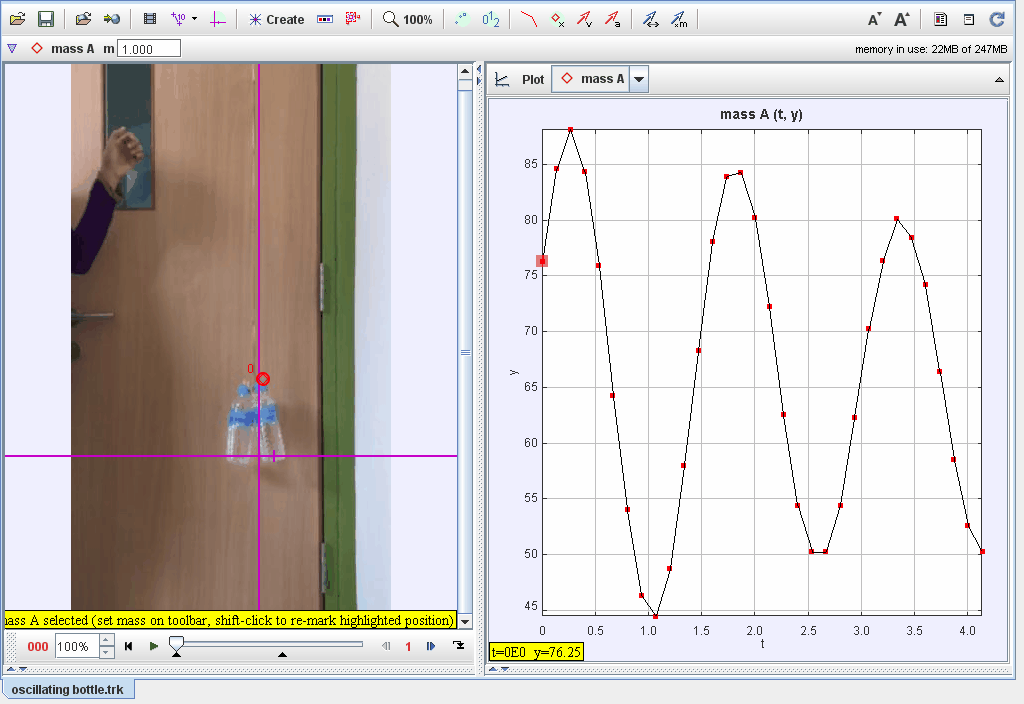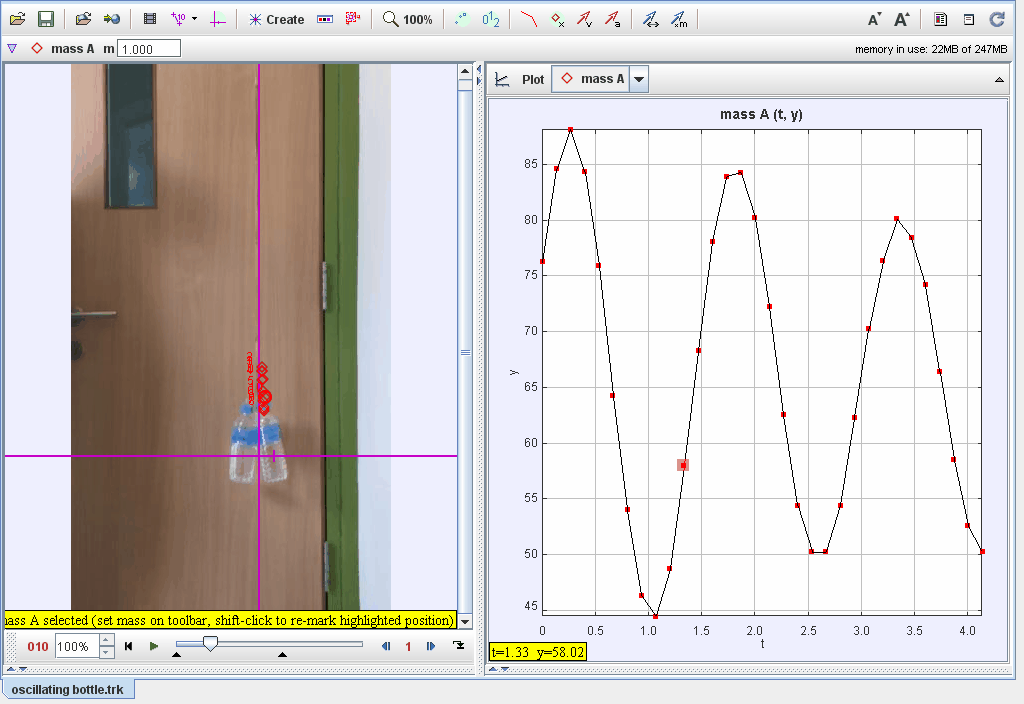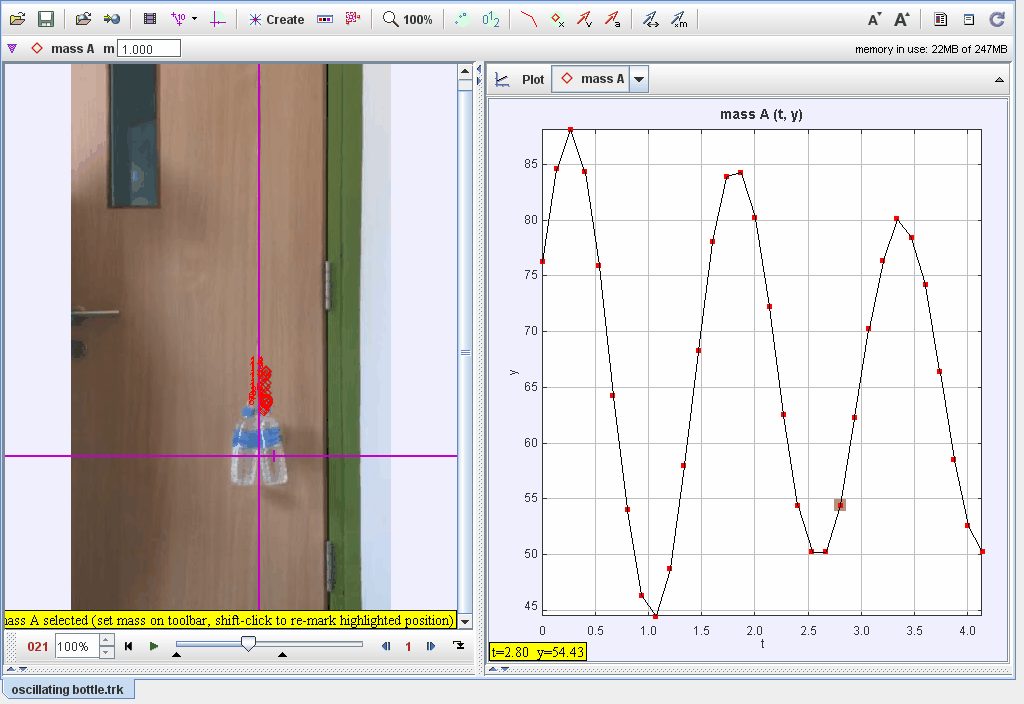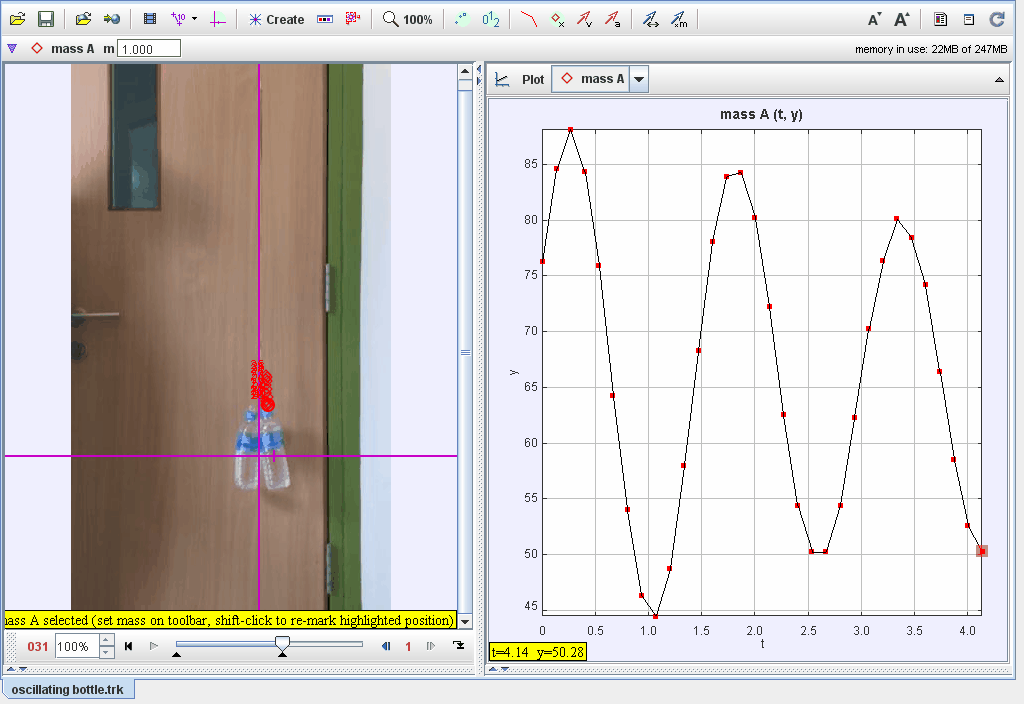
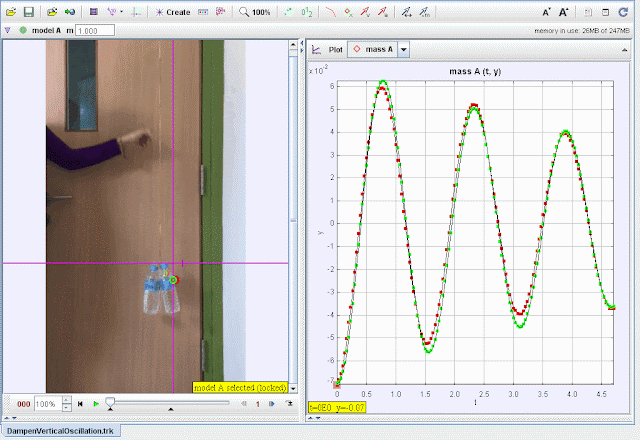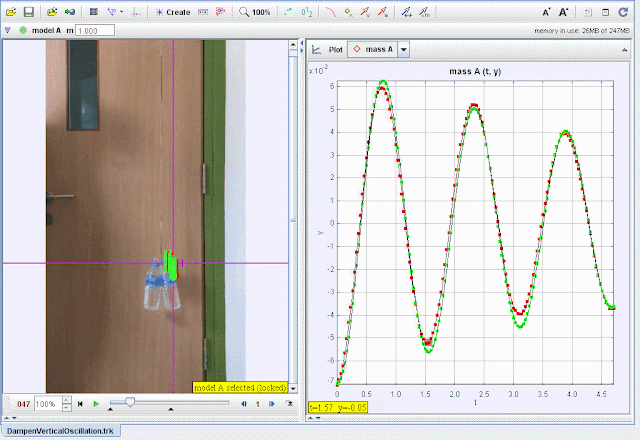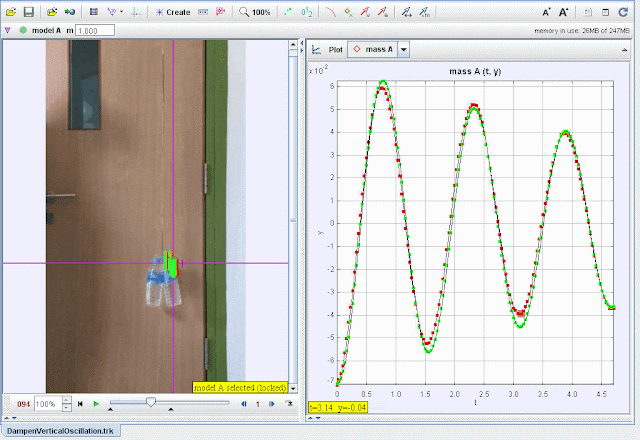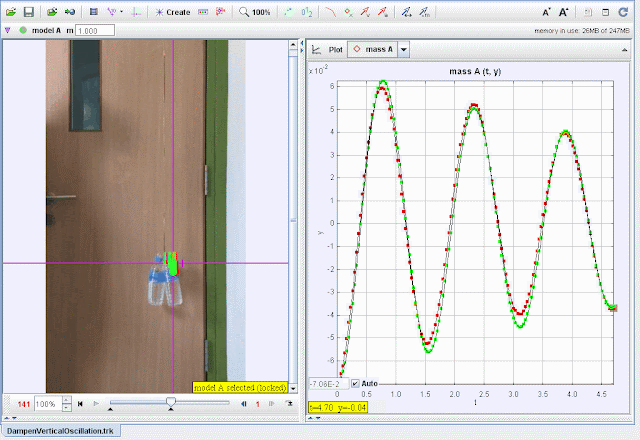
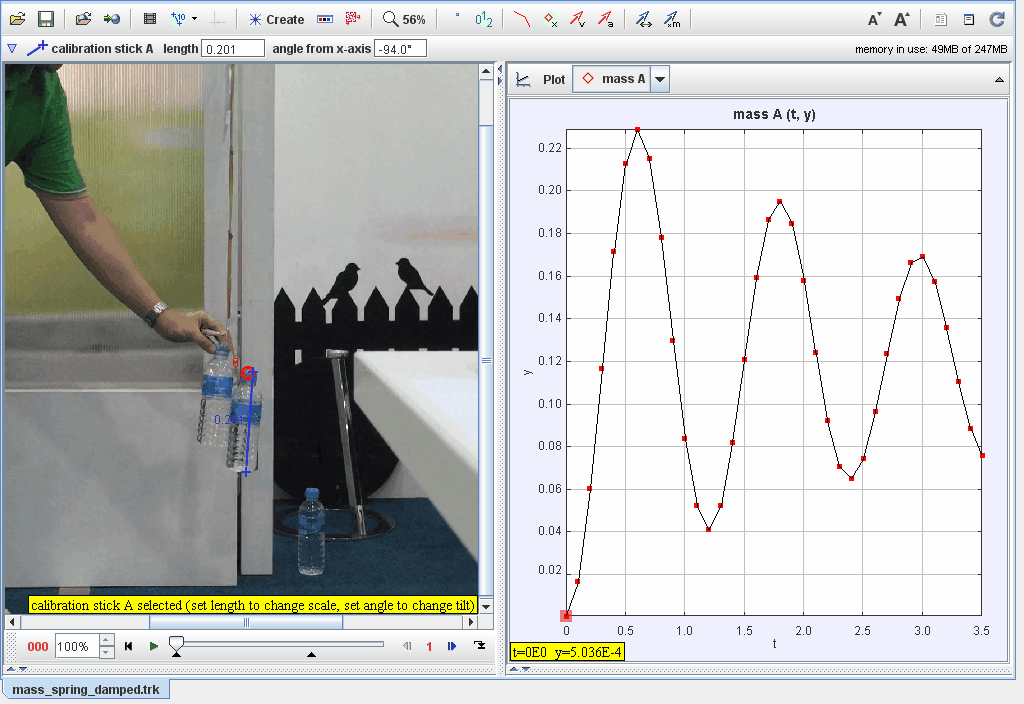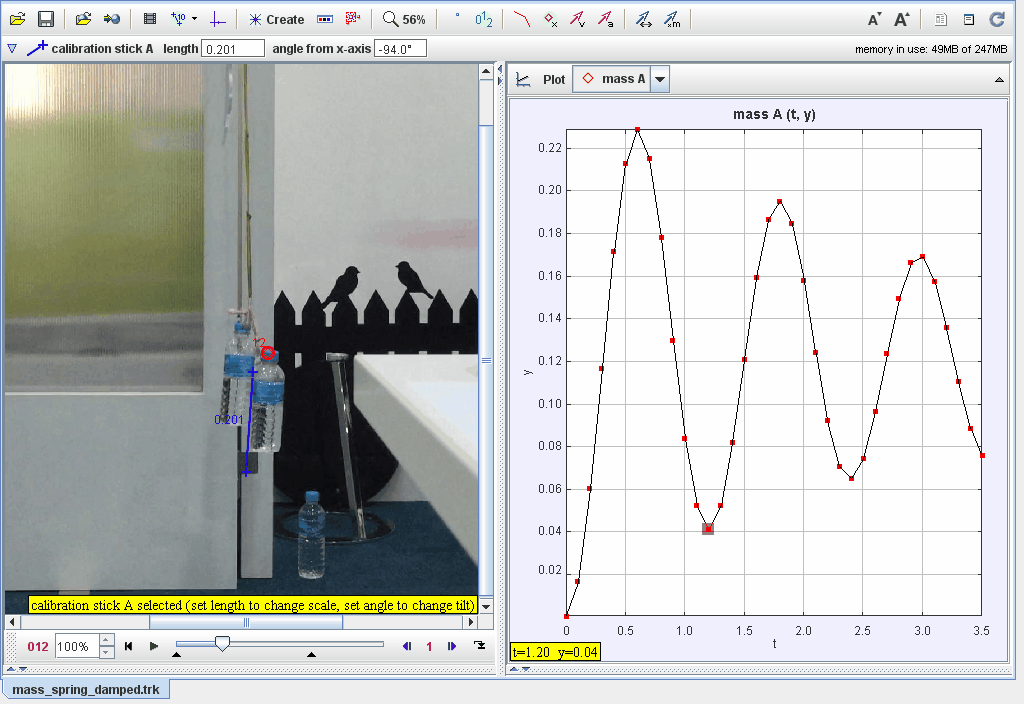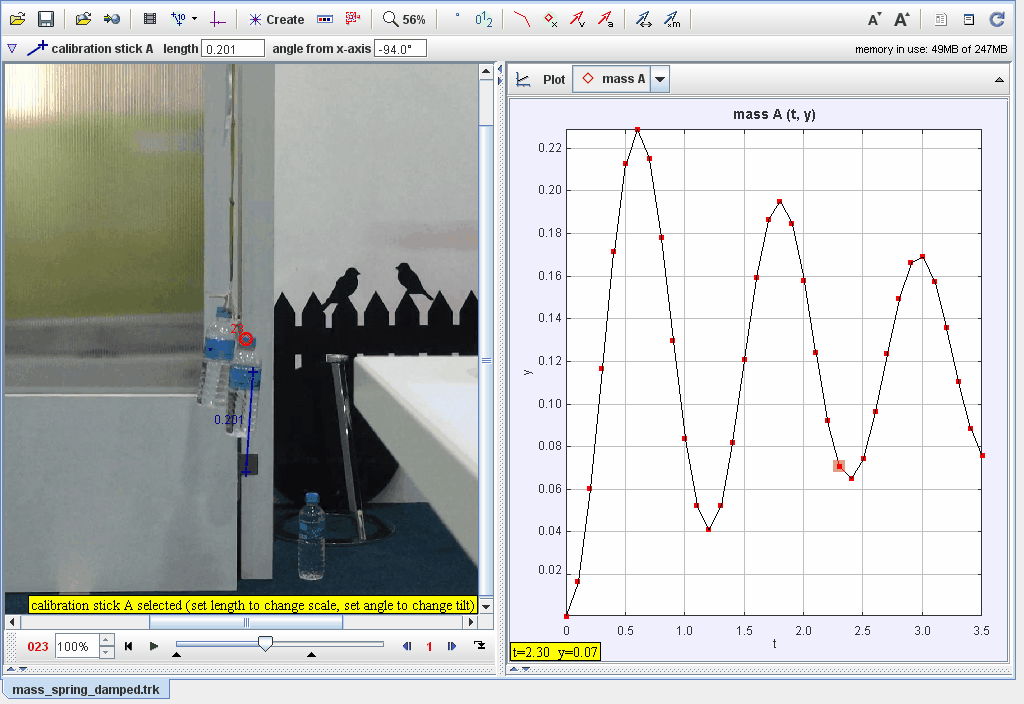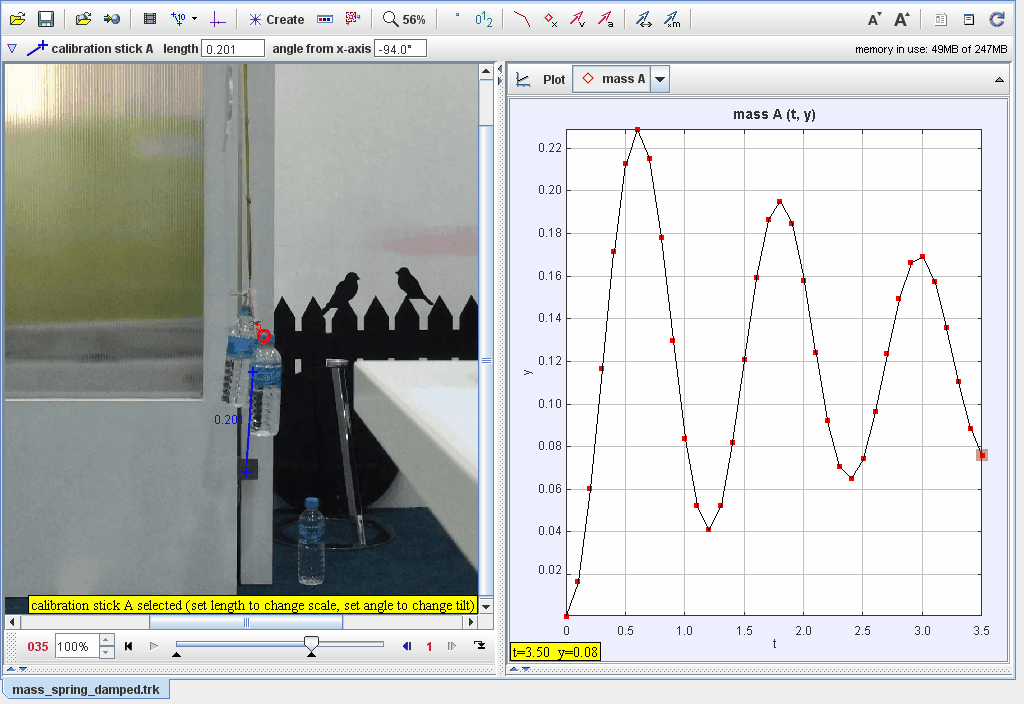
Read more: Tracker 2 Bottles Dampened Oscillation Up and Down
- Details
- Written by leongster
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 2988
- Details
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 3112
- Details
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 2885
- Details
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 2794
- Details
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 2838
- Details
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 2658
- Details
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 2342
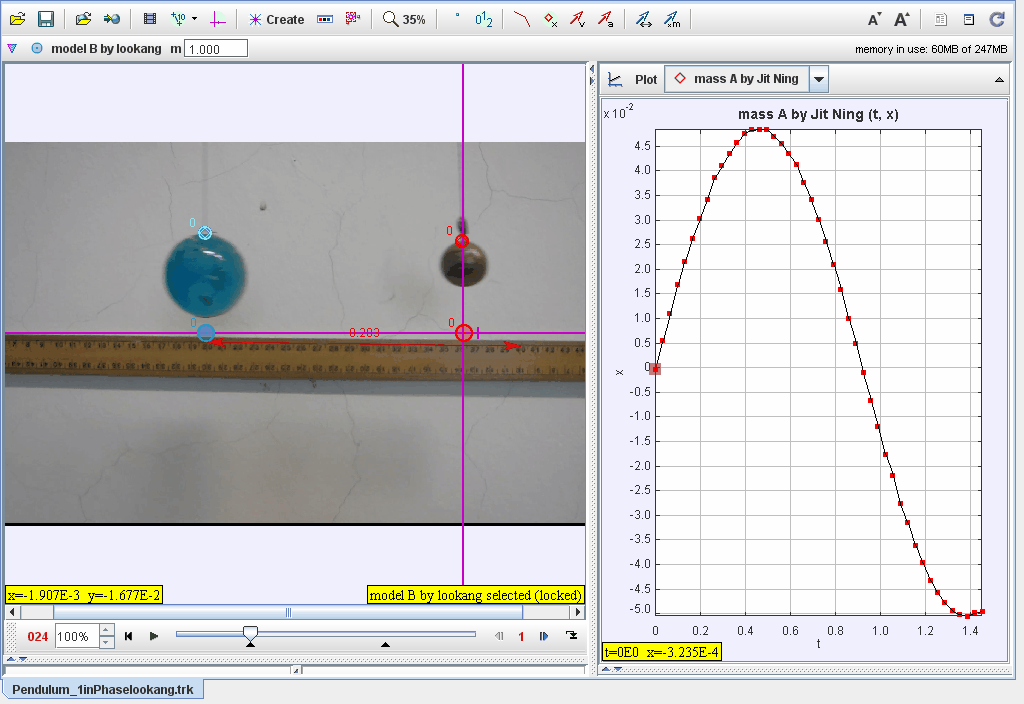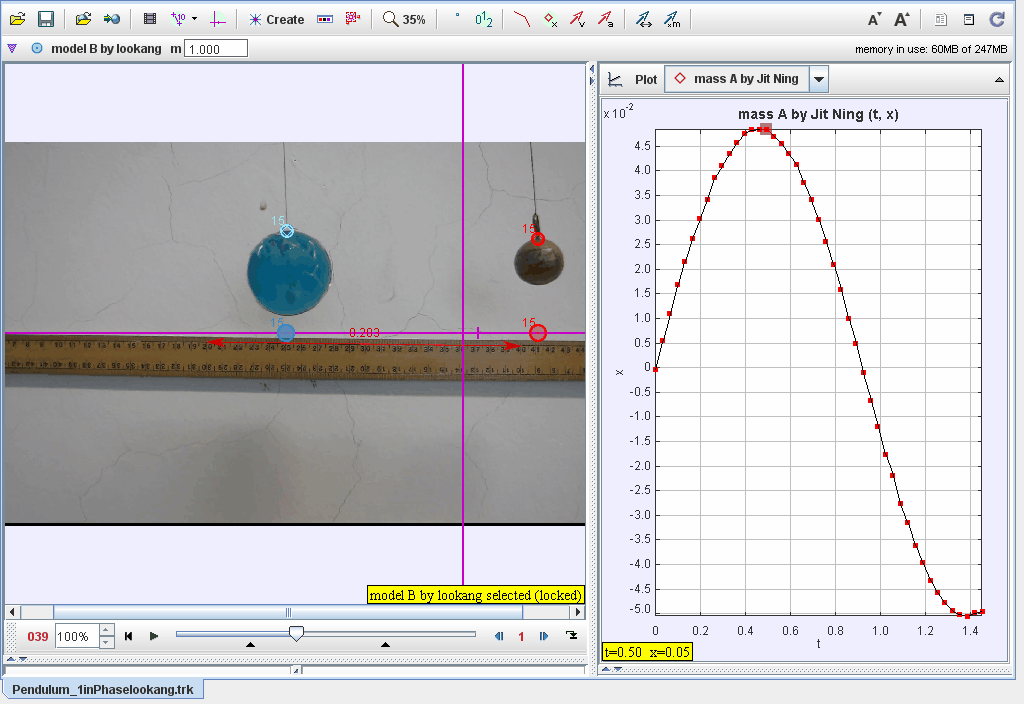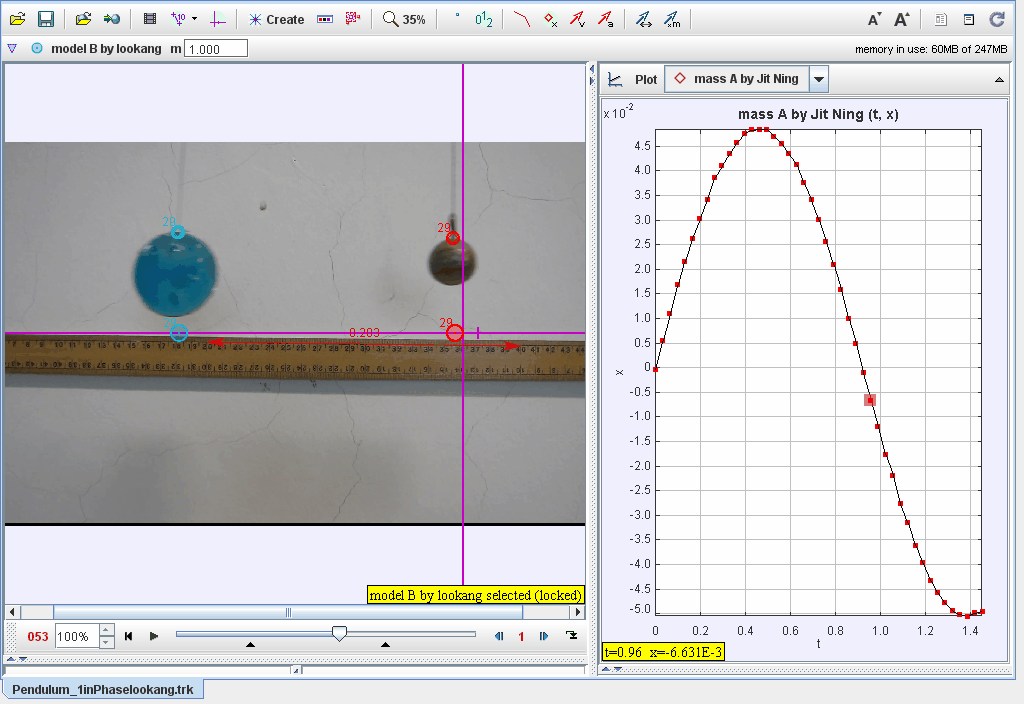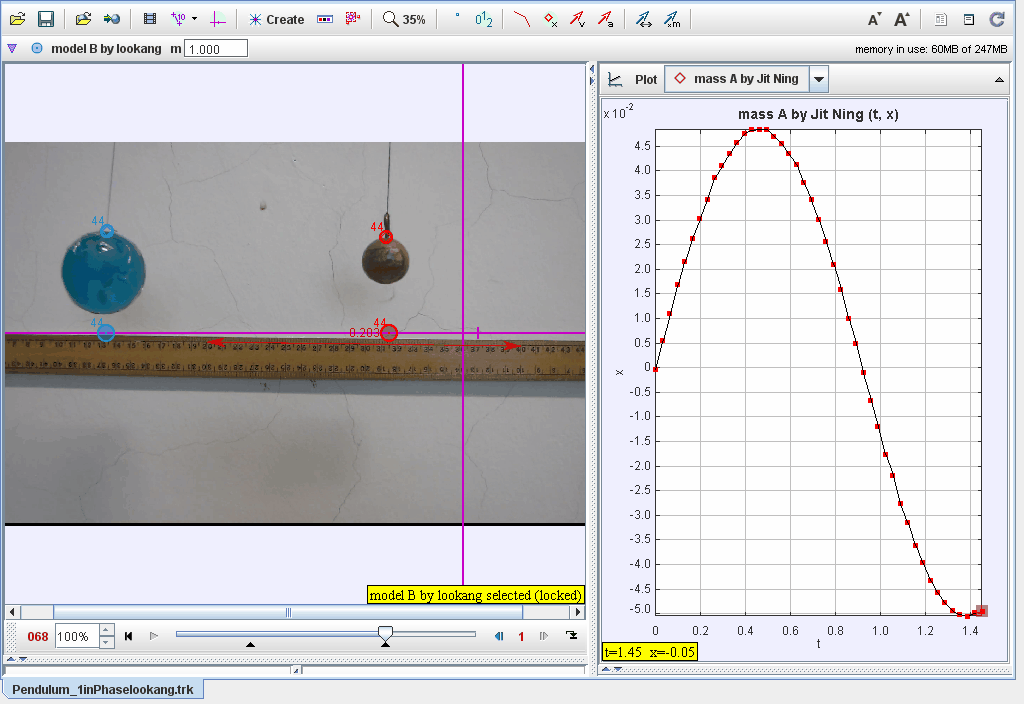
Read more: Tracker Modeling in 2 Pendulum swinging in anti-phase
- Details
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 2691
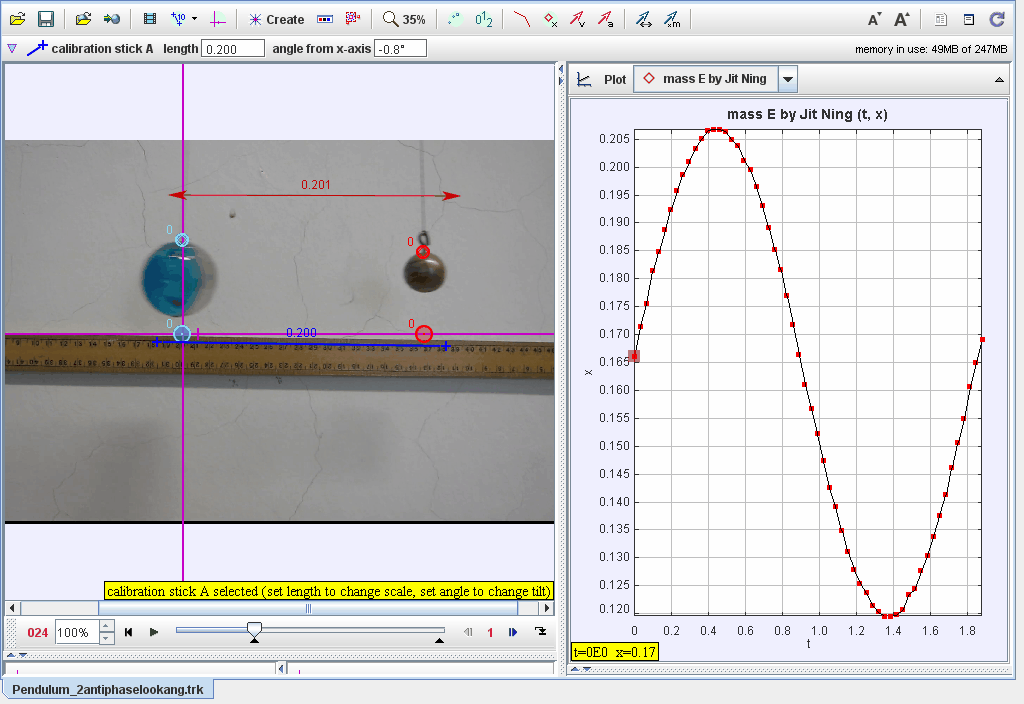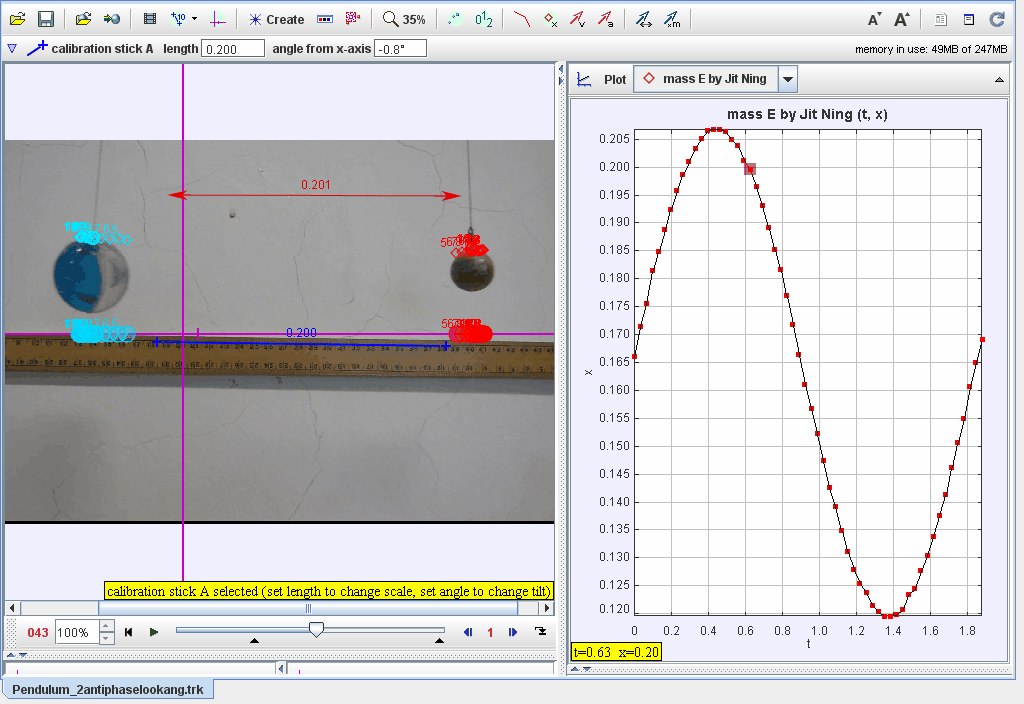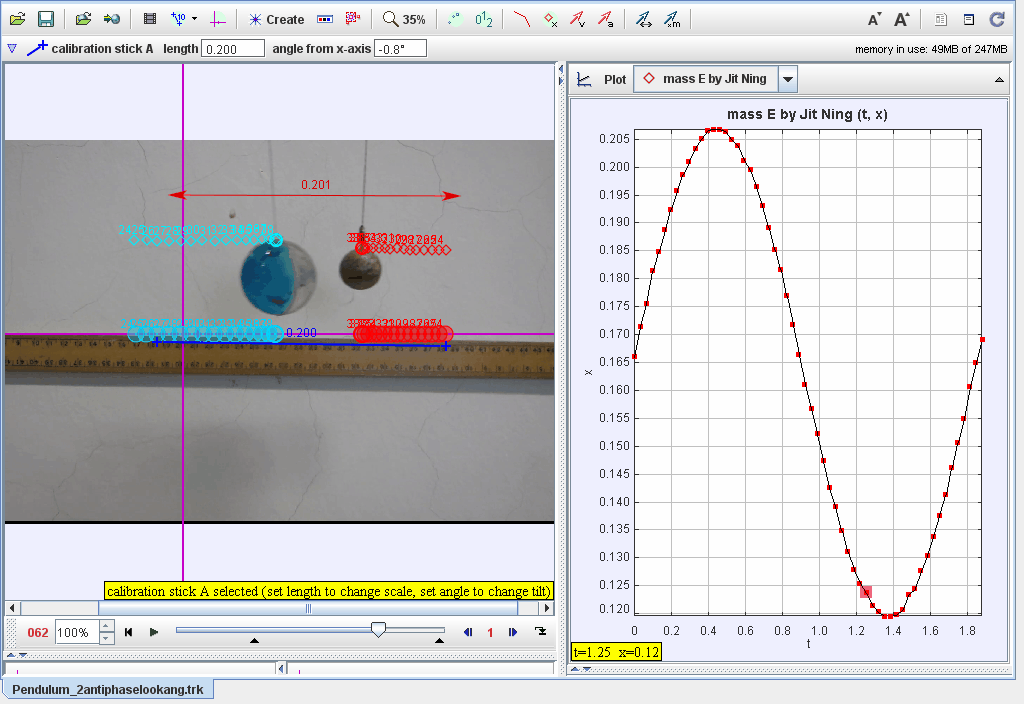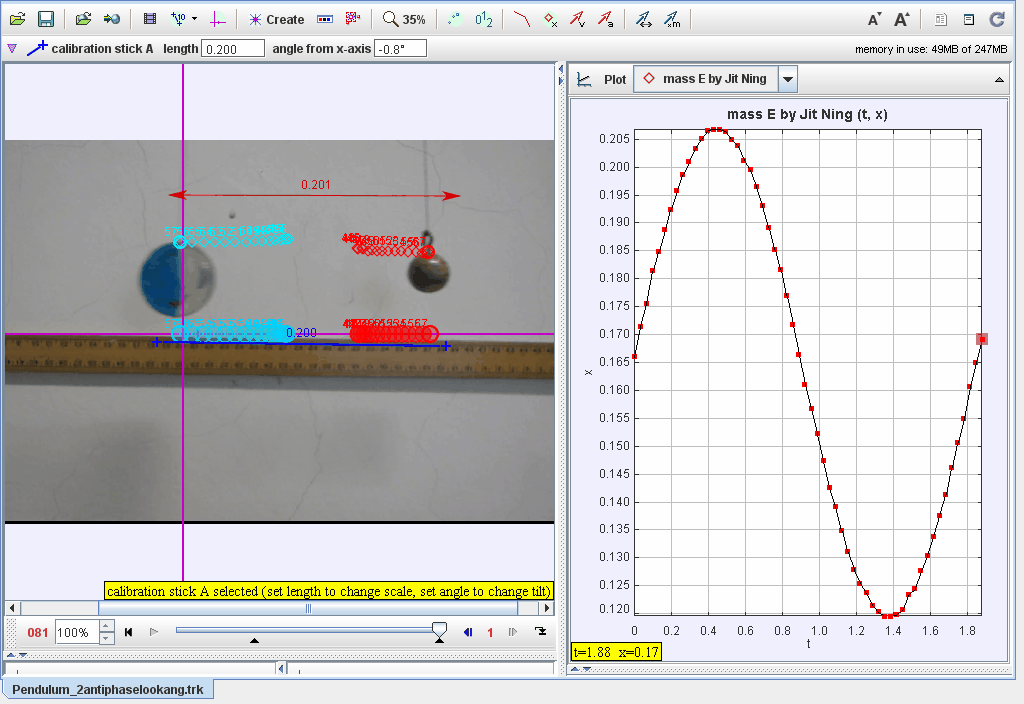
Read more: Tracker Modeling in 2 Pendulum swinging in out of phase
- Details
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 2781
- Details
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 2319
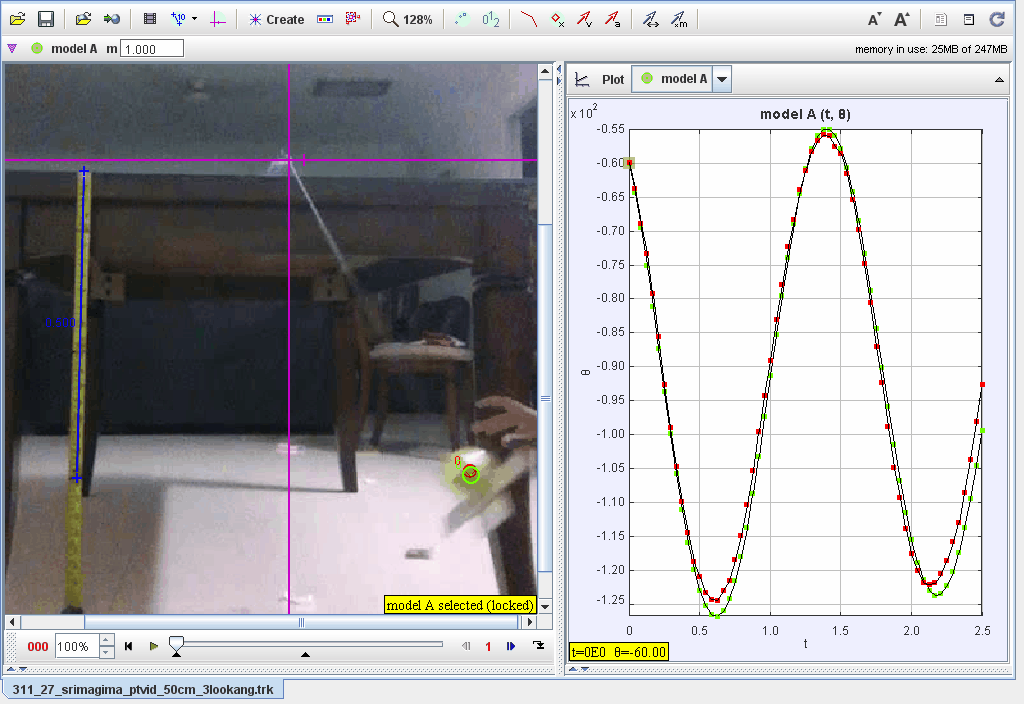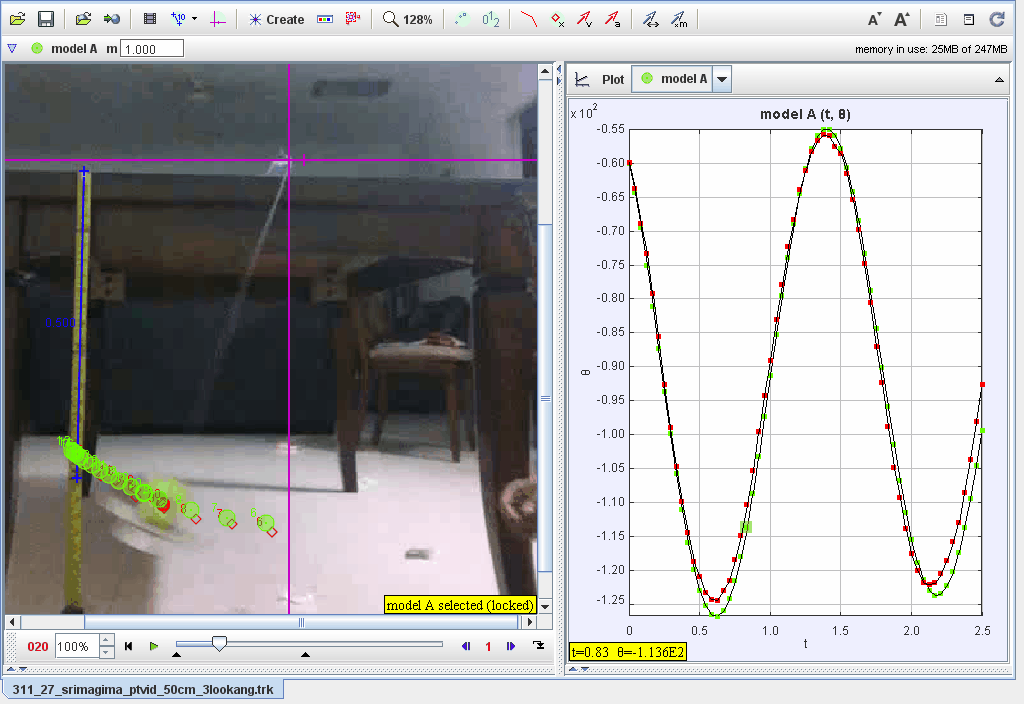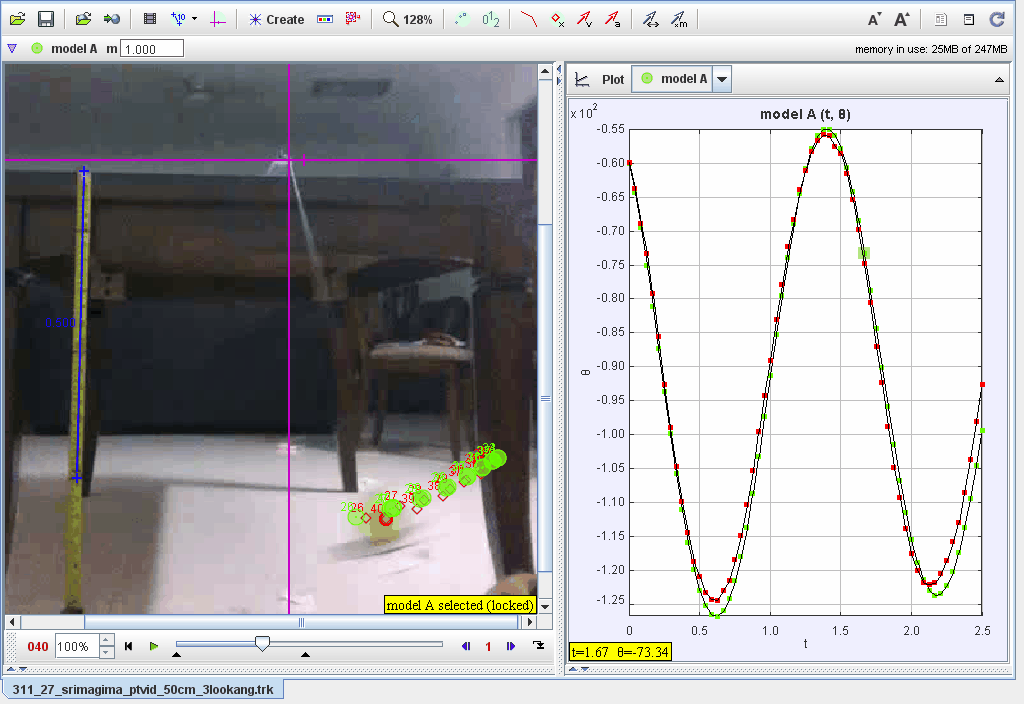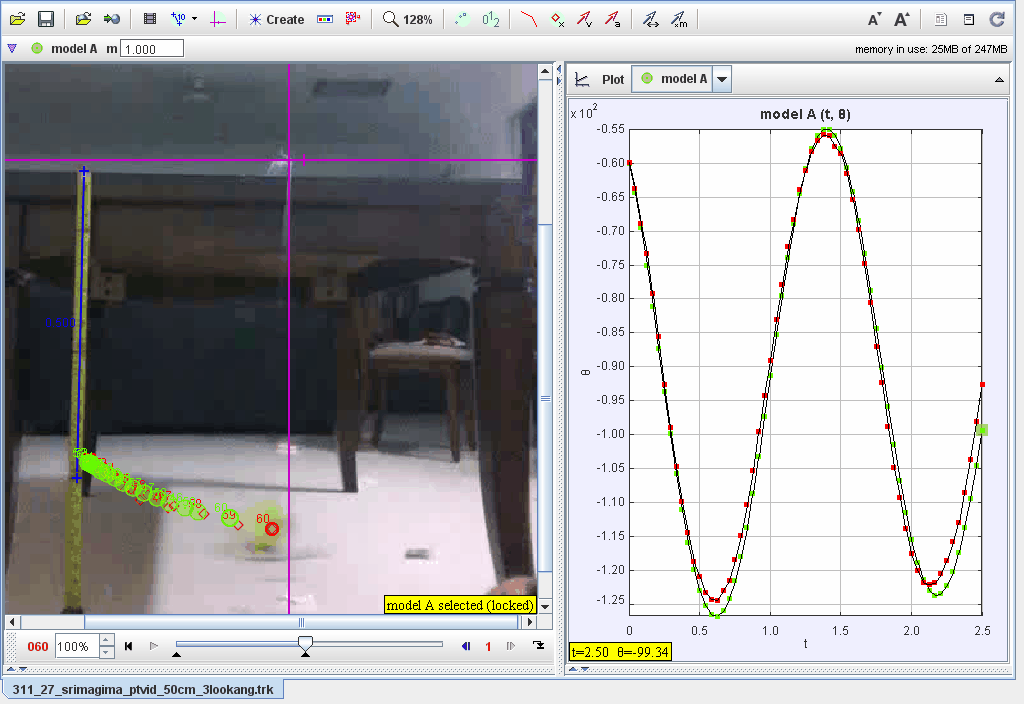
Read more: pendulum model (311_27_srimagima_ptvid_50cm_3lookang)
- Details
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 2387
- Details
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 2395
- Details
- Parent Category: 02 Newtonian Mechanics
- Category: 09 Oscillations
- Hits: 3022
- Details
- Written by Loo Kang Wee
- Parent Category: 02 Newtonian Mechanics
- Category: 10 Rotational Motion
- Hits: 3422
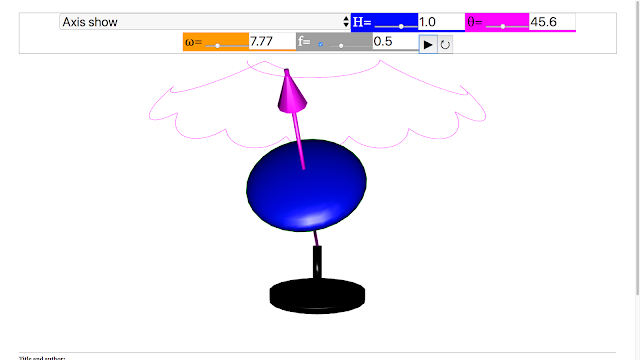
Read more: Spinning Gyroscope JavaScript HTML5 Applet Simulation Model
- Details
- Written by Fremont
- Parent Category: 02 Newtonian Mechanics
- Category: 10 Rotational Motion
- Hits: 3275
Read more: Rotation of Solid Sphere and Thin Shell JavaScript Simulation Applet HTML5
- Details
- Written by Fremont
- Parent Category: 02 Newtonian Mechanics
- Category: 10 Rotational Motion
- Hits: 4803
Read more: Multiple Objects Rolling Down Inclined Plane JavaScript Simulation Applet HTML5
- Details
- Written by Fremont
- Parent Category: 02 Newtonian Mechanics
- Category: 10 Rotational Motion
- Hits: 2803
Read more: Accelerated Platform with Cylinder JavaScript Simulation Applet HTML5
- Details
- Written by Wei Chiong
- Parent Category: 02 Newtonian Mechanics
- Category: 10 Rotational Motion
- Hits: 6147
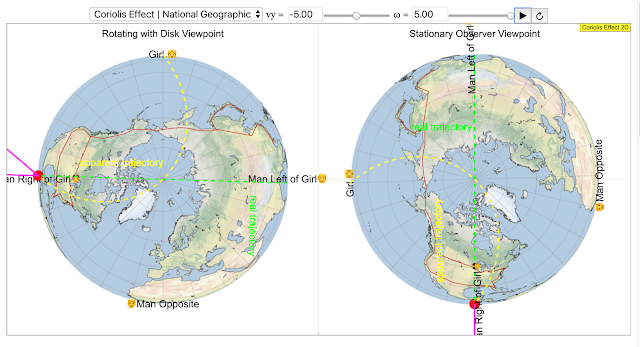
Read more: Coriolis Effect 2D JavaScript Simulation Applet HTML5
- Details
- Written by Wei Chiong
- Parent Category: 02 Newtonian Mechanics
- Category: 10 Rotational Motion
- Hits: 4371
Read more: Coriolis Effect 3D JavaScript Simulation Applet HTML5